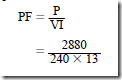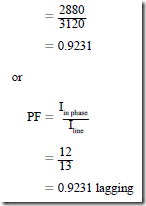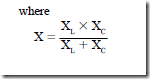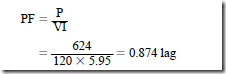PARALLEL CIRCUIT WITH BRANCHES CONTAINING R, XL, AND XC
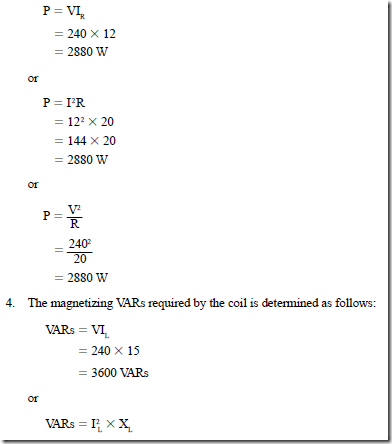
Figure 8–10 illustrates a parallel circuit consisting of three branch circuits. One branch contains resistance, a second branch contains pure inductance, and the third branch contains capacitance.
PROBLEM 5
Statement of the Problem
For the circuit shown in Figure 8–10, determine the following items:
1. The current taken by each branch
2. The line current
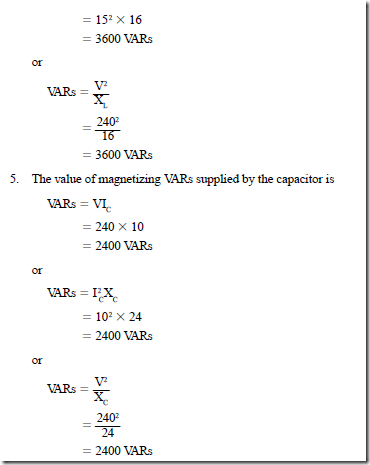
3. The power
4. The magnetizing VARs required by the coil
5. The magnetizing VARs supplied by the capacitor
6. The net magnetizing VARs supplied by the line
7. The power factor and the phase angle for the circuit
8. Total impedance of the circuit
Construct a vector diagram for the parallel circuit.
Solution
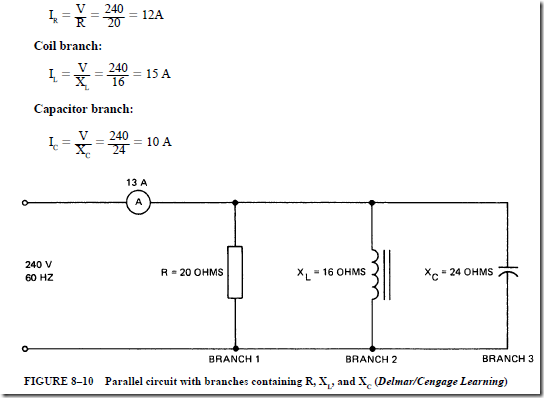
1. The current taken by each branch of the circuit is as follows:
Resistance branch:
2. The current in the capacitor branch leads the line voltage by 90°. The coil current lags the same line voltage by 90°. In other words, IC and IL are 180° out of phase with each other. I – IC (15 A – 10 A) yields a net quadrature current of 5 A inductive. This means that the line current consists of an in-phase component of 12 A and a lagging quadrature component of 5 A. The line current is
3. All of the power taken by this circuit is used in the resistance branch. The currents taken by the coil branch and the capacitor branch are 90° out of phase with the line voltage. As a result, the power taken by either of these branches is zero. The power for the resistance branch is the power for the entire parallel circuit. This value, in watts, can be determined by any one of the following expressions:
6. The net magnetizing VARs supplied by the line is the difference between the VARs required by the coil and the VARs supplied by the capacitor:
Net VARs = 3600 – 2400 = 1200 VARs
7. The power factor for this parallel circuit may be found using either of two ratios: (a) the ratio of the power in watts to the input volt-amperes, or (b) the ratio of the in-phase current to the total line current. The resulting power factor is lagging. This lag is due to the fact that the quadrature current taken by the coil branch is greater than the current taken by the capacitor branch. The net quadrature current supplied by the ac source lags the line voltage by 90°. The power factor is
8. The angle fJ for a lagging power factor of 0.9231 is 22.6°. The vector diagram for the parallel circuit with branches containing R, X , and X is shown in Figure 8–11.
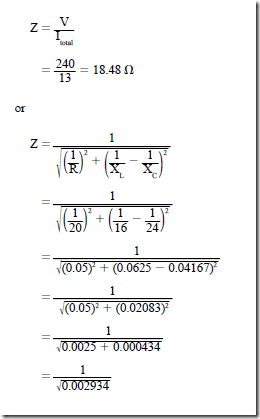
9. The total impedance of the circuit can be found using either of the following formulas:
In this formula, X is a positive number and X is a negative number. Therefore, Z will be either positive or negative depending on whether the circuit is more inductive (positive) or capacitive (negative).
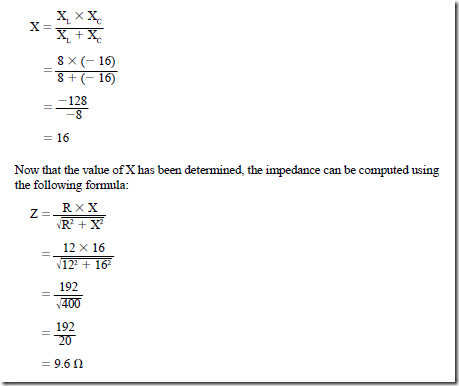
Assume a parallel circuit contains a 12-n resistor connected in parallel with an inductor that has an inductive reactance of 8 n and a capacitor that has a capacitive reactance of 16 n. To find the total impedance of this sample circuit using this formula, first determine the value of X:
PARALLEL CIRCUIT RESONANCE
The current in a series circuit reaches its maximum value at resonance. At this point, the effects of inductive reactance and capacitive reactance cancel each other. Also, the full line voltage is impressed across the resistance of the circuit. Thus, for the series circuit, the current and line voltage are in phase.
An analysis of a series and a parallel resonant circuit having identical values of R, L, and C will show the following similarities:
1. The line currents and the line voltages are in phase.
2. The power factors are unity.
3. The values of X and X are equal.
4. The impedances are purely resistive.
These similarities may give the impression that both circuits are identical at resonance. However, there are two important differences between series and parallel circuits, which can be seen by looking at the magnitudes of the currents and impedances:
1. The current is at a maximum in a series resonant circuit and at a minimum in a parallel resonant circuit.
2. The impedance is at a minimum in a series resonant circuit and at a maximum in a parallel resonant circuit.
These factors make parallel resonance a useful feature in tuned circuits.
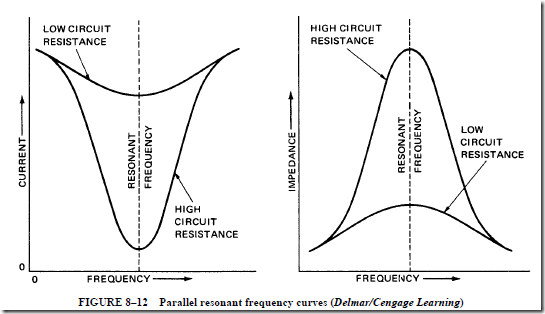
Current and Impedance Discussion
Figure 8–12 shows the current and impedance curves for a parallel resonant circuit. These curves are opposite to those shown for a series resonant circuit. The current equation for the parallel circuit is
where IXC is negligible at low frequencies. The capacitive reactance has high values at frequencies below resonance. This means that only very small currents flow. The opposite is true for the inductive branch, where I becomes a maximum value.
For frequencies at resonance, the current equation takes the following form:
At resonance, x and X are equal and 180° out of phase. Their currents are also equal and 180° out of phase and thus cancel. The current remaining is the resistive component, I . (Iis at a minimum.) At resonance, it is possible in theory to have the following valR L ues: an inductive current of 10 A, a capacitive current of 10 A, and a line current of 0 A.
At frequencies above resonance, the current equation takes the following form:
By examining what happens at frequencies below resonance, the student should be able to analyze the previous resonance case where I becomes a maximum value again.
The impedance curve is the reciprocal of the current curve. The impedance curve is defined by the equation Z = V/I.
The parallel circuit shown in Figure 8–10 has a lagging power factor of 0.9231. A larger capacitor may be used in the capacitive branch of the circuit. This increased value causes an increase in the leading current. For example, for a capacitor with X = 16 n, the quadrature current for this capacitor is 15 A leading. The coil in branch 2 of the circuit also requires 15 A. The 15 A taken by the capacitor leads the line voltage by 90°. The 15 A supplied to the coil lags the line voltage by 90°.
Resonant Parallel Circuit
Figure 8–13 shows a parallel circuit in which the coil and capacitor branches each take 15 A. This circuit is a resonant parallel circuit. The 15 A in the coil branch and the 15 A in the capacitor branch are 180° out of phase. As a result, the currents cancel each other. The source supplies the in-phase current only for the resistance load in the circuit. Therefore, the line current is the same as the current taken by the resistor.
PROBLEM 6
Statement of the Problem
For the circuit given in Figure 8–13, determine the following items:
1. The line current
2. The power
3. The power factor and the phase angle
Construct a vector diagram for this parallel resonant circuit.
PARALLEL CIRCUIT WITH BRANCHES CONTAINING R, Zcoil, AND XC
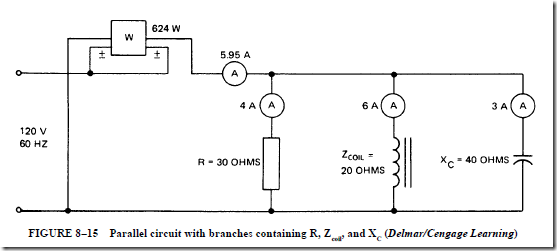
The circuit shown in Figure 8–15 contains three branches: a wattmeter, which indicates the true power in the circuit; and ammeters, which indicate the line current and the current in each branch.
One branch contains a pure resistance of 30 n. A second branch contains a load that has both resistance and inductance. This is similar to the load shown in Figure 8–8.
As before, the value of Zcoil is not to be confused with the value of Z total coil is used to represent the value of impedance for the coil only, and Z total is used to represent the value of impedance for the entire circuit. Finally, the third branch contains a capacitor with a capacitive reactance of 40 n.
Power Factor for the Circuit
The power factor of the circuit is found by the use of the following expression:
The entire circuit has a lagging power factor because the inductive reactive current is more than the current in XC. The phase angle is 29° lagging.
Power Factor for the Impedance Coil
To find the power factor of the impedance branch, the power loss in the coil must be determined first:
The current in the impedance coil lags the line voltage. The cosine of this angle of lag is 0.20. Thus, the angle itself is 78.5°.
The Vector Diagram. The angles and current values calculated for this problem are used to obtain the vector diagram shown in Figure 8–16. A graphical solution to the parallel circuit is shown in the following procedure. Steps 1 through 3 require a compass, protractor, and ruler.
1. Lay out the current vectors as shown:
This problem can be solved mathematically by resolving the I coil vector (6 A) into a horizontal (in-phase) component and a vertical (quadrature) component. This method is used in the following section on power factor correction.