CORRECTING MOTOR POWER FACTOR
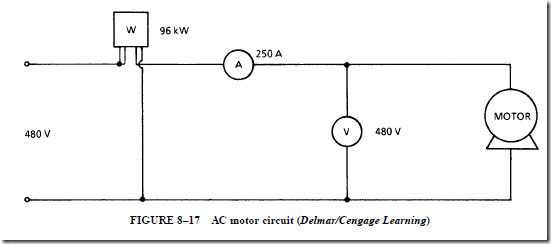
It is sometimes necessary to correct the power factor of a motor. The following procedure can be used to perform this task. Before the power factor of a motor can be corrected, it must first be determined how much out of phase current and voltage are with each other. In the circuit shown in Figure 8–17, a wattmeter, an ammeter, and a voltmeter are used to measure circuit values. The voltmeter indicates a voltage of 480 V connected to the motor. The ammeter shows a total current draw of 250 A, and the wattmeter shows the true power of the circuit to be 96 kW.
Computing Apparent Power
The apparent power or volt-amperes of the circuit can be determined by multiplying the applied voltage by the total current:
Computing Power Factor
Because both the true power and the apparent power are known, the power factor of the circuit can be found using the following formula:
 Calculating the Reactive Power
Calculating the Reactive Power
The amount of reactive power or VARs (volt-amperes-reactive) can be determined using the formula
The 72 kVARs represents the amount of reactive power in the circuit. Because this reactive component is caused by a motor, it is inductive. If the power factor is to be corrected, the same amount of capacitive VARs must be added to the circuit. The capacitive VARs will cancel the inductive VARs, and the current and voltage will be in phase with each other.
Calculating the Capacitance Needed
To calculate the amount of capacitance needed, first determine the amount of current that must flow to produce 72 capacitive kVARs. This can be found by using the following formula:
The amount of capacitive reactance needed to produce a flow of 150 A through a capacitor can now be computed using the following formula:
The amount of capacitance needed to produce a capacitive reactance of 3.2 n at 60 Hz can now be computed using the formula
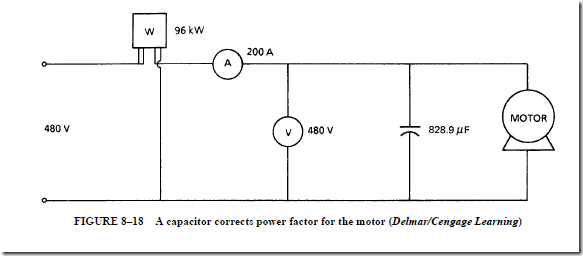
where C = 0.008289 F or 828.9 ,uF If a capacitance of 828.9 ,uF is connected across the motor as shown in Figure 8–18, the power factor will be corrected to unity or 100%.
FIGURE 8–18 A capacitor corrects power factor for the motor (Delmar/Cengage Learning)
Advantages of Unity Power Factor. Utility companies and industrial plants try to keep the power factor of their ac circuits as close to unity as possible. The unity power factor is desirable because of the following:
1. The quadrature current in the line wires causes I2 R losses, just as the in-phase current causes power losses in the circuit conductors. Therefore, if the power factor is raised to a value near unity, the power losses in the line wires are reduced and the efficiency of transmission is increased.
2. If the power factor is corrected to a value near unity, there is less voltage drop in the line. As a result, the voltage at the load is more constant.
3. AC circuits operated at a high power factor improve the efficiency and operating performance of ac generators and transformers supplying these circuits.
SUMMARY
• The voltage across any branch circuit in a parallel arrangement, neglecting line voltage drops, is the same as the line voltage.
• In the analysis of series circuits, the current is used as the reference vector.
• In the analysis of parallel circuits, the voltage is used as the reference vector. Thus, all angles in the analysis of parallel circuits are measured with respect to the voltage vector.
• For an ac parallel circuit with branches containing only pure resistance, the calculations are the same as those for a direct-current parallel circuit:
For an ac parallel circuit, the current in each branch circuit containing a pure resistive component is in phase with the line voltage. Thus, the power factor is
• In a parallel ac circuit containing R, X , and X components, the total line current may be out of phase with the line voltage. In this case, the line current must be calculated using vectors:
A parallel circuit with branches containing R, X , and X has the following properties as determined by calculation:
The current in the inductive branch lags the same line voltage by 90°.
and I are 180° out of phase with each other, and (IL – IC) yields a net quadrature current. The line current consists of an in-phase component and a leading or lagging quadrature component:
2. Power. All of the power taken by this circuit is used in the resistive branch.
Because the currents taken by the coil branch and the capacitor branch are 90° out of phase with the line voltage, no power is taken in either of these branches. The power for the resistance branch is the power for the entire parallel circuit:
3. Power factor (PF). The power factor for an ac parallel circuit is determined using either of the following ratios:
The net magnetizing VARs supplied by the line is the difference between the VARs required by the coil and the VARs supplied by the capacitor.
• Resonance in ac circuits:
1. Series and parallel resonant circuits having identical values of R, L, and C have the following similarities:
a. The line currents and the line voltages are in phase.
b. The power factors are unity.
c. The values of X and X are equal.
d.The impedances are purely resistive.
2. Series and parallel resonant circuits having the same values of R, L, and C are different in the following respects:
a. The current is at a maximum in a series resonant circuit and at a minimum in a parallel resonant circuit.
b. The impedance is at a minimum in a series resonant circuit and at a maximum in a parallel resonant circuit.
These factors make parallel resonance a useful feature in tuned circuits.
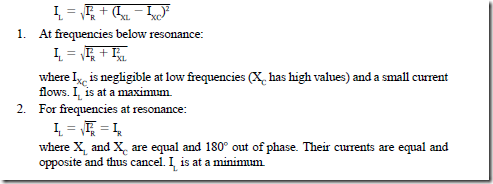
• The current and impedance curves for a parallel resonant circuit are opposite to those for a series resonant circuit. The current equation for the parallel resonant circuit is
3. At frequencies above resonance:
where IXL is negligible and XL has high values. IL is at a maximum again.
4. The impedance curve is the reciprocal of the current curve:
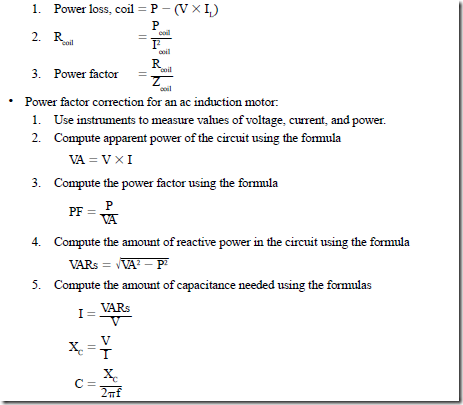
• For a parallel ac circuit containing impedance, the power lost in the coil, the resistance of the coil, and the power factor of the coil are determined as follows:
• Advantages of a unity power factor:
1. The quadrature current causes I2 R losses. Correcting the power factor to a value near unity reduces the power losses in the line wires and the efficiency of trans- mission is increased.
2. Correcting the power factor to a value near unity decreases the voltage drop.
As a result, the voltage at the load is more constant.
3. If ac circuits are operated at a high power factor, there is an improvement in the efficiency and operating performance of ac generators and transformers supplying these circuits.
Achievement Review
1. A parallel circuit consists of two branches connected to a 120-V, 60-Hz source.
The first branch has a noninductive resistance load of 50 n. The second branch consists of a coil with an inductance of 0.2 H and negligible resistance.
a. Find the current in each branch.
b. Determine the line current.
c. Determine the power factor of the parallel circuit.
d. Draw a labeled vector diagram for this circuit.
2. A resistance of 40 n is connected in parallel with a pure inductance of 0.24 H across a 120-V, 25-Hz supply.
a. Find the current taken by each branch.
b. Determine the line current.
c. Determine the power factor of the parallel circuit.
d. Determine the combined impedance of the parallel circuit.
e. Draw a labeled vector diagram for the parallel circuit.
3. A 120-V, 60-Hz source supplies a parallel circuit consisting of two branches.
Branch 1 is a noninductive resistance load of 5 n. Branch 2 feeds a 1000- ,uF capacitor with negligible resistance.
a. Determine
(1) the current taken by each branch.
(2) the line current.
(3) the combined impedance of the parallel circuit.
(4) the power factor and phase angle for the parallel circuit.
b. Draw a labeled vector diagram for the parallel circuit.
4. A 240-V, 60-Hz single-phase source supplies a parallel circuit consisting of two branches. Branch 1 has a resistance of 20 n in series with an inductance of
0.04 H. Branch 2 feeds a 50-,uF capacitor with negligible resistance.
a. Determine
(1) the current in each branch.
(2) the total current.
(3) the power factor of branch 1 (containing R and X components in series).
(4) the power factor of the entire parallel circuit.
b. Draw a labeled vector diagram for the parallel circuit.
5. A 240-V, 60-Hz parallel circuit supplies three branches. Branch 1 consists of a noninductive heating load with a resistance of 12 n, branch 2 feeds a pure inductive reactance load of 8 n, and branch 3 is a capacitor having a capacitive reactance of 16 n.
a. Determine
(1) the current taken by each of the three branches.
(2) the total current.
(3) the combined impedance of the parallel circuit.
(4) the circuit power factor.
(5) the total power in kilowatts taken by the parallel circuit.
b. Draw a labeled vector diagram for this circuit.
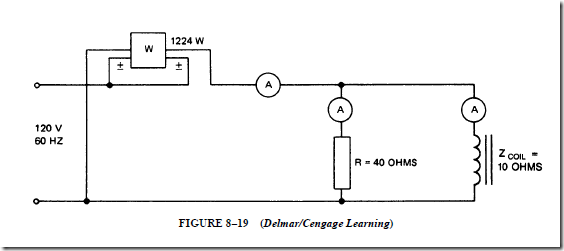
6. Using the circuit shown in Figure 8–19, determine
a. the current taken by each branch.
b. the power expended in the resistance branch, in watts.
c. the power expended in the coil branch, in watts.
d. the impedance of the coil.
e. the effective resistance and the inductive reactance components of the coil.
7. a. Using the circuit in the preceding question, determine
(1) the total current.
(2) the power factor and the phase angle for the inductive branch circuit.
(3) the power factor and the phase angle for the entire parallel circuit.
(4) the combined impedance of the parallel circuit.
b. Draw a vector diagram and label it properly for this circuit.
8. A 125-V, 60-Hz parallel circuit has three branches. Branch 1 has a resistance of 20 n, branch 2 feeds a coil with an inductance of 0.0211 H, and branch 3 supplies a 200-,uF capacitor. The resistances of both the coil and the capacitor are negligible.
Determine
a. the current in each branch.
b. the total current.
c. the power factor for the parallel circuit.
9. Explain the meaning of the term parallel resonance.
10. An ac parallel circuit consists of three branches connected to a 120-V, 60-Hz supply. Branch 1 consists of a noninductive resistance load, branch 2 feeds a coil, and branch 3 supplies a capacitor. The resistances of the coil and the capacitor are negligible. The total line current is 10 A. The power factor of the entire parallel circuit is 90% lagging. The capacitor has a capacitive reactance of 12.5 n.
a. Draw a labeled vector diagram of the circuit.
b. Determine
(1) the current taken by each of the three branches.
(2) the true power in watts taken by the entire parallel circuit.
11. The capacitor in the circuit described in question 5 is changed to one having the proper rating to give a circuit power factor of unity. Determine the following data for the capacitor value that causes the circuit to be in parallel resonance:
a. Rating in VARs
b. Capacitive reactance
c. Rating in microfarads
12. In the circuit given in question 6, there is to be a third branch feeding a capacitor.
This capacitor has the proper rating to correct the power factor to unity. Using the information provided in question 6, determine
a. the rating of the capacitor, in VARs.
b. the capacitive reactance of the capacitor (assume the resistance to be negligible).
c. the rating of the capacitor, in microfarads. Construct a vector diagram for this circuit.
13. A noninductive heater and an ac motor are operated in parallel across a 120-V, 25-Hz source. The heater takes 600 W. The motor takes 360 W at a lagging power factor of 60%. Determine
a. the current taken by each of the two branches.
b. the total current.
c. the circuit power factor.
14. A 120-V induction motor requires 24 A at a lagging power factor of 75%. If a lamp load of 30 A is connected in parallel with the motor, what are the power fac- tor and the phase angle for the entire parallel circuit?
15. Why is it important to maintain a high power factor with alternating-current systems?
16. At full load, a 220-V, 2-horsepower (hp), single-phase induction motor takes 12 A when operated on a 60-Hz service. The full-load power factor is 80% lagging.
At full load, determine
a. the phase current.
b. the power, in watts.
c. the quadrature current.
d. the magnetizing VARs required.
e. the input volt-amperes.
17. The power factor of the circuit in question 16 is to be corrected to a value of unity by connecting a capacitor in parallel with the motor. Determine
a. the rating of the capacitor, in VARs.
b. the capacitive reactance of the capacitor.
c. the rating of the capacitor, in microfarads.
d. the line current after the power factor is increased to 100% or unity.
18. An industrial plant has a load of 50 kW at a power factor of 70% lag, feeding from a 240-V, 60-Hz system. Determine
a. the line current.
b. the capacitor rating, in kilovars, required to raise the power factor to unity.
c. the line current after the capacitor is added to the circuit.
PRACTICE PROBLEMS FOR UNIT 8
Resistive Inductive Parallel Circuits
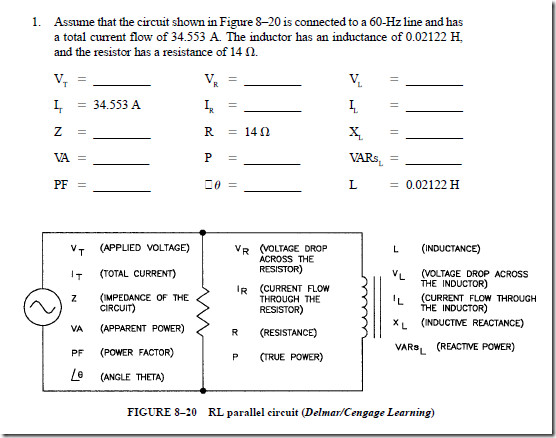
Find the missing values for the following circuits. Refer to Figure 8–20 and the formulas under the Resistive Inductive (Parallel) section of Appendix 15.
Resistive Capacitive Parallel Circuits
Find the missing values for the following circuits. Refer to Figure 8–21 and the formulas listed under the Resistive Capacitive (Parallel) section of Appendix 15.
5. Assume that the circuit shown in Figure 8–21 is connected to a 60-Hz line and has a total current flow of 10.463 A. The capacitor has a capacitance of 132.626 ,uF, and the resistor has a resistance of 14 n.
7. Assume that the circuit in Figure 8–21 is connected to a 600-Hz line and has a current flow through the resistor of 65.6 A and a current flow through the capacitor of 124.8 A. The total impedance of the circuit is 2.17888 n.
8. Assume the circuit is connected to a 1000-Hz line and has a true power of 486.75 W and a reactive power of 187.5 VARs. The total current flow in the circuit is
Resistive-Inductive-Capacitive Parallel Circuits
Find the missing values in the following circuits. Refer to Figure 8–22 and the formulas under the Resistive-Inductive-Capacitive (Parallel) section of Appendix 15.
9. The circuit shown in Figure 8–22 is connected to a 120-volt, 60-Hz line. The resistor has a resistance of 36 n, the inductor has an inductive reactance of 40 n and the capacitor has a capacitive reactance of 50 n.
10. The circuit in Figure 8–22 is connected to a 400-Hz line with a total cur- rent flow of 22.627 A. There is a true power of 3840 W. The inductor has a reactive power of 1920 VARs, and the capacitor has a reactive power of 5760 VARs.
11. The circuit in Figure 8–22 is connected to a 60-Hz line. The apparent power in the circuit is 48.106 VA. The resistor has a resistance of 12 n. The inductor has an inductive reactance of 60 n, and the capacitor has a capacitive reactance of 45 n.
12. The circuit shown in Figure 8–22 is connected to a 1000-Hz line. The resistor has a current flow of 60 A, the inductor has a current flow of 150 A, and the capacitor has a current flow of 70 A. The circuit has a total impedance of 4.8 n.





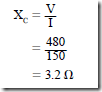
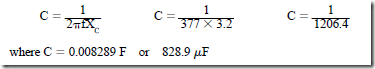

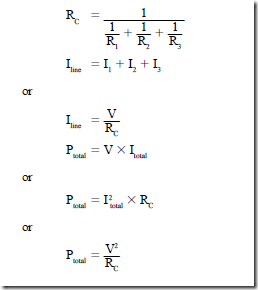
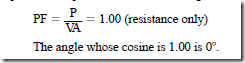
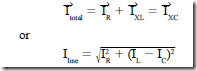


![image_thumb[1] image_thumb[1]](http://lh6.ggpht.com/-WrZtDJNhIJ4/VLF9OJ10QHI/AAAAAAABDi4/lBYLUBZCb9U/image_thumb%25255B1%25255D_thumb.png?imgmax=800)
![image_thumb[2] image_thumb[2]](http://lh4.ggpht.com/-yo2Z-O8MKzM/VLF9VSGB8iI/AAAAAAABDjI/ehpRJkpRfc8/image_thumb%25255B2%25255D_thumb.png?imgmax=800)
![image_thumb[3] image_thumb[3]](http://lh6.ggpht.com/-MHIZoi9CHwU/VLF9d6g1rkI/AAAAAAABDjY/_EO5zNbhgsQ/image_thumb%25255B3%25255D_thumb.png?imgmax=800)
![image_thumb[4] image_thumb[4]](http://lh4.ggpht.com/-zbDMt58hTxQ/VLF9mH1qWfI/AAAAAAABDjo/_1czdokFEJc/image_thumb%25255B4%25255D_thumb.png?imgmax=800)
![image_thumb[5] image_thumb[5]](http://lh5.ggpht.com/-fjWpmSeoz20/VLF__KuTmeI/AAAAAAABDkE/scO2Nv-L6qk/image_thumb%25255B5%25255D_thumb.png?imgmax=800)
![image_thumb[6] image_thumb[6]](http://lh3.ggpht.com/-AW2jxIJokUI/VLGALaNUILI/AAAAAAABDkU/VHohPSgFYxY/image_thumb%25255B6%25255D_thumb.png?imgmax=800)
![image_thumb[7] image_thumb[7]](http://lh3.ggpht.com/-eoYWsjdT3og/VLGAdXjYA9I/AAAAAAABDkk/yBMoeLaBf1k/image_thumb%25255B7%25255D_thumb.png?imgmax=800)
![image_thumb[8] image_thumb[8]](http://lh5.ggpht.com/-DqnJWIO_iTg/VLGAqq7l7pI/AAAAAAABDk0/ryqlBIFMbws/image_thumb%25255B8%25255D_thumb.png?imgmax=800)
![image_thumb[10] image_thumb[10]](http://lh3.ggpht.com/-rcWrxLAK12w/VLGA9yeuzVI/AAAAAAABDlE/8CMxn4L5F8s/image_thumb%25255B10%25255D_thumb.png?imgmax=800)
![image_thumb[11] image_thumb[11]](http://lh4.ggpht.com/-E-e7H_jyZpw/VLGBV5RfxuI/AAAAAAABDlU/vT8sKMJ0U8A/image_thumb%25255B11%25255D_thumb.png?imgmax=800)