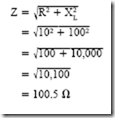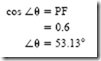FIGURE OF MERIT
It has been pointed out that inductor coils have some resistance. For commercial coils, the inductive reactance is the useful component. The effective resistance is the unwanted component, which must be held to as low a value as possible. The ratio of the inductive reactance in ohms to the effective resistance in ohms is called the figure of merit. The symbol for the figure of merit is the letter Q.
The expression for the figure of merit is
A large Q (high figure-of-merit value) is desirable because it means that the useful inductive reactance is high and the effective resistance is low. A low value of Q means that the resistance component is relatively high. As a result, there is a large power loss.
By referring to the expressions for the figure of merit (Q), it can be seen that an increase in frequency will cause an increase in the inductive reactance and in the effective resistance. However, the inductive reactance and the effective resistance do not increase in the same ratio with an increase in frequency. Thus, the figure of merit (the Q of an inductor coil) must be determined for the frequency, or band frequencies, at which the coil is to be used.
For example, a 0.2-H inductor coil has a resistance of 100 n and is used at a frequency of 10,000 Hz. What is the Q of this coil?
Reactors that have a high Q value are often treated as pure inductors, and the amount of wire resistance is ignored because it is such a small part of the total impedance of the coil. For example, assume that an inductor has a Q of 10. This indicates that the amount of inductive reactance is 10 times greater than the wire resistance. In the following example, it will be assumed that an inductor has a wire resistance of 10 n and an inductive reactance of 100 n. When the total impedance of the coil is computed, it will be seen that the actual amount of wire resistance is only a small part of the coil’s total impedance:
PROBLEM 3
Statement of the Problem
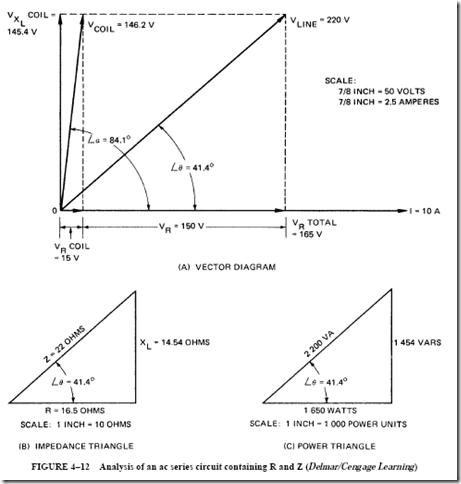
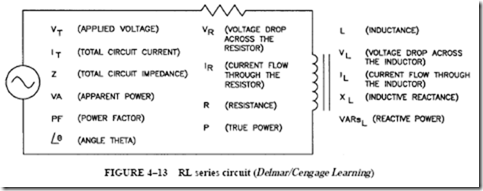
Refer to Figure 4–13. It will be assumed that the inductor has a high Q value and contains zero resistance. The circuit contains 30 n of resistance and 40 n of inductive reactance and is connected to a 240-V, 60-Hz line. Refer to the formulas in the Resistive Inductive (Series) section of Appendix 15 to find the following unknown values:
1. Total circuit impedance (Z)
2. Current flow (I)
3. Voltage drop across the resistor (VR )
4. Watts, true power (P)
5. Inductance of the inductor (L)
6. Voltage drop across the inductor (VL )
7. Volt-amperes-reactive, reactive power (VARs)
8. Volt-amperes, apparent power (VA)
9. Power factor (PF)
10. Phase angle, which indicates how many degrees the voltage and current are out of phase with each other (Le)
Solution
3. In a series circuit, the current is the same at any point in the circuit. Therefore, 4.8 A of current flows through both the resistor and the inductor. The amount of voltage dropped across the resistor can be computed by using the following formula:
4. True power for the circuit can be computed by using any of the power formulas. The following formula is used in this example:
9. Power factor is the ratio of true power compared to apparent power. It can be computed by dividing any resistive value by its like total value. For example, the power factor can be computed by dividing the voltage drop across the resistor by the total circuit voltage, or resistance divided by impedance, or watts divided by volt-amperes. The power factor is generally expressed as a percentage. The decimal fraction computed from the division will, therefore, be changed to a percent by multiplying it by 100. In this circuit the following formula is used:
10. The power factor of a circuit is the cosine of the phase angle. The phase angle indicates how many degrees the current and voltage are out of phase with each other. Because the power factor of this circuit is 0.6, angle theta is as follows:
SUMMARY
• The resistance of a circuit or component to ac is the effective resistance.
• Impedance is the quantity resulting from the combination of effective resistance and reactance.
• Skin effect is the action whereby alternating current is forced toward the surface of a conductor.
• Eddy currents are induced by the alternating magnetic field around a current-carrying conductor in any metal near the conductor.
1. Eddy current losses occur in the iron cores of reactors, transformers, and stator windings in ac generators and motors.
2. Eddy currents are minimized by laminating the cores used in ac equipment.
• Hysteresis losses are caused by a constantly changing magnetic field due to ac.
1. The molecules of the core structure or other metallic material near the conductors of an ac circuit also change direction as the field changes. The result of these changes is molecular friction.
2. Power is required to overcome this molecular friction.
3. Hysteresis loss occurs as heat in the metallic structure.
• Dielectric loss is a small heat loss in the insulation.
1. The insulation of a conductor is subjected to a voltage stress as the impressed voltage rises to a maximum value twice in each cycle.
2. Usually, this loss is so small it can be neglected.
• The effective resistance for an ac circuit is greater than the true ohmic resistance. Effective resistance consists of skin effect losses, eddy current losses, hysteresis losses, and dielectric losses and is represented by the symbol “R.” Power from the circuit is required to overcome the effective resistance.
• The effective (ac) resistance of a reactor can be found by using
• A silicon steel core placed in a coil instead of an iron core will greatly decrease the eddy current and hysteresis losses. As a result, the effective resistance of the reactor is decreased.
• In the high-frequency range, the effective resistance can be many times higher than the dc resistance.
• The vector resolution method yields a right triangle that can be solved using simple algebra. In this method, the vertical components should not be added algebraically to the horizontal components. The equation used with this method has the following form:
![clip_image002[1]_thumb](http://lh3.ggpht.com/-ZGlzWRe16VA/VK7QwF3kSuI/AAAAAAABCww/GmyBiS_-npo/s1600-h/clip_image0021_thumb2.gif "clip_image002[1]_thumb"){kind=small}
Achievement Review
1. Determine the inductive reactance in ohms and the inductance in henrys for the coil shown in Figure 4–3.
2. Determine the inductive reactance in ohms and the inductance in henrys for the coil shown in Figure 4–4.
3. Explain why there are differences in the inductive reactance in ohms and the inductance in henrys in the results of questions 1 and 2, using the same coil.
4. Explain the difference between the true ohmic resistance and the effective resistance of ac components.
5. An electromagnet consists of a coil with a laminated core assembly. This device takes 6 A when connected to a 120-V dc source. When energized from a 120-V, 60-Hz ac source, the current is 2 A. A wattmeter indicates 100 W.
a. Determine
(1) the true ohmic resistance of the coil.
(2) the effective resistance of the coil.
b. Explain why there is a difference between the true ohmic resistance and the effective resistance values for the same coil winding.
6. Determine the following values for the electromagnet coil in question 5:
a. The impedance
b. The inductive reactance
c. The inductance
d. The power factor
7. Explain why the current taken by the electromagnet coil in question 5 decreased from 6 A at 120 V dc to a value of 2 A when connected to a 120-V, 60-Hz ac source.
8. A series circuit containing a noninductive resistance of 3 n and a coil is connected to a 120-V, 60-Hz supply. The current is 25 A. The true power, as indicated by a wattmeter, is 2500 W. Determine
a. the power factor of the series circuit.
b. the effective resistance of the coil.
c. the impedance and inductive reactance for the coil.
d. the power factor of the coil.
9. Determine the following values for the circuit given in question 8:
a. The total circuit impedance
b. The total resistance of the circuit
10. Determine the following values for the circuit given in question 8:
a. The reactive power component for the coil
b. The reactive power component for the entire series circuit
c. The true power in watts taken by the coil
d. The true power in watts taken by the noninductive 3-n resistance
11. Draw a vector diagram for the circuit in question 8. Select convenient scales for the current and voltage. Label all vectors properly.
12. The temperature of a noninductive heater unit is controlled by a reactor. The heater unit and the reactor are connected in series across a 250-V, 60-Hz source. The voltage across the heater unit is 200 V. The voltage across the reactor is 100 V. The current in the circuit is 10 A.
a. Construct a vector diagram for the series circuit.
b. Determine
(1) the power factor of the series circuit.
(2) the power factor of the reactor.
(3) the impedance of the reactor.
(4) the effective resistance of the reactor.
(5) the inductive reactance of the reactor.
13. Determine the following data for the circuit given in question 12:
a. The impedance of the entire series circuit
b. The power expended in the heater unit, in watts
c. The power expended in the coil, in watts
d. The total power expended in the series circuit, in watts
e. The total apparent power taken by the series circuit, in watts
f. The reactive power component, in VARs, for the series circuit
14. Two reactors are connected in series across a 10-V, 10-kHz source. They are spaced so that there is no interaction between their electromagnetic fields. Coil 1 has an effective resistance of 200 n and an inductance of 200 millihenrys (mH). Coil 2 has an effective resistance of 300 n and an inductance of 10 mH.
Determine
a. the total resistance, in ohms.
b. the total inductive reactance, in ohms.
c. the total impedance, in ohms.
d. the current, in milliamperes.
e. the total true power expended in the series circuit, in watts.
15. Explain what is meant by the term figure of merit.
16. A coil has an inductance of 300 microhenrys (μΗ) and a figure of merit of 90 when operated at a frequency of 1500 kHz. Determine the effective resistance of the coil at this frequency.
PRACTICE PROBLEMS FOR UNIT 4
Resistive Inductive Series Circuits
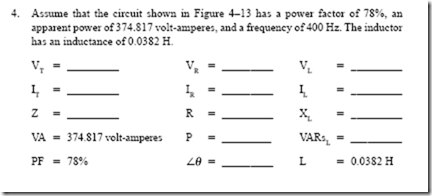
Find the missing values for the following circuits. Refer to problem 3 and the circuit shown in Figure 4–13.
