20–4 THE NEED FOR ADDED ARMATURE COILS
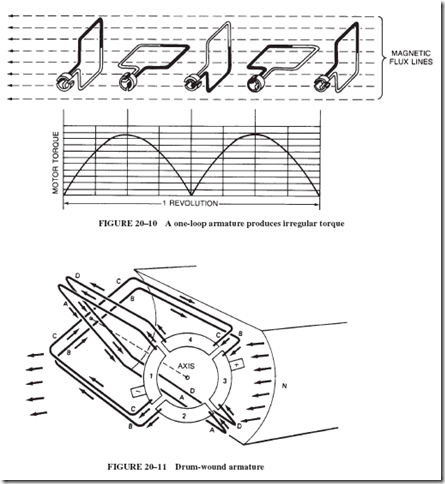
This impractical single-coil armature has a variety of faults, one of which is the irregularity of the torque that it produces. When the loop is horizontal, the force on the wire is at its greatest; when the loop is vertical, there is no force on the wire. This is illustrated by the graph in Figure 20–10. Note the similarity of this graph with that of Figure 19–8, which shows the voltage fluctuations of a simple generator. Other drawings in this chapter, too, will look familiar and will help convey the idea that the design and the internal functions of DC motors are very similar to those of DC generators.
A steadier torque is achieved by using the several coils of a drum-wound armature. The armature shown in Figure 20–11 is just as appropriate in a motor as it is in a generator.
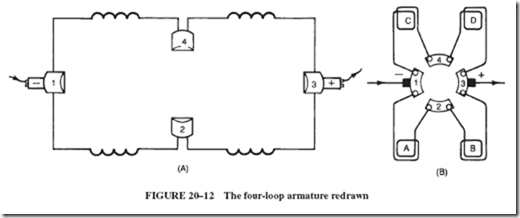
Figure 20–11 shows a similar but simpler drum winding. Assume that the S pole of the field magnet is at the left. (It has been omitted from the diagram in order to show the windings clearly.) Trace incoming electrons from the negative brush through commutat or segment 1 through coil A to segment 2, then through B to segment 3, where the positive brush leads to the completed circuit (not shown) through a source of DC and back to the negative brush. From the negative brush, another circuit can be traced through armature coils C and D to the positive brush. Compare this drum winding with that shown in Figure 19–13 to note the similarity in principle.
The circuitry of this drum-wound armature is redrawn two different ways in Figure 20–12 to reveal the series-parallel arrangement of the four-loop armature. Note that with this arrangement, only one additional pair of commutator segments is needed. All four coils, receiving current from the power source simultaneously, contribute their torque to aid rotation of the armature.
In the preceding section we explained that commutation must take place at the moment a loop enters the neutral plane. In a practical DC motor, the brushes are so arranged that they will short-circuit the loop as it passes through the neutral plane. This will eliminate undesirable sparking between the brushes and the commutator.
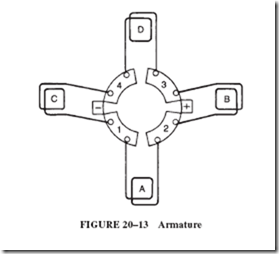
As Figure 20–13 shows, there is no current in coil C because both ends of it touch the same brush. Similarly, there can be no current in coil B. This condition exists at the instant when coils B and C are in a vertical position. Even if there were current in coils B and C, no torque would be produced. Therefore, the absence of current in the coils during this short interval is no disadvantage. At this same instant, coils A and D are in horizontal position, where they are producing maximum torque.
20–5 FROM TORQUE TO HORSEPOWER
Some students may wonder about the significance of torque, since this term has been used so frequently in our discussion of mechanical energy.
Torque is an essential component used in the calculation of the horsepower rating of a machine. Torque by itself does not tell the power of a machine; speed must be taken into account. We can say that
Horsepower is proportional to torque and speed.
Let us see what needs to be done to determine the amount of horsepower developed by a four-coil armature such as the one shown in Figure 20–11. First we need to determine the torque exerted on the armature. This can be done two different ways: experimentally and mathematically.
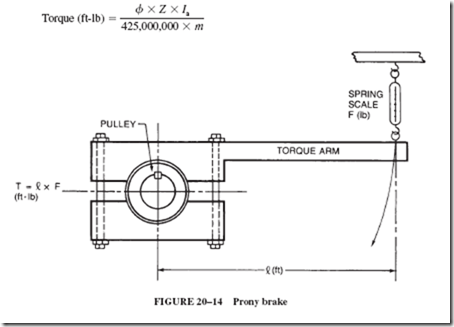
Experimentally, the torque of a motor is measured directly by a device called a Prony brake, as shown in Figure 20–14. Tightening the bolts makes the brake tend to turn along with the motor pulley. The brake arm is restrained by the stationary spring scale; thus, a torque load is placed on the motor. The torque is the product of the effective length (l) of the torque arm in feet times the net force (F) on the scale in pounds.
A more sophisticated device known as an electrodynamometer can be used to measure torque. Such a device, resembling an electric motor, utilizes the interaction of magnetic forces to yield readings of torque when coupled to the shaft of a motor.
Mathematically, the torque exerted on the armature of a motor can be found by use of the following equation:
EXAMPLE 20–1
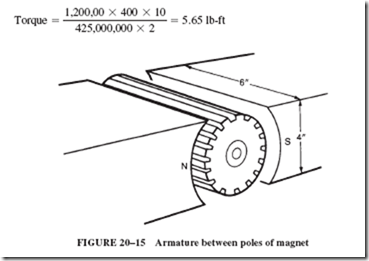
Given: An armature, 6 inches long, placed between magnetic poles as shown in Figure 20–15. The armature carries 200 turns. Flux density equals 50,000 lines per square inch. Current through the armature equals 10 amperes. The armature is designed like the one shown in Figures 20–11 and 20–12.
Find: Torque exerted by the armature.
Solution
200 turns = 400 wires = Z in the formula (every loop has two edges that cut the flux lines). Total number of flux lines to be cut 5 1,200,000 (24 x 50,000).
Calculation of Horsepower
Remember, horsepower is a function of torque and speed. Specifically,
Speed of the motor can be found with a revolutions counter and stopwatch, or a tachometer, or a calibrated stroboscopic light. Tachometers can read the rotational speed directly in revolutions per minute (rpm). Thus, the true mechanical horsepower output (or brake horsepower) can be determined from measurements of torque and speed.
EXAMPLE 20–2
Given: The armature of the preceeding example developing 5.65 pound-feet, turning at 1,250 revolutions per minute.
Find: The horsepower output.
Solution
One horsepower equals 746 watts, so 1.34 horsepower equals 1,000 watts. Assume that this armature has a current of 10 amperes. If it is 100% efficient, a power input of 100 volts 3 10 amperes 5 1,000 watts, or 1.34 horsepower.
If the horsepower output is known, from electrical data, torque can readily be calculated for any known value of rpm.
SUMMARY
• Electrons moving through a magnetic field are pushed sideways in a direction at right angles to the field and to their original direction.
• This fact explains the motion of armatures in DC and AC motors and the controlled movement of electron streams in electrical arcs or cathode-ray tubes.
• A three-finger right-hand rule is used to describe the motor effect.
• Torque is the twisting effect that produces rotation about an axis.
• The quantity of torque is needed for the computation of horsepower.
• DC motors require commutators for the reversal of current through the armature loop every 180°.
• Reversal of current must occur when the wire loop enters the neutral plane.
• The drum armature is an effective way of arranging coils to produce a continuous torque in a motor.
Achievement Review
1. In the sketch below, how should magnet poles be placed so that the wire is moved upward when the switch is closed?
2. What happens to the wire in question 1 if an alternating current (60 cycle) is sent through the wire?
3. A brass strip is fastened to the left terminal in the sketch below and rests in loose contact with the right-hand terminal. What happens when a battery is connected to the terminals
a. With the left one negative?
b. With the right one negative?
7. The center of a 6-foot-long plank is placed on a fulcrum as shown. How much torque is developed if a force of 75 pounds is applied at the end of the plank?
8. Calculate the torque produced on a lap-wound armature of 300 wires. Total armature current is 40 amperes; flux is 2,125,000 lines; m 5 2.
9. How much force does the torque in question 8 produce at the rim of a 4-inch- diameter pulley?
10. The armature in question 8 rotates at 990 revolutions per minute. Calculate the horsepower.