Electrical Measuring Instruments (Another Application of Electromagnetism)
17–1 d’ARSONVAL METERS
The instruments most commonly used to perform measurements are ammeters, voltmeters, and ohmmeters. These instruments are all similar in construction and are modifications of a basic instrument called a galvanometer. Galvanometers are often known as d’Arsonval meters or as permanent-magnet meter movements. The action of galvanometers and that of most measuring instruments depends on the magnetic effects of a small current.
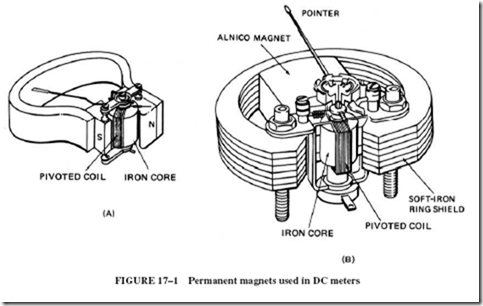
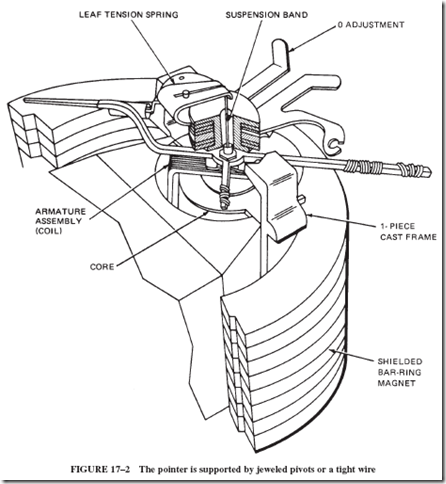
Two forms of permanent magnets for use in DC meters are shown in Figure 17–1. The steel horseshoe magnet in Figure 17–1A has been used in instruments for many years. Also in common use is an Alnico magnet in the form of a rectangular slug; see Figure 17–1B. The flux for this type of magnet divides into the two sides of the soft iron rings surrounding the slug. These rings also act as shields to protect the magnet assembly from outside magnetic disturbances. In both forms of magnet construction, a stationary cylindrical iron core is located between the poles. Due to this core, there is an evenly distributed, uniformly strong magnetic field in the space where the moving coil operates. This uniform field makes it possible to space numbers evenly on the meter scale. To obtain a support that is nearly free of friction, the moving pointer can be supported either by jeweled pivots (similar to those that support the balance wheel of a watch) or by a taut springy wire or band, as shown in Figure 17–2.
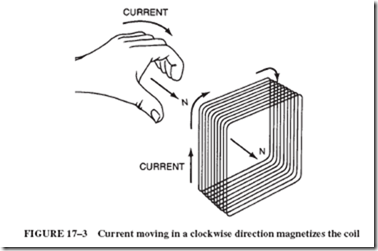
What makes the pointer move? The pointer is fastened to a coil that becomes an electromagnet when there is a current through it. In Figure 17–3, it can be seen that a current moving in a clockwise direction in the coil causes the coil to act as a magnet, having a north pole on the near side and a south pole on the far side.
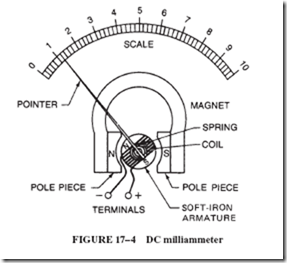
Due to magnetic attraction and repulsion forces, the coil tries to turn between the poles of the magnet so that unlike poles will be as close together as possible. The amount of the turning force depends on the strength of the permanent magnet and on the number of ampere-turns of the movable coil. The springy coil support offers mechanical resistance to the motion of the coil. If the current in the moving coil is increased, the magnetic effect of the coil is stronger and the coil turns even more. As a result, the pointer indicates the increased current on the scale; see Figure 17–4. When the current stops, the spring or twisted band returns the coil and pointer to the 0 mark.
The coil assembly is constructed so that one end of the spring or band is fastened to the moving coil and the other end is stationary. As a result, the spring can serve as a conductor to connect the movable coil to the stationary wiring in the meter.
The meter shown in Figure 17–4, as well as other meters based on this type of construction, operates only on direct current. If an alternating current is applied to this meter, the magnetic poles of the coil reverse rapidly. Since the coil is too large to swing back and forth at the AC frequency of 60 times per second, the coil does not turn at all. A meter that is meant to operate on 1 ampere DC is not damaged by 1 ampere AC, but the meter reads 0 on AC.
A simple galvanometer, by itself, has a very limited use. If a galvanometer is to be used as a current indicator, only a very small current is allowed in the fine wire of the moving coil. Since this coil has a low resistance, it is possible to apply only a very small voltage to the moving coil. The most useful galvanometers are scaled as milliammeters or microammeters. (These meters will indicate how many thousandths or millionths of an ampere pass through the meter.)
17–2 DC AMMETERS
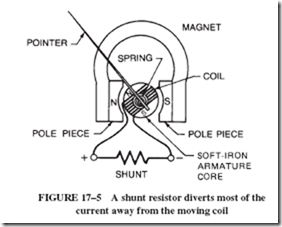
To measure large currents with the galvanometer, a known large fraction of a large current is bypassed through a parallel low resistor called a shunt, as illustrated in Figure 17–5. In this arrangement, only a small fraction of the total current passes through the moving coil. The scale is marked to indicate the total current through the entire am- meter (galvanometer plus shunt circuit).
In order to calculate the value of a shunt resistor, it is necessary to know two other points of information about the meter to be modified:
1. How much current it takes to drive the pointer from its 0 position to the end of the scale. This full-scale deflection current is known as the sensitivity of the meter.
2. The internal resistance of the meter movement.
EXAMPLE 17–1
Given: A d’Arsonval meter movement with a sensitivity of 1 milliamp and internal resistance of 50 ohms.
Find: The value of a shunt resistor necessary to convert this meter into an ammeter with a 5-amp range.
Solution
Look at Figure 17–6. Note that the shunt is a parallel resistance. When there is a 5-ampere current through the meter, only 0.001 ampere passes through the moving coil. The balance of the current, 4.999 amperes, must go through the shunt. Ohm’s law can be used to find the resistance in ohms of the shunt if the potential difference between A and B is known. The voltage between A and B can be found, since it is known that there is a current of 0.001 ampere in the 50-ohm coil.
A 5-ampere ammeter is formed by combining a resistance of 0.01 ohm in parallel with the 1-milliamp meter.
Note: This low shunt value defines the low resistance of an ammeter. Ammeters must have a very low resistance so that the insertion of the meter into a circuit does not reduce the circuit current to be measured.
An experimenter planning to make this meter conversion need not look for a 0.01-ohm resistor in a supply catalog. Copper wire can be used to make a shunt. The
wire is soldered or firmly attached to the meter movement to avoid the introduction of resistance due to a poor contact. The following procedure is used to add a shunt made from copper wire to a meter.
1. Decide on a reasonable length of wire (3 inches, for example).
2. Find the resistance of 1,000 feet of this wire (3 inches 5 0.01 ohm, 1 foot 5
0.04 ohm, and 1,000 feet 5 40 ohms).
3. Refer to Figure A–2 in the Appendix to find the copper wire size that has approximately the same value in ohms per 1,000 feet as the value determined in Step 2. (No. 26-gauge wire has a resistance value close to 40 ohms per 1,000 feet.)
4. Use the available wire size that has the nearest resistance value, and calculate the required length. (Either reverse the previous calculations or use the methods shown in Section 7–4.)
Meter manufacturers generally make meter shunts of a material called manganin (a copper-nickel-manganese alloy) rather than copper. The advantage of using manganin is that the resistance of this material does not change appreciably with temperature changes. Furthermore, since the resistivity of manganin is greater than that of copper, a sturdy assembly that takes up a small amount of space can be made using only a short strip of this material.
17–3 MULTIRANGE AMMETERS
The diagram in Figure 17–7 represents the preferred arrangement of shunts for an ammeter with two scales. The circles marked 12 and 110 represent either binding posts or selector switch contacts. A set of possible values of shunt resistance is shown.
• When the 2-ampere contact is used, the shunt consists of R1 and R2 in series.
• When the 10-ampere contact is used, R2 acts as the shunt; R1 is in series with the moving coil.
This arrangement is called an Ayrton shunt. A three-scale ammeter contains a three-section shunt.
17–4 VOLTMETERS
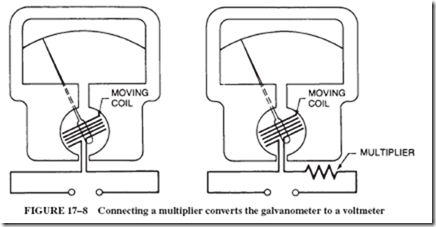
A voltmeter is obtained by connecting a high resistance in series with the galvanometer, as shown in Figure 17–8. Such voltage-dropping, series-connected resistors are known as multipliers, because they multiply the usable range of the basic meter movement.
Unlike an ammeter, a voltmeter must be connected directly across (parallel to) the source of energy. In addition, the voltmeter should be connected in parallel with any device supplied by the measured voltage.
There are two reasons for making the voltmeter a high-resistance instrument.
• Only a tiny current can be permitted through the moving coil.
• The addition of the voltmeter in the circuit should not alter the voltage being measured.
If these statements are not very meaningful, review our discussion of loaded voltage dividers (Section 12–4).
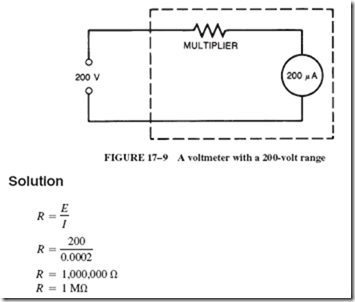
The conversion of a galvanometer (milliammeter or microammeter) to a volt- meter is a simple process both in the calculation of the required resistance and in the construction of the meter, as shown in Figure 17–9. It is necessary to calculate the value of the series resistor that limits the current to the galvanometer’s full-scale capability when the desired voltage is applied. Once again, Ohm’s law is used to determine the necessary value.
EXAMPLE 17–2
Given: A galvanometer with 200-microampere sensitivity.
Find: The value of the multiplier needed to construct a voltmeter with a 200-volt range, as shown in Figure 17–9.
This value is the total resistance of the voltmeter (the moving coil plus the series resistor). In general, the resistance of the moving coil is so small (in the order of 50 to 100 ohms) that it is disregarded. Therefore, a 1-megohm resistor (1 Mohm or 1 MΩ) connected in series with the galvanometer coil results in a 200-volt voltmeter.
Anyone who is concerned about the inaccuracy introduced by disregarding the coil resistance (50 to 100 ohms) in Example 17–2 should consider the following questions. If a resistor marked with a value of 999,900 ohms is issued for a job, how can the technician know if it is 999,900 ohms or 1,000,000 ohms? How accurate is the microammeter movement assumed for the problem? How accurately can a voltmeter be read? In general, lower- priced meters have a 2% accuracy and higher-priced ones have a 1% accuracy.
Multirange voltmeters contain several resistors. The meter range to be used is deter- mined by the choice of binding posts or the selector switch setting.
Figure 17–10 shows calculated values for the series resistors of a multirange meter. The actual values can vary from the stated values by 1% or 2%. With the selector switch at the 2.5-volt position, R 5 2.5/0.001 5 2,500 ohms total (30 ohms in the meter plus 2,470 ohms in the series resistor). At the 25-V position R 5 25/0.001 5 25,000 Ω and at the 250-V position R 5 250,000 Ω.
Sensitivity of Voltmeters
The quality of a voltmeter is indicated by its sensitivity, that is to say, by the amount of current required to force the pointer to the end of the scale (full-scale deflection.) Obviously a very sensitive instrument requires only a minimal amount of current. To meet this condition the meter must have a very large internal resistance.
The sensitivity of a voltmeter is stated in ohms per volt (Ω/V). In Example 17–2, for instance, the 200-volt meter has a resistance of 1,000,000 ohms; therefore, the sensitivity of this meter in ohms per volt is
The multirange voltmeter in Figure 17–10 has a sensitivity of 1,000 ohms per volt on all scales. A large value of sensitivity is desirable, since a high-resistance voltmeter uses a very small current to operate the meter movement. A meter rated at 1,000 ohms per volt will take a current of 1 milliampere for a full-scale reading.
A voltmeter with a sensitivity of 20,000 ohms per volt operates on 50 microamperes (μA) at full scale.
17–5 OHMMETERS
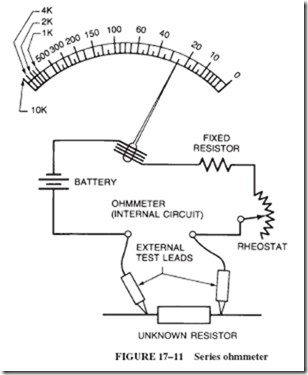
An ohmmeter contains a battery, series resistors, and a galvanometer (microammeter) movement, as shown in Figure 17–11. The battery ranges from 1.5 to 45 volts. The same type of coil assembly is used in the ohmmeter as is used in the types of meters covered previously. An increase in the current in the meter causes the pointer to move to the right. The meter is scaled so that it indicates the amount of resistance in ohms when the meter is placed between the tips of the external test leads.
To use an ohmmeter, the tips of the test leads are first held together (short circuited) and the rheostat (variable resistor) is adjusted so that the meter pointer moves to the right-hand end of the scale and points to the 0 ohms mark. The meter now indicates a condition that is already known: there is no resistance between the test leads. The rheostat adjustment is made to compensate for changes in the resistance of the battery as it ages.
When the test leads are separated, there is no current in the circuit, and the pointer drops back to the left end of the scale to the indication of infinite resistance. (The presence of several inches of air between the test leads means that there is high resistance between them.)
When the test leads are touched to the ends of a resistor of unknown value, the resistance is read directly from the ohms scale. An ohmmeter normally has several ranges, where different combinations of series resistance and battery voltage are used for the individual range.
The ohmmeter shown in Figure 17–11 is called a series ohmmeter. When it is in- stalled in a case containing multiple-contact switches, voltmeter resistors, and ammeter shunts, the resulting assembly is called a multimeter or volt-ohmmeter. Volt-ohmmeters are widely used in testing electronic equipment.
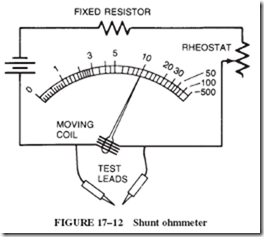
To measure very low resistance values, a shunt ohmmeter is used, as shown in Figure 17–12. The scale reads from left to right because the high resistance permits more current through the meter. Zero resistance in the test lead circuit permits most of the current to bypass the meter.