17–9 CLAMP-ON METERS
All the instruments discussed so far in this chapter operate on magnetic principles. Be aware, though, that modern technology provides meters that employ principles of electronics and induction. Induction, as you will learn in the following chapter, involves transfer of energy from one conductor onto another without physical contact. This energy transfer occurs via the magnetic field that surrounds a current-carrying conductor. This phenomenon allows the technician to measure electrical current in a conductor without
having to break the circuit or otherwise interrupt the service. The instrument used in this manner is called a clamp-on meter, or just clamp meter, also sometimes referred to as an amp clamp; see Figure 17–19. Such instruments have a tapered jaw that can be opened and closed single-handedly by pressure action on a side lever. The jaws are clamped around the conductor in which the current is to be measured.
Although these instruments are primarily used to measure current, they can also be used to obtain voltage readings, in both AC and DC installations. The meter scale is built right into the handheld body of the instrument and can be of either analog or digital design.
Some manufacturers also offer clamp-on accessories that can be attached to a VOM or DMM, thereby providing greater versatility to an existing meter. Figure 17–20 shows how a handheld VOM looks before and after being outfitted with a clamp-on accessory.
17–10 DIGITAL MULTIMETERS
Digital multimeters, such as the one shown in Figure 17–21, have become increasingly popular in the past few years. The most apparent difference between digital meters and analog meters is the fact that digital meters display their reading in discrete digits instead
of with a pointer and scale. Some digital meters have a range switch (similar to the one used with analog meters) that sets the full-range value of the meter. Many digital meters have voltage range settings from 200 millivolts to 2,000 volts. The lower ranges are used for accuracy. For example, assume it is necessary to measure a voltage of 16 volts. The meter will be able to make a more accurate measurement when set on the 20 volts range than it will when set on the 2,000 volts range.
Digital meters that do not contain a range-setting control are known as auto-rang- ing meters. They contain a function control switch that permits selection of the electrical quantity to be measured—such as AC volts, DC volts, ohms, etc. When the meter probes are connected to the object to be tested, the meter automatically selects the proper range and displays the value.
Analog meters change scale value by inserting or removing resistance from the meter circuit. The typical resistance of an analog meter is 20,000 ohms per volt for DC and 5,000 ohms per volts for AC. This means that if the meter is set for a full-scale value of 60 volts, there will be 1.2 megohms of resistance connected in series with the meter if it is being used to measure DC (60 3 20,000 5 1,200,000) and 300 kilohms if is it is being used to measure AC (60 3 5,000 5 300,000). The impedance of the meter is of little concern if it is used to measure circuits connected to a high current source. For example, assume the voltage of a 480-volt panel is to be measured with a multimeter with a resistance of 5,000 ohms per volt. If the meter is set on the 600 volts range, the resistance connected in series with the meter is 3 megohms (600 3 5,000 5 3,000,000). This will permit a current of 160 microamperes to flow in the meter circuit (480/3,000,000 5 0.000160), which would not be enough to affect the circuit being tested.
Now assume that this meter is to be used to test a 24-volt circuit with a current flow of 100 microamperes. If the 60 volts range is used, the meter circuit contains a resistance of 300 kilohms (60 3 5,000 5 300,000). This means that a current of 80 microamperes will flow when the meter is connected to the circuit (24/300,000 5 0.000080). The con- nection of the meter to the circuit has changed the entire circuit operation. This is known as the loading effect.
Digital meters do not have this problem. Most digital meters have an input imped- ance of about 10 megohms on all ranges. This is accomplished by using field effect transistors (FETs) and a voltage divider circuit. A simple schematic for this circuit is shown in Figure 17–22. Notice that the meter input is connected across 10 megohms of resistance regardless of the range setting of the meter. If this meter is used to measure the voltage of the 24-volt circuit, a current of 2.4 microamperes will flow through the meter. This is not enough current to upset the rest of the circuit, so voltage measurements can be made accurately.
Digital Ohmmeters
Digital ohmmeters display the resistance in figures instead of using a meter movement. When using a digital ohmmeter, be sure to notice the scale indication on the meter. For example, most digital meters will display a “k” on the scale to indicate kilohms or an “M” to indicate megohms (recall that kilo means 1,000 and mega means 1,000,000). If the meter is showing a resistance of 0.200 k, it means 0.200 3 1,000, or 200 ohms. If the meter indicates 1.65 M, it means 1.65 3 1,000,000, or 1,650,000 ohms.
Appearance is not the only difference between analog and digital ohmmeters. Their operating principle is also different. Analog ohmmeters operate by measuring the amount of current change in the circuit when an unknown value of resistance is added. Digital ohmmeters measure resistance by measuring the amount of voltage drop across an un- known resistance. In the circuit shown in Figure 17–23, a constant current generator is used to supply a known amount of current to a resistor, RX. Let us assume that the amount of current supplied is 1 milliampere. The voltage dropped across the resistor is proportional to the resistance of the resistor and the amount of current flow. For example, assume the value of the unknown resistor is 4,700 ohms. The voltmeter would indicate a drop of
volts when 1 milliampere of current flows through the resistor.
The scale factor of the ohmmeter can be changed by changing the amount of current flow through the resistor. Digital ohmmeters generally exhibit an accuracy of about 61%.
The ohmmeter must never be connected to a circuit when the power is turned on.
Since the ohmmeter uses its own internal power supply, it has a very low operating voltage. If a meter is connected to power when it is set in the ohms position, it will probably be damaged or destroyed.
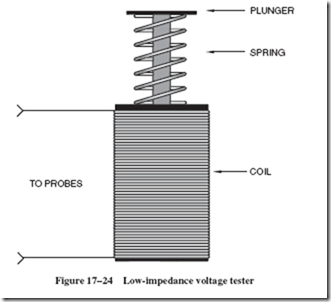
Low-Impedance Voltage Tester
Another device used to test voltage is simply referred to as a voltage tester. This device does measure voltage, but it does not contain a meter movement or digi- tal display. Rather, it contains a coil and a plunger. The coil produces a magnetic field, which is proportional to the voltage the tester is connected to. The higher the voltage the tester is connected to, the stronger the magnetic field becomes. The plunger must overcome the force of a spring as it is drawn into the coil, as shown in Figure 17–24, and acts as a pointer to indicate the amount of voltage the tester is connected to. The tester has an impedance of approximately 5,000 ohms and can generally be used to measure voltages as high as 600 volts. This type of tester has a very large current draw when compared to other types of voltmeters and should never be used to test low-power circuits.
The relatively high current draw of the voltage tester can be an advantage when testing certain types of circuits, however, because the tester is not susceptible to giving the misleading voltage readings caused by high-impedance ground paths or feedback voltages. An example is shown in Figure 17–25. A transformer is used to supply power to a load. Notice that neither the output side of the transformer nor the load is connected to ground. If a high-impedance voltmeter is used to measure between one side of the transformer and a grounded point, it will most likely indicate some amount of voltage. This is due to the fact that ground can act as a large capacitor and permit a small amount of current to flow through the circuit created by the meter. This high-impedance ground path can support only a few microamperes of current flow, but it is enough to operate the meter. If a voltage tester is used to make the same measurement, it will not show a voltage because there cannot be enough current flow to attract the plunger. A voltage tester is shown in Figure 17–26.
SUMMARY
• A DC galvanometer consists of a small coil of wire and a pointer connected in an assembly, moved by magnetic action in the field of a permanent magnet; small spiral hairsprings return the pointer back to the 0 mark on the meter scale.
• Galvanometers are also known as d’Arsonval meters or permanent magnet meter movements.
• An ammeter is a low-resistance meter and consists of a galvanometer and a shunt resistor of low value in parallel with the galvanometer.
• An ammeter is connected in series with the device in which current is to be measured.
• A voltmeter is a high-resistance meter and consists of a galvanometer plus a resistor in series with the galvanometer.
• A voltmeter can be connected directly to a voltage source and must be connected across (in parallel with) the device in which voltage is to be measured.
• An ohmmeter consists of a galvanometer, dry cells, and series resistors. It measures the resistance between the test leads and the instrument. An ohmmeter must be used in a dead circuit; this instrument must NOT be used on resistors that have current in them from some other source.
• A wattmeter contains a voltage coil across the line and a current coil in series with the line. A wattmeter will indicate either DC or AC watts.
• There are more electrons on the negative terminal of a device (such as a meter, resistor, or battery) than there are on its positive terminal. (Remember this point when you must determine either the polarity of a device or the electron current direction. Electrons move from the negative toward the positive terminal.)
• Electrical measurements are based on the accuracy of mathematical formulas and the reliability of precision instruments.
• Wheatstone bridges are laboratory-type, precision instruments for the measurement of unknown resistors.
• Wheatstone bridges operate on the principle that the voltage drops in a series circuit must equal the applied voltage.
• Clamp-on meters permit current readings without interrupting the circuit.
• Digital voltmeters generally maintain the same input resistance regardless of the range setting.
• Some digital meters are auto-ranging.
• Digital ohmmeters measure resistance by measuring the voltage drop across an un- known resistor when a known amount of current flows through it.
• Low-impedance voltage testers contain a coil and a plunger rather than a meter movement or digital display.
• Low-impedance voltage testers do not give misleading voltage readings caused by high- impedance ground paths or feedback voltages, an advantage when testing certain circuits.
Achievement Review
1. State the purpose of each of the following: the hairsprings in a galvanometer, a shunt in an ammeter, and a series resistor in a voltmeter.
2. Under what conditions is each of the following devices used: a Wheatstone bridge, a megohmmeter?
3. Diagram the internal circuit of a voltmeter, an ammeter, an ohmmeter, and a wattmeter.
4. The user of an ohmmeter finds that when the test leads are shorted, the adjustment does not cause the pointer to move to the 0 mark. Instead, the pointer stops at about R 5 5. Why?
5. Calculate the resistance of the shunt required to convert a 100-microampere meter with a 40-ohm moving coil to a 10-milliampere meter.
6. Calculate the series resistor required to convert the 100-microampere meter in question 5 to a voltmeter with a full scale of 100 millivolts.
7. Calculate the series resistor required to convert the 100-microampere meter in question 5 to a voltmeter scaled to 100 volts.
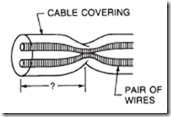
8. A pair of #28 AWG copper wires in a telephone cable is accidentally short- circuited. A Wheatstone bridge is connected to the accessible ends of the wires. The values for the resistances are as follows: R1 5 100 Ω, R2 5 327.8 Ω, R3 5 100 Ω. Using the formula R1 RX 5 R2 R3, calculate the distance from the accessible end of the cable to the point where the pair of wires is shorted. (The ambient temperature is 70°F.)
9. a. Using the data from the illustration in Figure 17–7, calculate the voltage across the moving coil and the voltage across the shunt when 2 amperes pass through the meter (use terminals 2 and – ).
b. Using the 10 and – terminals, find the voltage across the moving coil and the voltage across the shunt when 10 amperes pass through the meter.
10. Two resistors, A and B, are connected in parallel. This combination is placed in series with a third resistor, C. This entire group is connected across a 120-volt DC supply. The resistance of A is 20 ohms. The current in B is 3 amperes, and the current in C is 5 amperes.
a. Determine the voltage across A, the resistance of B, and the resistance of C.
b. If resistor A is accidentally open-circuited, find the new voltages across resistor A and resistor C.
11. A 0- to 150-volt voltmeter has a resistance of 2,000 ohms per volt. It is de- sired to change this voltmeter to a 0- to 600-volt instrument by the addition of an external multiplier. What is the resistance, in ohms, of this external multiplier?
12. A 0- to 150-volt DC voltmeter has a resistance of 100 ohms per volt.
a. What is the instrument resistance?
b. What is the instrument full-scale current?
c. Extend the range of the voltmeter to 750 volts by adding an external multi- plier. What is the resistance of this external multiplier?
d. What is the power dissipation of the external multiplier when the voltmeter is used to measure 750 volts?
13. A d’Arsonval movement has a full-scale deflection at 25 milliamperes, and the coil has a resistance of 2 ohms.
a. What is the resistance of the multiplier required to convert this instrument into a voltmeter with a full-scale deflection at 300 volts?
b. What is the resistance of the shunt required to convert this instrument into an ammeter with a full-scale deflection at 25 amperes?
14. The power to a 25-watt lamp is being measured with a voltmeter and an am- meter. The voltmeter has a resistance of 14,160 ohms. The meter is connected directly across the lamp terminals. When the ammeter reads 0.206 ampere, the voltmeter reads 119 volts.
a. What is the true power taken by the lamp?
b. What percentage of error is introduced if the instrument power is neglected?
15. A DC instrument has a resistance of 2.5 ohms. It gives a full-scale deflection when carrying 20 milliamperes.
a. What is the resistance of the shunt required to give the instrument a full-scale deflection when the current is 10 amperes?
b. What resistance is connected in series with the instrument movement so that a full-scale deflection occurs when the instrument is connected across 150 volts?
16. The resistance of a 0- to 50-millivoltmeter is 10 ohms. This meter is connected with an external shunt in a circuit in which the current is 100 amperes.
a. Draw a diagram showing the method of connecting the instrument and the shunt in the circuit.
b. What instrument current causes full-scale deflection?
c. Determine the resistance of the shunt that is used with the instrument to cause a full-scale deflection.
17. Why are electronic wattmeters replacing dynamic wattmeters?
18. Digital meters that do not contain a control to set the range for measuring volt- age, current, or resistance are known as meters.
19. Most digital voltmeters exhibit an input resistance of about 10 MΩ on all ranges.
How is this accomplished?
20. Explain the difference in operating principle between analog ohmmeters and digital ohmmeters.