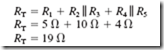Series-Parallel Circuits and Loaded Voltage Dividers
12–1 SIMPLIFYING SERIES-PARALLEL CIRCUITS
Pure series circuits and pure parallel circuits are seldom encountered in the practical applications of electricity and electronics. It is more common to deal with circuits that combine the aspects of both.
This chapter will introduce you to seemingly complicated circuit problems that may be solved by the same principles you have learned in the last two chapters. These principles can be summarized as follows:
In series circuits, current is the same; voltages add. In parallel circuits, voltage is the same; currents add.
There is no one formula that can be applied to an entire circuit to obtain the desired answer or answers. Ohm’s law must be applied first to one part of the circuit and then to another part. This procedure will lead from what we know to what we need to find.
EXAMPLE 12–1
Given: The circuit shown in Figure 12–1A.
Find: The total circuit resistance..
Solution
a. Identify all parallel combinations and solve for their equivalent resistance values.
Note the symbols R2 7 R3 and R4 7 R5 used to identify these values.
The symbol 7 means “the parallel combination of.” In the computation here, R2 7 R3 is a symbolic way of saying “the equivalent resistance of the parallel combination of R2 and R3.”
b. Redraw the circuit with the computed equivalent values, as shown in Figure 12–1B.
c. Redraw the circuit as a single resistance, as shown in Figure 12–1C.
EXAMPLE 12–2
Given: The circuit shown in Figure 12–2A.
Find: The total circuit resistance.
Solution
Note: R4 is not in parallel with R2 as it may seem upon casual inspection. Instead, it is the series combination of R3 and R4 that is connected parallel to R2. The result is a single parallel circuit with an equivalent resistance of 2.73 ohms.
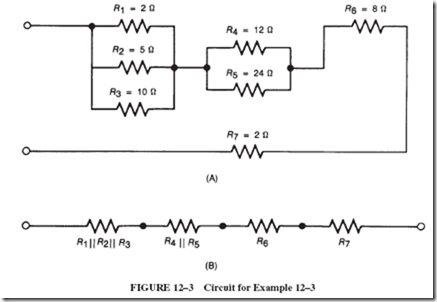
EXAMPLE 12–3
Given: The circuit shown in Figure 12–3A.
Find: The total circuit resistance..
Solution
Note: The parallel combinations have been identified for you, and the circuit has been redrawn accordingly, as shown in Figure 12–3B. Now it is your task to compute the equivalent values and to finish the problem. The answer is 19.25 ohms.
EXAMPLE 12–4
Given: The circuit shown in Figure 12–4.
Find: The total circuit resistance.
Solution
Once again, the parallel combinations have been identified for you, and the circuit should be redrawn accordingly. Finish it! The answer is 50 ohms.
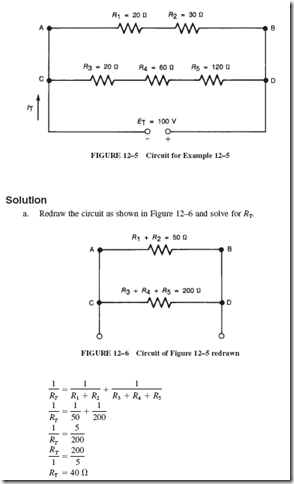
EXAMPLE 12–5
Given: The circuit shown in Figure 12–5.
Find:
a. The total circuit resistance RT.
b. The current from A to B.
c. The current from C to D.
d. The total current supplied.
e. Each voltage drop across the resistors.
12–2 KIRCHHOFF’S VOLTAGE LAW
We will now use Example 12–5 to develop the ideas expressed by Kirchhoff’s voltage law, which states:
Around any closed loop, the algebraic sum of the voltages is equal to zero.
Or, as some people prefer it:
Around any closed loop, the sum of the voltage drops is equal to the sum of the voltage rises.
Note that one key phrase is common to both statements, namely: “Around any closed loop . . .”
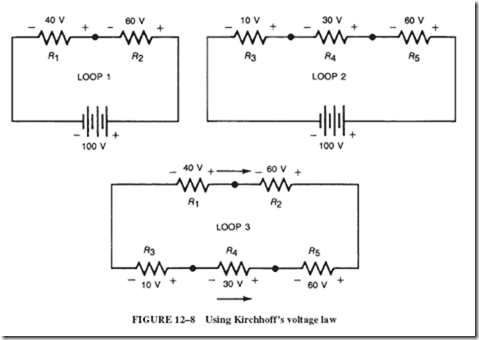
The problem of Example 12–5 has three closed loops, as shown in Figure 12–8.
Recall our convention of determining the polarity of a voltage drop. This was fully explained in Section 10–9, which you may have to review before you continue.
Tracking the loops in a clockwise direction, we obtain the following information:
This, indeed, confirms Kirchhoff’s voltage law. Incidentally, the note at the conclusion of Example 12–5 is another statement confirming the truth of Kirchhoff’s voltage law.