12–3 KIRCHHOFF’S CURRENT LAW
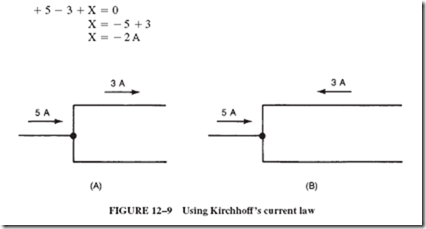
Consider a junction of three wires, called a node, as shown in Figure 12–9. Information is given concerning the amount and direction of the current in only two of the wires. With this information, however, it is possible to deduce the amount and direction of the unknown current in the third wire. This is what Kirchhoff’s current law is all about. This law can be stated as follows:
At any node, the algebraic sum of the currents equals zero.
You have probably reasoned already that, in Figure 12–9A, a current of 2 amperes is flowing from left to right, and in Figure 12–9B, a current of 8 amperes is flowing from left to right. It appears that the total amount of current flowing into a junction must equal the current coming out. In short: What goes in must come out.
To apply Kirchhoff’s current law, we arbitrarily assign positive direction to currents flowing into the junction and negative direction to currents coming out of the juncion. If X denotes the unknown quantity, it follows that for Figure 12–9A
Remember, the negative sign of the answer denotes the current flowing away from the junction.
The following example is designed to demonstrate the usefulness of Kirchhoff’s volt- age and current laws in the solution of complex circuits.
EXAMPLE 12–6
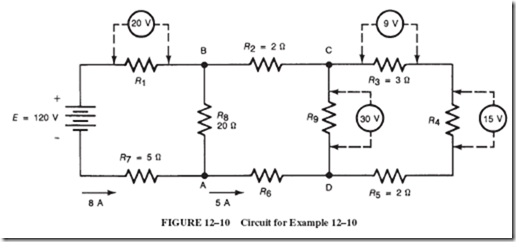
Given: The circuit shown in Figure 12–10.
Find: All voltage drops, all resistance values, and all branch currents that are not given.
Solution
Note: Remember, our aim is to find all branch currents.
Applying the principles of Kirchhoff ’s current law, the following facts can be confirmed.
• The 8-ampere current entering node A divides in such manner that 3 amperes will flow upward (from A to B) through R8.
• The power source provides a line current of 8 amperes to the circuit. The same 8-ampere current returns to the power source through R1. Therefore, I1 5 8 amperes.
• At point B the current I8 combines with the current I2 to constitute the 8-ampere line current leaving point B toward the left. This implies that I2 must be equal to 5 amperes.
• Knowing that I2 5 5 amperes and I3 5 3 amperes, it follows that I9, flowing from D to C, equals 2 amperes.
• This completes all the computations for the different branch currents. We suggest that you enter the new information into your diagram, as shown in Figure 12–10.
Using Ohm’s law, we can now find the voltage drops of the first loop as follows:
12–4 LOADED VOLTAGE DIVIDERS
At this time you should have a complete understanding of simple voltage dividers, which were covered in Chapter 10. A brief review of Sections 10–8 through 10–11 is highly recommended before proceeding with this section.
To understand the concept of loaded voltage dividers, consider the following hypothetical problem.
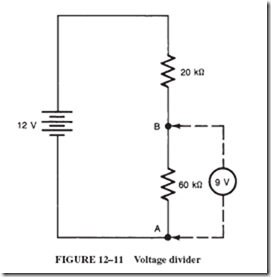
Let us assume you own a small portable radio that requires a 9-volt battery. All you have available is a 12-volt battery and a few resistors, so you decide to construct a voltage divider like the one shown in Figure 12–11. The values of the resistors are chosen in a ratio of 1 to 3; therefore, the supply voltage will be divided in a like ratio and you have your desired 9-volt supply, or so you think.
But watch what happens when you connect your radio across terminals A and B. Your radio, too, has a resistance, say 30 kilohms. When you connect the radio across the 60-kilohm resistor, you have a parallel circuit from A to B with an equivalent resistance of 20 kilohms. Now your voltage division is in a ratio of only 1 to 1, and your radio receives only 6 volts instead of the anticipated 9 volts. The radio has loaded the voltage divider. In other words, simple (unloaded) voltage dividers are series circuits, while loaded voltage dividers represent series-parallel circuits.
Note: The word load refers, of course, to any device that draws current from the voltage divider. That much is probably obvious to you. What may be new is the fact that
A small resistance is a big load and a large resistance represents a small load.
You see, the nominal size of a load is determined by the current it draws, not by its resistance value. Knowing this, you may appreciate the fact that the output from a voltage divider changes with varying load conditions. This f luctuation of voltage output is referred to as voltage regulation.
A high percentage of voltage regulation is undesirable and can be improved by minimizing the value of the so-called bleeder resistor. The bleeder is defined as that portion of the voltage divider that is connected parallel to the load.
The design of a voltage divider begins with choosing the size of the bleeder resistor so that it will draw at least 10% of the load current. An example will illustrate this.
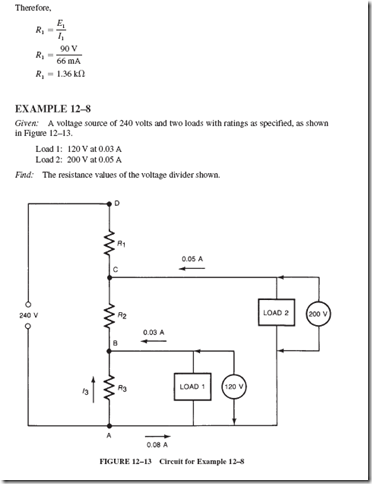
EXAMPLE 12–7
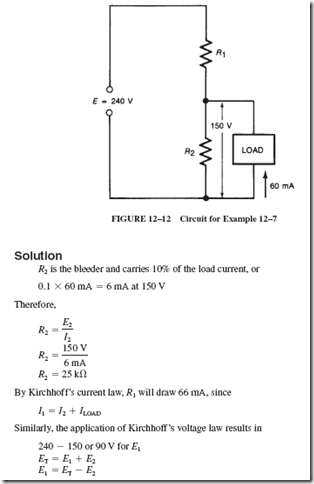
Given: A power source of 240 volts and a load that draws 60 milliamps at 150 volts, as shown in Figure 12–12.
Find: The proper resistance values of the voltage divider.
SUMMARY
• Series-parallel circuits can be redrawn in a simplified form by substituting equivalent resistors for parallel and series combinations.
• Kirchhoff’s voltage law states that the algebraic sum of voltages around any closed
loop equals zero.
• Kirchhoff’s current law states that the algebraic sum of currents at a node equals zero.
• Practical voltage dividers are series-parallel circuits whose resistance values are determined by the load(s).
• Heavy loads are characterized by a low resistance, and small loads are characterized by high resistance.
• Low-value bleeder resistors improve the voltage regulation of voltage dividers.
Achievement Review
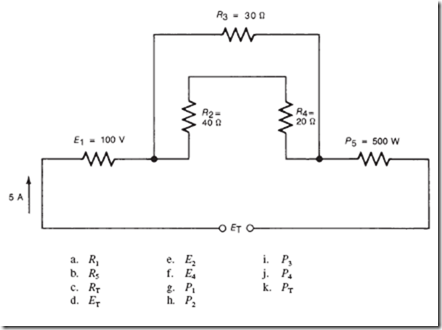
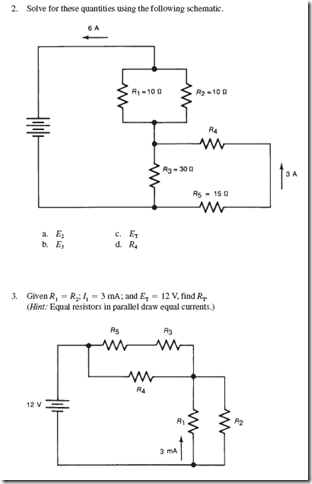
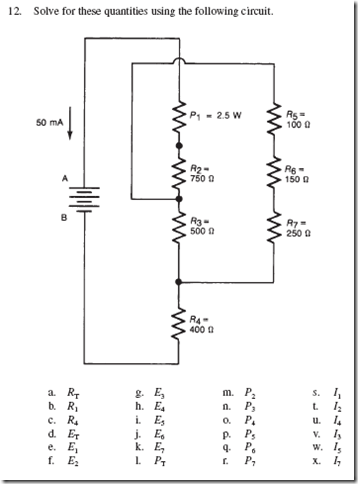
1. Solve for these quantities using the following schematic.
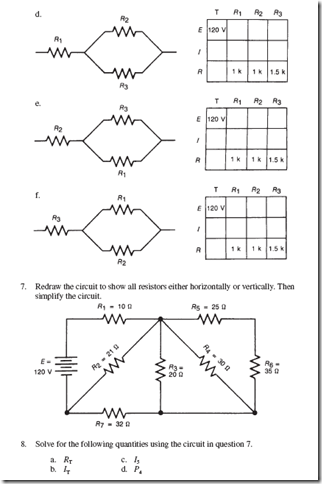
9. Solve the following circuit to find all voltage drops and all the currents. Sug- gested sequence of operations:
a. Find RT and IT.
b. Apply Kirchhoff’s and Ohm’s laws to find unknown.
c. Enter all your answers in the drawing. (It helps to use different colors.)
d. Confirm your results by adding voltages around each loop.
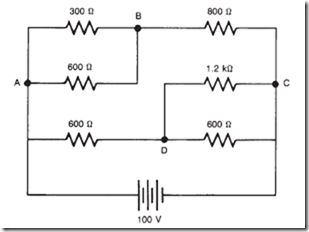
10. Find
a. The total resistance of the circuit.
b. The voltage drop across A–B.
c. The current in the branch A–D–C.
d. The potential difference between B and D.