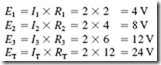Series Circuits
10–1 CHARACTERISTICS OF SERIES CIRCUITS
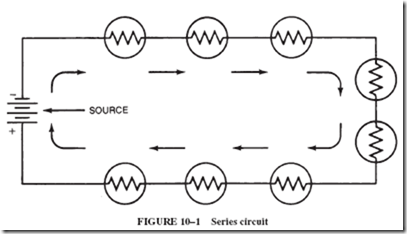
When any number of devices are connected so that there is only a single circuit path for electrons, the devices are in series. Each device has the same amount of current in it; see Figure 10–1.
Christmas tree lights are generally connected in series. For many years such an arrangement could be bothersome because all lamps would fail when any lamp burned out. Recent innovations have eliminated this nuisance problem by “shunting” a bypass around the defective filament. This desirable improvement was necessary because when any one lamp burns out, its filament is removed from the circuit, thereby interrupting the current. Each device in a series circuit has the same current through it as every other device in the circuit, since there is only one path for the electrons to travel.
10–2 THE VOLTAGE DROP
The eight lamps shown in Figure 10–1 share the 120 volts supplied and, assuming all lamps in the circuit to be of equal size, they will share alike. In other words, each lamp has a potential difference of 15 volts (120/8) across its terminals. These individual voltages appearing across each resistance of a series circuit are known as voltage drops.
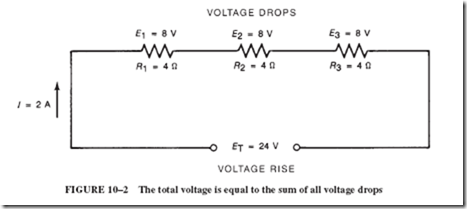
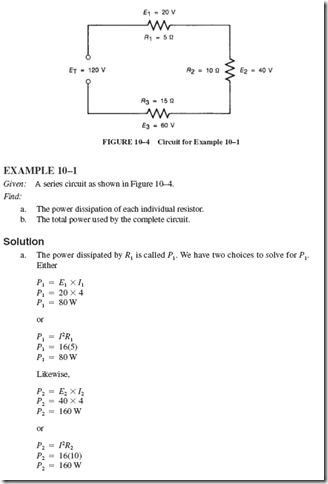
To reinforce this idea, let us consider the circuit of Figure 10–2. Let us first examine the nomenclature used in this schematic diagram. Note that all resistors have been labeled with subscripts: R1, R2, and R3. Since all the resistors are equal in size, we may assume that they will share the supply voltage of 24 volts equally among themselves. These voltage drops of 8 volts each are labeled correspondingly: E1, E2, and E3. After 8 volts are dropped at R1, only 16 volts are left for the remainder of the circuit.
These examples should confirm that
The total voltage of a series circuit is equal to the sum of all the voltage drops.
Mathematically stated,
ET = E1 + E2 + E3 + . . . + En
This equation is sometimes explained by saying that the sum of the voltage drops equals the sum of the voltage rises. In this case, E1, E2, E3, etc., represent the voltage drops, and ET the voltage rise. A voltage rise can thus be explained as a voltage source, as shown in Figure 10–2.
10–3 RESISTANCE AND CURRENT IN SERIES CIRCUITS
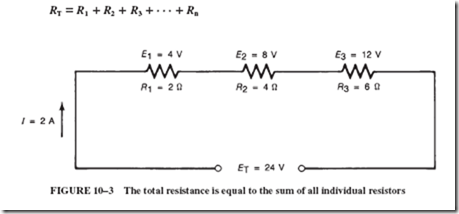
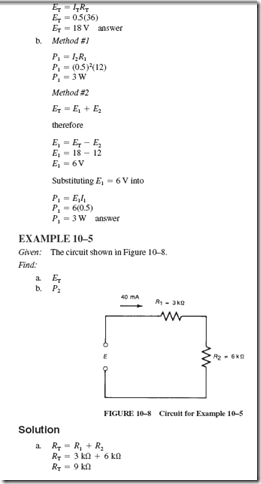
Compare the circuit in Figure 10–2 with that of Figure 10–3. Can you detect the similarities and the differences? Both circuits consist of three resistors attached in series to a 24-volt supply. Both circuits have 2 amperes flowing through the resistors. By Ohm’s law, we compute the total resistance (RT) that is connected to the battery.
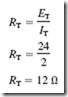This may have been obvious to you right along. After all, if you add all the resistances in each circuit, you obtain the same result for RT, namely, 12 ohms. In other words,
The total resistance of a series circuit is equal to the sum of all individual resistors.
Mathematically stated,
This idea of adding individual values does not apply to the current. You may remember our earlier statement, in Section 10–1, that the current in a series circuit has the same value everywhere. The current in these two circuits is 2 amperes at any given point. Yet it is customary to label the current with different subscripts as it flows through different components. We refer to it as I1 when it flows through resistor R1 and, correspondingly, I2 when it flows through R2. The current in the supply line is named IT to correspond to the supply voltage ET. Some people prefer to use the subscript S for the word supply, and write IS and ES. Just remember, by whatever name you call it,
The current in a series circuit is the same everywhere.
Mathematically stated,
So much for the similarities. Now let us have a quick look at the difference between the two circuits. Have you noticed? The voltage drops are different when the resistors are different. In fact, the voltage drops are proportional to the values of the resistors. For instance, if one resistor is twice as large as another, its voltage drop, too, will be twice as large. This, of course, can be confirmed by use of Ohm’s law. We compute the following for the circuit in Figure 10–3:
From now on, as you can see, we must use matching subscripts whenever we use Ohm’s law or the power equations. This idea will be illustrated by the solved sample problems in the next two sections.
The characteristics of series circuits can be summed up in three rules, which can be employed using Ohm’s law to solve the values of any series circuit that contains only one power source. These rules are:
1. The sum of the voltage drops across the individual circuit components is equal to the applied voltage. (Voltage drops add.)
2. The current is the same through all components in a series circuit. (Current remains the same.)
3. The total resistance is equal to the sum of all resistive components in the circuit.
(Resistance adds.)
10–4 POWER CONSUMPTION IN SERIES CIRCUITS
You should recall, from our discussion in Chapter 7, that the wattage rating of resistors is an important specification. With our knowledge of the power equations, determining the power dissipation of an individual resistor, as well as an entire circuit, becomes an easy task. The following example will illustrate.
10–5 CALCULATION OF SERIES CIRCUIT QUANTITIES
This section presents a number of solved problems to demonstrate the proper techniques of solving series circuit problems. Study them carefully.
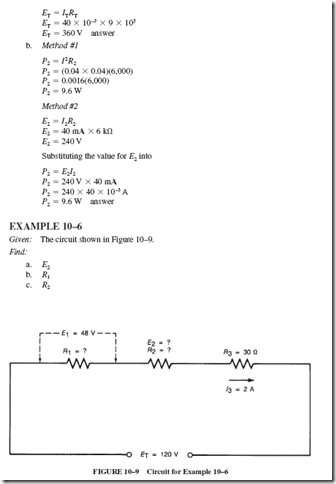
EXAMPLE 10–2
Given: An antique radio circuit in which the heating elements of five radio tubes are connected as shown in Figure 10–5 and a voltmeter connected across R5 that reads V. (Historical note: This was a common method of radio construction until the late 1950s, when vacuum tubes began to be supplanted by solid-state electronic devices.)
