5–4 SIGNIFICANT DIGITS
Most practical calculations are generally limited to only three significant digits. What does this statement mean? An example will make this clear.
Assume that some friends you know want to brag about the performance of their new automobile. They tell you that they clocked their speed at 104.6784935 miles per hour. (That is downhill with a tailwind blowing.) How would you react to such information, short of calling them liars? Obviously, speedometers do not give 10-digit readouts. Neither do electrical measuring instruments. Most such instruments (called meters) are limited to three-digit accuracy.
Does this mean that electricity is a sloppy science? Quite the contrary! Electronic measurements can be extremely precise; however, practical considerations would recommend that all numbers be limited to three-digit accuracy. Thus, your friends should have reported their speed as 105 miles per hour.
5–5 ROUNDING OFF TO THREE SIGNIFICANT DIGITS
The last statement may have puzzled you. “Why 105 miles per hour?” you might ask. “Why not 104 miles per hour?” The answer to this is determined by the fourth digit. If the fourth digit is 5 or greater, the third digit should be rounded off to the next higher number. On the other hand, if the fourth digit is smaller than 5, it would be dropped along with all successive digits.
It must be pointed out that 0’s are not considered to be significant unless they are preceded by a number other than 0. Let us consider, for instance, the number 0.00020543. In rounding off such a number, it is important to recognize that the numeral 2 is the first significant digit to be retained. Thus, the answer would be reported as 0.000205, or in scientific notation as
2.05 x10–4
As stated earlier, in electrical theory we are often confronted with very large or very small numbers. To overcome the inherent inconvenience of dealing with such awkward numbers, it is customary to modify the basic measuring units by attaching a prefix to their multiples or submultiples.
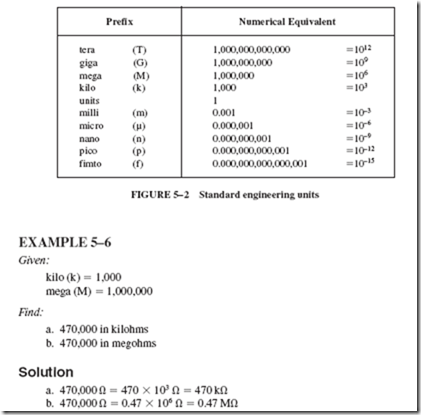
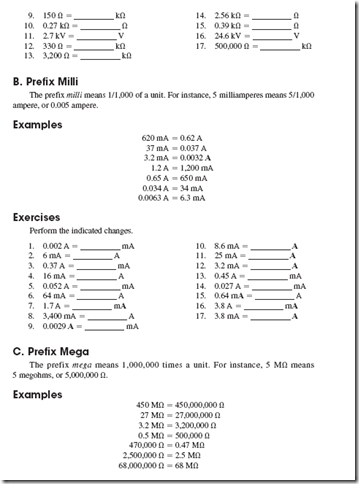
For instance, 1,000 volts can be called 1 kilovolt. This example shows that the word kilo stands for a multiple of l,000, or 103. In other words, 1 kilo means 1,000 times a unit.
Can you see that with this convention we can express a number like 27,000 ohms as 27x 103 = 27 kilohms, or just 27 kΩ?
Now that we understand the word kilo, let us have a look at Figure 5–2 to become acquainted with some other prefixes. Note that these prefixes, commonly called engineering units or engineering notation, are in steps of 1,000 instead of 10. Engineering units are commonly used throughout the electrical field. Many scientific calculators have a function indicated as ENG that displays the answers in engineering units. Scientific notation units will be given in steps of 1,000 instead of 10 when this function is activated. The advantage of using engineering notation is that the calculator will display scientific notation in steps of 1,000 only, which corresponds to common engineering prefixes. When a calculator is not set for engineering notation, an answer may appear as
Note that the decimal point in the answer has moved and the exponent is now 203 instead of 202. The scientific notation unit 203 corresponds to milli, so the answer can be read as 12.689764 milli.
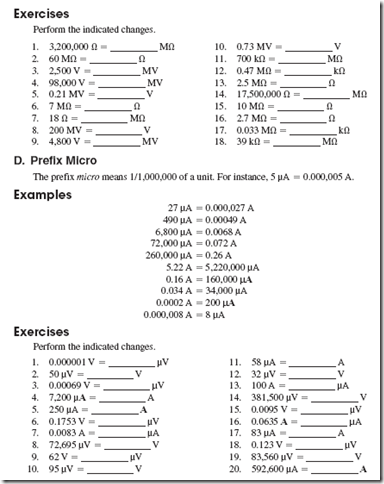
Note that both uppercase letters and lowercase letters are used for letter symbols. It is especially important to distinguish between M for mega and m for milli. Note also the letter symbol for micro—the Greek letter μ (pronounced: myoo).
EXAMPLE 5–5
Given:
More examples are shown in conjunction with the Achievement Review practice problems at the end of the chapter.
5–7 MULTIPLICATION AND DIVISION WITH POWERS OF 10
Even though it is assumed that all students of electricity have a basic knowledge of algebra, it may be helpful to briefly refresh our memory.
Rule #1 (Multiplication): When powers of 10 are to be multiplied, add their exponents. For instance,
SUMMARY
• Powers of 10 are useful in making computations involving very large or very small numbers.
• Scientific notation mandates that a decimal point be placed behind the first significant
digit of a number expressed in powers of 10.
• Three-digit accuracy is generally sufficient for quantities related to practical applications. Numbers should be rounded off accordingly.
• Rounding off to three digits demands a look at the fourth digit to determine whether to round up or down.
• Metric prefixes are used in the electrical trades to describe multiples or submultiples of basic units. Examples are kilovolt, milliampere, and megohm.
• In multiplying powers of 10, the exponents are added.
• In dividing powers of 10, the exponent of the divisor is subtracted from the exponent of the dividend.
Achievement Review
PART I ROUNDING OFF MATHEMATICAL ANSWERS
Key Ideas
1. It will be assumed that electric circuit quantities are sufficiently accurate to justify solutions containing three significant digits.
2. A sequence of significant figures never begins with 0.
3. The fourth significant digit determines whether the third figure should be rounded off.
4. If a number has less than three digits, fill in the rest with 0’s. Thus, 75 5 75.0.
Examples
 PART IV ELECTRICAL UNITS, SCIENTIFIC NOTATION, CONVERSION, AND ABBREVIATIONS
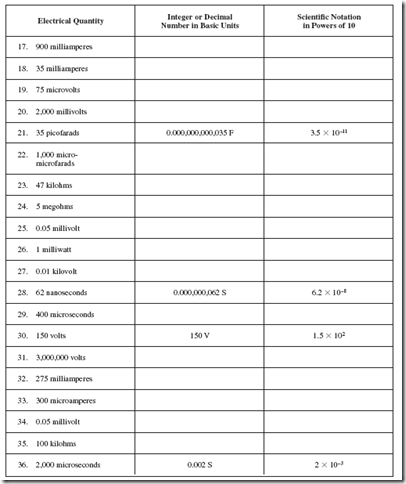
PART IV ELECTRICAL UNITS, SCIENTIFIC NOTATION, CONVERSION, AND ABBREVIATIONS
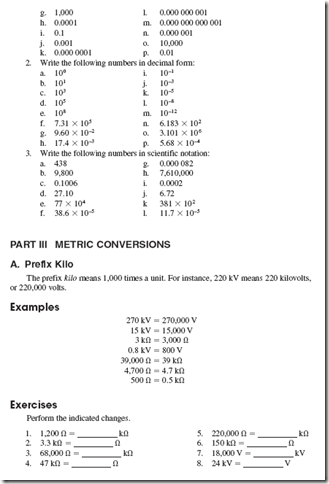
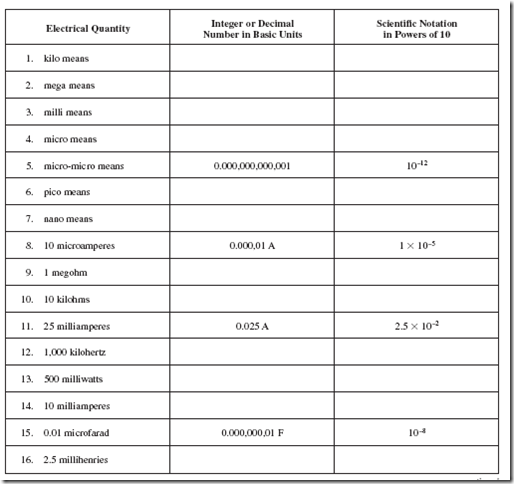
Express each of the following electrical terms, units, or numbers as integers or decimal numbers, as well as powers of 10 (scientific notation).
Note: For this task, we have included some electrical units we have not explained yet but that are important for the aspiring electrical worker to know. These are the units of:
• Electrical energy—called the watt-hour (Wh)
• Frequency—called the hertz (Hz)
• Electrical capacity—called the farad (F)
• Inductance—called the henry (H)
Some answers have been provided throughout this assignment so that you, the student, may have guidelines for this task. Be sure to study all these examples before you begin.
A.