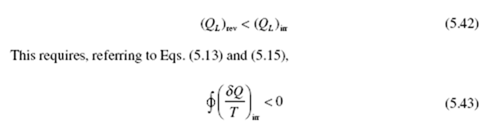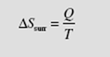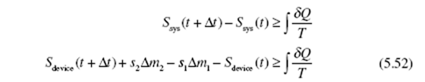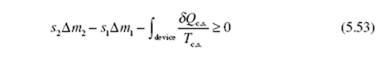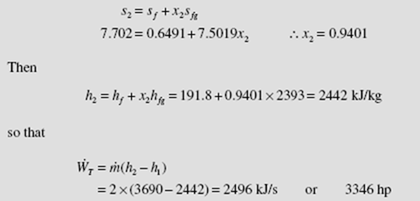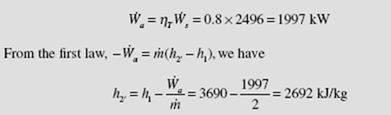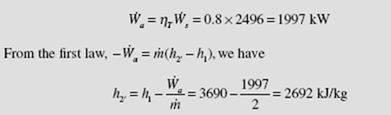5.7 The Inequality of Clausius
The Carnot cycle is a reversible cycle and produces work which we will refer to as Wrev. Consider an irreversible cycle operating between the same two reservoirs, shown in Fig. 5.9. Obviously, since the Carnot cycle possesses the maximum possible
Figure 5.9 A reversible and an irreversible engine operating between two reservoirs.
efficiency, the efficiency of the irreversible cycle must be less than that of the Carnot cycle. In other words, for the same amount of heat addition Q , we must have
Wirr < Wrev (5.41)
From the first law applied to a cycle (W = Q – Q ) we see that, assuming that (Q ) and (Q ) are the same,
since the above integral for a reversible cycle is zero.
If we were considering an irreversible refrigerator rather than an engine, we would require more work for the same amount of refrigeration Q . By applying the first law to refrigerators, we would arrive at the same inequality as that of Eq. (5.43). Hence, for all cycles, reversible or irreversible, we can write
This is known as the inequality of Clausius. It is a consequence of the second law of thermodynamics. It will be used to establish entropy relationships for real processes.
5.8 Entropy Change for an Irreversible Process
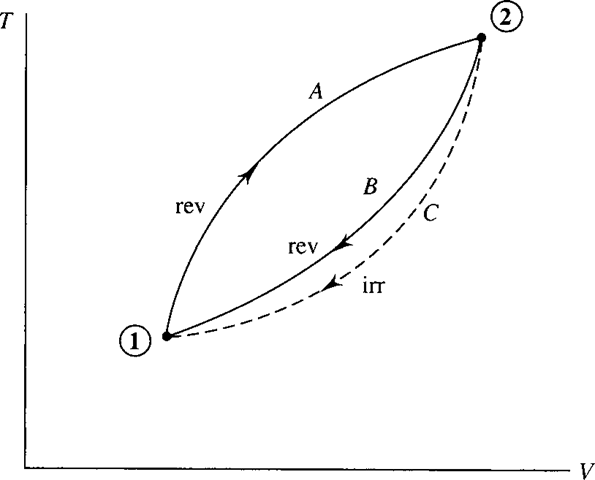
Consider a cycle to be composed of two reversible processes, shown in Fig. 5.10. Suppose that we can also return from state 2 to state 1 along the irreversible process marked by path C. For the system experiencing the reversible cycle we have
Figure 5.10 A cycle that includes the possibility of an irreversible process.
For the system experiencing the cycle involving the irreversible process, the Clausius inequality demands that
The equality holds for a system experiencing a reversible process and the inequality for an irreversible process.
We can also introduce the entropy production σ into Eq. (5.48):
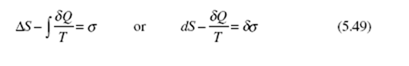where we have used δσ since it is a path dependent function. It is obvious that entropy production is zero for a reversible process and positive for an irreversible process, i.e., any real process.
Relationship (5.48) leads to an important conclusion in thermodynamics. Con- sider an infinitesimal process of a system at absolute temperature T. If the process is reversible, the differential change in entropy is δQ/T; if the process is irreversible, the change in entropy is greater than δQ/T. We thus conclude that the effect of irreversibility (e.g., friction) is to increase the entropy of a system. Alternatively, observe that the entropy production σ in Eq. (5.49), for any real process, must be positive.
Finally, consider an isolated system, a system that exchanges no work or heat with its surroundings. For such a system the first law demands that E2 = E1 for any process. Equation (5.48) takes the form
ΔS ≥ 0 (5.50)
demanding that the entropy of an isolated system either remains constant or
increases, depending on whether the process is reversible or irreversible. Hence, for any real process the entropy of an isolated system must increase. Alternatively, we can state that the entropy production associated with a real process of an isolated
system must be positive since for an isolated system ΔS = σ .
We can restate Eq. (5.50) by considering an isolated system to be composed of
any system under consideration plus the surroundings to that system as a second larger system. The heat and work transferred to the system under consideration are negative the heat and work transferred from the surroundings. This combination of the system and surroundings is often referred to as the universe. For the universe we can write
where σ = 0 applies to a reversible (ideal) process and the inequality to an irreversible (real) process. Relation (5.51), the principle of entropy increase, is often used as the mathematical statement of the second law. In Eq. (5.51), σ has also been referred to as ΔSgen , the entropy generated.
EXAMPLE 5.11
Air is contained in one half of an insulated tank. The other half is completely evacuated. The membrane separating the two halves is punctured and the air quickly fills the entire volume. Calculate the specific entropy change of this iso- lated system.
Solution
The entire tank is chosen as the system boundary. No heat transfer occurs across the boundary and the air does no work. The first law then takes the form ΔU = mCv (T2 − T1 ) = 0. Hence, the final temperature is equal to the initial temperature. Using Eq. (5.25) for the entropy change, we have, with T 1=T2
Note that this satisfies Eq. (5.48) since for this example Q = 0, so that ∫δQ /T = 0 < mΔs.
EXAMPLE 5.12
Two kilograms of steam at 400°C and 600 kPa are cooled at constant pressure by transferring heat from a cylinder until the steam is completely condensed. The surroundings are at 25°C. Determine the net entropy production.
Solution
The entropy of the steam that defines our system decreases since heat is transferred from the system to the surroundings. From the steam tables this change is found to be
ΔSsys = m(s2 − s1 ) = 2(1.9316 − 7.708) = −11.55 kJ/K
The heat transfer to the surroundings occurs at constant temperature. Hence, the entropy change of the surroundings [see Eq. (5.18)] is
The heat transfer for the constant-pressure process needed to condense the steam is
The entropy production is positive, as it must be.
5.9 The Second Law Applied to a Control Volume
Return once again to a fixed control volume, a device, with one inlet and one outlet. At ime t the system occupies a small volume 1 (that enters the device from the inlet pipe over a time increment Δt) plus the device; then at t + Δt, the system occupies the device plus the small volume 2 that leaves the device. The second law for the steady-state device, can be stated by applying Eq. (5.48):
Recognizing that Sdevice (t + Δt) = Sdevice (t) due to the steady flow (all quantities are
independent of time), we have
where δQc.s. is the incremental heat transfer into the device at a boundary location where the temperature is T , the subscript “c.s.” representing the control surface c.s. surrounding the device. Introducing the entropy production of Eq. (5.49),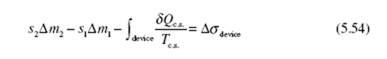 
Let’s assume that the entire control surface is at a constant temperature T c.s, a rather common assumption. If we divide Eq. (5.54) by Δt and use dots to denote rates, we arrive at the steady-state rate equation
Zero rate of entropy production is associated with a reversible process whereas positive entropy production is associated with the many irreversibilities that may exist within a control volume; these are due to viscous effects, separation of the
flow from turbine or compressor blades, and large temperature differences over which heat transfer takes place, to name a few.
By transferring energy to the control volume via heat transfer, we can obviously increase the entropy of the fluid flowing from the control volume so that s > s . If irreversibilities are present, σ·device will be positive and s2 will be greater than the exiting entropy for the same rate of heat transfer without irreversibilities. We also note that for an adiabatic steady-flow process, the entropy also increases from inlet to exit due to irreversibilities since, for that case, Eq. (5.56) shows that
s2 ≥ s1 (5.56)
since σ·device must be positive or zero. For the reversible adiabatic process the inlet entropy and exit entropy are equal since σ·device = 0; this is the isentropic process. We use this fact when studying a reversible adiabatic process involving, for example, steam or a refrigerant flowing through an ideal turbine or compressor.
We have calculated the efficiency of a cycle earlier in this chapter. Now we desire a quantity that can be used as a measure of the irreversibilities that exist in a particular device. The efficiency of a device is one such measure; it is defined as the ratio of the actual performance of a device to the ideal performance. The ideal performance is often that associated with an isentropic process. For example, the efficiency of a turbine would be
where wa is the actual shaft work output and ws is the maximum shaft work output, i.e., the work associated with an isentropic process. In general, the efficiency is defined using the optimum output; for a nozzle we would use the maximum kinetic energy increase. For a compressor the actual work required is greater than the min- imum work requirement of an isentropic process. For a compressor or pump the efficiency is defined to be 
Each efficiency above is often called the adiabatic efficiency since each efficiency is based on an adiabatic process.
EXAMPLE 5.13
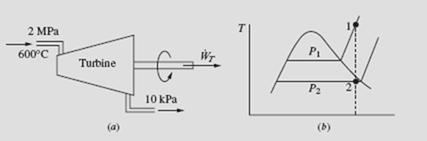
Superheated steam enters a turbine, as shown, and exits at 10 kPa. For a mass flow rate of 2 kg/s, determine the maximum power output.
Solution
If we neglect kinetic energy and potential energy changes, the first law, for an
adiabatic process, is −WT = m(h2 – h1). Since we desire the maximum power output, the process is assumed to be reversible, the entropy exiting is the same as the entropy entering, as shown (such a sketch is quite useful in visualizing the process). From the steam tables, at 600°C and 2 MPa,
With the above value for s we see that state 2 is in the quality region. The quality is determined as follows:
EXAMPLE 5.14
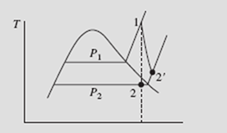
The turbine of Example 5.13 is assumed to be 80 percent efficient. Determine the entropy and temperature of the final state. Sketch the real process on a T–s diagram.
Solution
Using the definition of efficiency, the actual power output is
Using this value and P2′ = 10 kPa, we see that state 2′ lies in the superheated region, since h2′ > hg. This is shown schematically below. At P2 = 10 kPa and h2′ = 2770, we interpolate to find the value of T2′ :
The entropy is interpolated to be s2′ = 8.46 kJ/kg ⋅ K.
Note that the irreversibility has the desired effect of moving state 2 into the super- heated region, thereby eliminating the formation of droplets due to the condensation of moisture. In an actual turbine, moisture formation cannot be tolerated because of damage to the turbine blades, so the irreversibility is somewhat desirable.
EXAMPLE 5.15
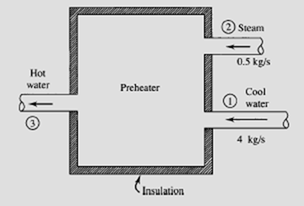
A preheater is used to preheat water in a power plant cycle, as shown. The super- heated steam is at a temperature of 250°C and the entering water is at 45°C. All pressures are 600 kPa. Calculate the rate of entropy production.
Solution
The conservation of mass requires that
m3= m2 + m· 1 = 0.5 + 4 = 4.5 kg/s
The first law allows us to calculate the temperature of the exiting water. Neglecting kinetic energy and potential energy changes and assuming zero heat transfer, the first law takes the form m· 3h3 = m· 2h2 + m· 1h1. Using the steam tables (h is the enthalpy of saturated liquid at 45°C),
This enthalpy is less than that of saturated liquid at 600 kPa. Thus, the exiting water is subcooled. Its temperature is interpolated from the saturated steam tables (find T that gives h = 496 kJ/kg) to be
The entropy at this temperature is then interpolated (using sf) to be s3 = 1.508 kJ/kg · K. The entropy of the entering superheated steam is found to be s2 = 7.182 kJ/kg · K. The entering entropy of the water is sf at T1 = 45°C, or s1 = 0.639 kJ/kg · K. Finally, modifying Eq. (5.56), to account for two inlets, we have, with no heat transfer,
This is positive, indicating that entropy is produced, a consequence of the second law. The mixing process between the superheated steam and the sub- cooled water is indeed an irreversible process.
Quiz No. 1
1. An inventor claims a thermal engine operates between ocean layers at 27 and
10°C. It produces 10 kW and discharges 9900 kJ/min. Such an engine is
(A) Impossible
(B) Reversible
(C) Possible
(D) Probable
2. A heat pump is to provide 2000 kJ/h to a house maintained at 20°C. If it is
−20°C outside, what is the minimum power requirement?
(A) 385 kJ/h
(B) 316 kJ/h
(C) 273 kJ/h
(D) 184 kJ/h
3. Select an acceptable paraphrase of the Kelvin-Planck statement of the second law.
(A) No process can produce more work than the heat that it accepts.
(B) No engine can produce more work than the heat that it intakes.
(C) An engine cannot produce work without accepting heat.
(D) An engine has to reject heat.
4. A power plant burns 1000 kg of coal each hour and produces 500 kW of power. Calculate the overall thermal efficiency if each kg of coal produces 6 MJ of energy.
(A) 0.35
(B) 0.30
(C) 0.25
(D) 0.20
5. Two Carnot engines operate in series between two reservoirs maintained at 327 and 27°C, respectively. The energy rejected by the first engine is input into the second engine. If the first engine’s efficiency is 20 percent greater than the second engine’s efficiency, calculate the intermediate temperature.
(A) 106°C
(B) 136°C
(C) 243°C
(D) 408°C
6. A heat pump is to maintain a house at 20°C when the outside air is at
−25°C. It is determined that 1800 kJ is required each minute to accomplish
this. Calculate the minimum power required.
(A) 3.87 kW
(B) 4.23 kW
(C) 4.61 kW
(D) 3.99 kW
7. Which of the following entropy relationships is incorrect?
(A) Air, V = const: Δs = Cv lnT2 /T1
(B) Water: Δs = Cp lnT2 /T1
(C) Reservoir: Δs = Cp lnT2 /T1
(D) Copper: Δs = Cp lnT2 /T1
8. Two kilograms of air are heated at constant pressure of 200 kPa to 500°C.
Calculate the entropy change if the initial volume is 0.8 m3.
(A) 2.04 kJ/K
(B) 2.65 kJ/K
(C) 3.12 kJ/K
(D) 4.04 kJ/K
9. Two kilograms of air are compressed from 120 kPa and 27°C to 600 kPa in a rigid container. Calculate the entropy change.
(A) 5.04 kJ/K
(B) 4.65 kJ/K
(C) 3.12 kJ/K
(D) 2.31 kJ/K
10. A paddle wheel provides 200 kJ of work to the air contained in a 0.2-m3 rigid volume, initially at 400 kPa and 40°C. Determine the entropy change if the volume is insulated.
(A) 0.504 kJ/K
(B) 0.443 kJ/K
(C) 0.312 kJ/K
(D) 0.231 kJ/K
11. 0.2 kg of air is compressed slowly from 150 kPa and 40°C to 600 kPa, in an adiabatic process. Determine the final volume.
(A) 0.0445 m3
(B) 0.0662 m3
(C) 0.0845 m3
(D) 0.0943 m3
12. A rigid, insulated 4-m3 volume is divided in half by a membrane. One chamber is pressurized with air to 100 kPa and the other is completely evacuated. The membrane is ruptured and after a period of time equilibrium is restored. The entropy change of the air is nearest
(A) 0.624 kJ/K
(B) 0.573 kJ/K
(C) 0.473 kJ/K
(D) 0.351 kJ/K
13. Ten kilograms of air are expanded isentropically from 500°C and 6 MPa to
400 kPa. The work accomplished is nearest
(A) 7400 kJ
(B) 6200 kJ
(C) 4300 kJ
(D) 2990 kJ
14. Find the work needed to isentropically compress 2 kg of air in a cylinder at
400 kPa and 400°C to 2 MPa.
(A) 1020 kJ
(B) 941 kJ
(C) 787 kJ
(D) 563 kJ
15. Calculate the total entropy change if 10 kg of ice at 0°C are mixed in an insulated container with 20 kg of water at 20°C. Heat of melting for ice is
340 kJ/kg.
(A) 6.19 kJ/K
(B) 3.95 kJ/K
(C) 1.26 kJ/K
(D) 0.214 kJ/K
16. A 5-kg block of copper at 100°C is submerged in 10 kg of water at 10°C, and after a period of time, equilibrium is established. If the container is insulated, calculate the entropy change of the universe.
(A) 0.082 kJ/K
(B) 0.095 kJ/K
(C) 0.108 kJ/K
(D) 0.116 kJ/K
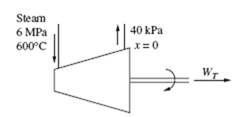
17. Find wT of the insulated turbine shown.
(A) 910 kJ/kg
(B) 1020 kJ/kg
(C) 1200 kJ/kg
(D) 1430 kJ/kg
18. The efficiency of the turbine of Prob. 17 is nearest
(A) 92%
(B) 89%
(C) 85%
(D) 81%
19. A nozzle accelerates steam at 120 kPa and 200°C from 20 m/s to the atmosphere. If it is 85 percent efficient, the exiting velocity is nearest
(A) 290 m/s
(B) 230 m/s
(C) 200 m/s
(D) 185 m/s
20. A turbine produces 4 MW by extracting energy from 4 kg of steam which flows through the turbine every second. The steam enters at 600°C and 1600 kPa and exits at 10 kPa. The turbine efficiency is nearest
(A) 82%
(B) 85%
(C) 87%
(D) 91%
Quiz No. 2
1. An engine operates on 100°C geothermal water. It exhausts to a 20°C stream. Its maximum efficiency is nearest
(A) 21%
(B) 32%
(C) 58%
(D) 80%
2. Which of the following can be assumed to be reversible?
(A) A paddle wheel
(B) A burst membrane
(C) A resistance heater
(D) A piston compressing gas in a race engine
3. A Carnot engine operates between reservoirs at 20 and 200°C. If 10 kW of
power is produced, find the rejected heat rate.
(A) 26.3 kJ/s
(B) 20.2 kJ/s
(C) 16.3 kJ/s
(D) 12.0 kJ/s
4. An automobile that has a gas mileage of 13 km/L is traveling at 100 km/h.
At this speed essentially all the power produced by the engine is used to overcome air drag. If the air drag force is given by 1 ρV 2 AC
determine the thermal efficiency of the engine at this speed using projected area A = 3 m2, drag coefficient C
= 0.28, and heating value of gasoline 9000 kJ/kg. Gasoline
has a density of 740 kg/m3.
|
(A) |
0.431 |
|
(B) |
0.519 |
|
(C) |
0.587 |
|
(D) |
0.652 |
5. A proposed power cycle is designed to operate between temperature
reservoirs of 900 and 20°C. It is supposed to produce 43 hp from the
2500 kJ of energy extracted each minute. Is the proposal feasible?
(A) No
(B) Yes
(C) Maybe
(D) Insufficient information
6. A Carnot refrigeration cycle is used to estimate the energy requirement in an attempt to reduce the temperature of a specimen to absolute zero.
Suppose that we wish to remove 0.01 J of energy from the specimen when
it is at 2 × 10−6 K. How much work is necessary if the high-temperature
reservoir is at 20°C?
(A) 622 kJ
(B) 864 kJ
(C) 1170 kJ
(D) 1465 kJ
7. One kilogram of air is heated in a rigid container from 20 to 300°C. The
entropy change is nearest
(A) 0.64 kJ/K
(B) 0.54 kJ/K
(C) 0.48 kJ/K
(D) 0.34 kJ/K
8. Which of the following second law statements is incorrect?
(A) The entropy of an isolated system must remain constant or increase.
(B) The entropy of a hot copper block decreases as it cools.
(C) If ice is melted in water in an insulated container, the net entropy decreases.
(D) Work must be input if energy is transferred from a cold body to a hot body.
9. A piston allows air to expand from 6 MPa to 200 kPa. The initial volume and temperature are 500 cm3 and 800°C. If the temperature is held constant, calculate the entropy change.
(A) 10.9 kJ/K
(B) 9.51 kJ/K
(C) 8.57 kJ/K
(D) 7.41 kJ/K
10. The entropy change in a certain expansion process is 5.2 kJ/K. The nitrogen, initially at 80 kPa, 27°C, and 4 m3, achieves a final temperature of 127°C. Calculate the final volume.
(A) 255 m3
(B) 223 m3
(C) 158 m3
(D) 126 m3
11. A piston is inserted into a cylinder causing the pressure in the air to change from 50 to 4000 kPa while the temperature remains constant at 27°C. To accomplish this, heat transfer must occur. Determine the entropy change.
(A) −0.92 kJ/kg ⋅ K
(B) −0.98 kJ/kg ⋅ K
(C) −1.08 kJ/kg ⋅ K
(D) −1.26 kJ/kg ⋅ K
12. A torque of 40 N · m is needed to rotate a shaft at 40 rad/s. It is attached to a paddle wheel located in a rigid 2-m3 volume. Initially the temperature is 47°C and the pressure is 200 kPa; if the paddle wheel rotates for 10 min and 500 kJ of heat is transferred to the air in the volume, determine the entropy increase assuming constant specific heats.
(A) 3.21 kJ/K
(B) 2.81 kJ/K
(C) 2.59 kJ/K
(D) 2.04 kJ/K
13. Four kilograms of air expand in an insulated cylinder from 500 kPa and 227°C to 20 kPa. What is the work output?
(A) 863 kJ
(B) 892 kJ
(C) 964 kJ
(D) 1250 kJ
14. Steam, at a quality of 85 percent, is expanded in a cylinder at a constant pressure of 800 kPa by adding 2000 kJ/kg of heat. Compute the entropy increase.
(A) 3.99 kJ/ kg ⋅ K
(B) 3.74 kJ/ kg ⋅ K
(C) 3.22 kJ/ kg ⋅ K
(D) 2.91 kJ/ kg ⋅ K
15. Two kilograms of saturated steam at 100°C are contained in a cylinder. If the
steam undergoes an isentropic expansion to 20 kPa, determine the work output.
(A) 376 kJ
(B) 447 kJ
(C) 564 kJ
(D) 666 kJ
16. Ten kilograms of iron at 300°C are chilled in a large volume of ice and
water. Find the total entropy change.
(A) 0.88 kJ/K
(B) 1.01 kJ/K
(C) 1.26 kJ/K
(D) 1.61 kJ/K
17. Five kilograms of ice at −20°C are mixed with 10 kg of water initially at
20°C. If there is no significant heat transfer from the container, determine
the net entropy change. It takes 330 kJ to melt a kg of ice.
(A) 0.064 kJ/K
(B) 0.084 kJ/K
(C) 1.04 kJ/K
(D) 1.24 kJ/K
18. Two kilograms of air are stored in a rigid volume of 2 m3 with the temperature initially at 300°C. Heat is transferred from the air until the pressure reaches 120 kPa. Calculate the entropy change of the air.
(A) −0.292 kJ/K
(B) −0.357 kJ/K
(C) −0.452 kJ/K
(D) −0.498 kJ/K
19. Calculate the entropy change of the universe for Prob. 18 if the surroundings are at 27°C.
(A) 0.252 kJ/K
(B) 0.289 kJ/K
(C) 0.328 kJ/K
(D) 0.371 kJ/K
20. Two hundred kilowatts are to be produced by a steam turbine. The outlet steam is to be saturated at 80 kPa and the steam entering will be at 600°C. For an isentropic process, determine the mass flow rate of steam.
(A) 0.342 kg/s
(B) 0.287 kg/s
(C) 0.198 kg/s
(D) 0.116 kg/s