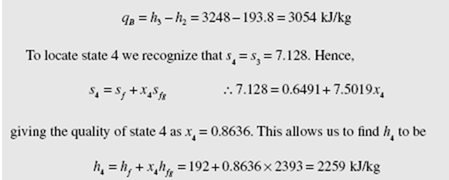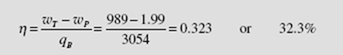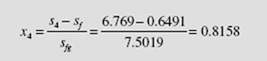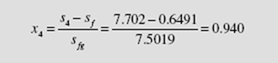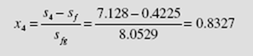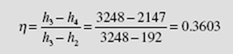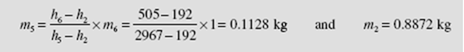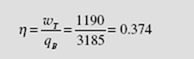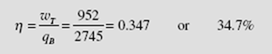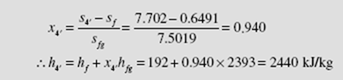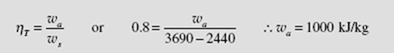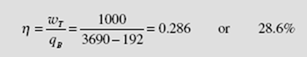Power and Refrigeration Vapor Cycles
The ideal Carnot cycle is used as a model against which all real and all other ideal cycles are compared. The efficiency of a Carnot power cycle is the maximum possible for any power cycle. We observed that the Carnot-cycle efficiency is increased by raising the temperature at which heat is added or by lowering the temperature at which heat is rejected. We will observe that this carries over to real cycles: using the highest maximum temperature and the lowest minimum temperature maximizes cycle efficiency.
We will first discuss vapor cycles that are used to generate power, then vapor cycles that are used to refrigerate or heat a space. In the next chapter we will examine both power and refrigeration cycles that use air as the working fluid.
6.1 The Rankine Cycle
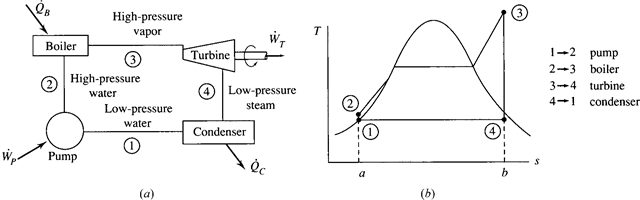
The electric power generating industry uses power cycles that operate in such a way that the working fluid changes phase from a liquid to a vapor. The simplest vapor power cycle is called the Rankine cycle, shown schematically in Fig. 6.1a. A major feature of such a cycle is that the pump requires very little work to deliver high- pressure water to the boiler. A possible disadvantage is that the expansion process in the turbine usually enters the quality region, resulting in the formation of liquid droplets that may damage the turbine blades.
The Rankine cycle is an idealized cycle in which losses in each of the four com- ponents are neglected. The Rankine cycle is composed of the four ideal processes shown on the T-s diagram in Fig. 6.1b:
1 → 2: Isentropic compression in a pump
2 → 3: Constant-pressure heat addition in a boiler
3 → 4: Isentropic expansion in a turbine
4 → 1: Constant-pressure heat rejection in a condenser
The pump is used to increase the pressure of the saturated liquid. Actually, states 1
and 2 are essentially the same since the high-pressure lines are extremely close to the saturation curve; they are shown separated for illustration only. The boiler (also called a steam generator) and the condenser are heat exchangers that neither require nor produce any work. An energy balance was applied to similar devices of the Rankine cycle in Sec. 4.8.
If we neglect kinetic energy and potential energy changes, the net work output is the area under the T–s diagram, represented by area 1-2-3-4-1 of Fig. 6.1b; this
Figure 6.1 The Rankine cycle. (a) The major components. (b) The T–s diagram.
is true since the first law requires that Wnet= Q net. The heat transfer to the water ispresented by area a-2-3-b-a. Thus, the thermal efficiency η of the Rankine cycle is
that is, the desired output divided by the energy input (the purchased energy). Obviously, the thermal efficiency can be improved by increasing the numerator or by decreasing the denominator. This can be done by increasing the pump outlet pres- sure P2, increasing the boiler outlet temperature T3, or decreasing the turbine outlet pressure P .
Note that the efficiency of the Rankine cycle is less than that of a Carnot cycle operating between the high temperature T and the low temperature T since most of the heat transfer from a high-temperature reservoir (the boiler) occurs across large temperature differences, a situation that occurs in most heat exchangers (recall that heat transfer across a finite temperature difference is irreversible; i.e., a source of loss).
Let us now find expressions for the work and the heat transfer of the four devices of Fig. 6.1a. Section 4.8 provides the following expressions for the pump, boiler, turbine, and condenser:
where wP and qC are expressed as positive quantities. In terms of the above, the thermal efficiency is
The pump work is usually quite small compared to the turbine work and can most often be neglected. With this approximation there results
This is the relation most often used for the thermal efficiency of the Rankine cycle.
EXAMPLE 6.1
A steam power plant is proposed to operate between the pressures of 10 kPa and 2 MPa with a maximum temperature of 400°C, as shown. Determine the maximum efficiency possible from the power cycle.
Solution
Let us include the pump work in the calculation and show that it is negligible. Also, we will assume a unit mass of working fluid since we are only interested in the efficiency. The pump work is [see Eq. (4.56)]
From the steam tables we find the values listed above. Using Eq. (4.52) we find that
The work output from the turbine is
wT = h3 − h4 = 3248 − 2259 = 989 kJ/kg
Consequently, the efficiency is
Obviously, the work required in the pumping process is negligible, being only
0.2 percent of the turbine work. In engineering applications we often neglect quantities that have an influence of less than 3 percent, since invariably there is
some quantity in the calculations that is known to only ±3 percent, for example,
the mass flow rate, the dimensions of a pipe, or the density of the fluid.
6.2 Rankine Cycle Efficiency
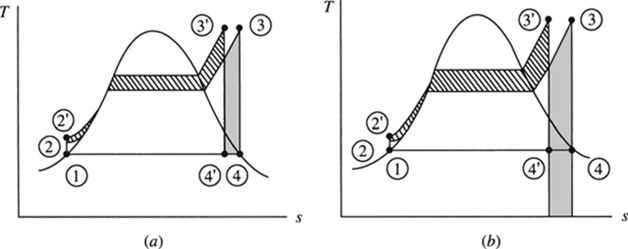
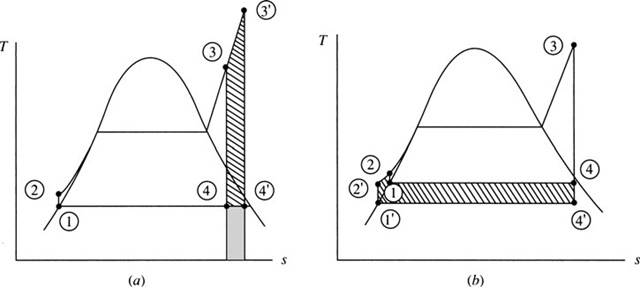
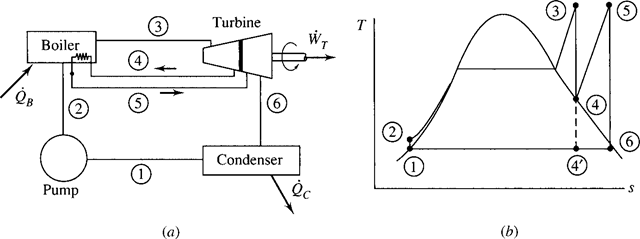
The efficiency of the Rankine cycle can be improved by increasing the boiler pres- sure while maintaining the maximum temperature and the minimum pressure. The net increase in work output is the crosshatched area minus the shaded area of Fig. 6.2a; the added heat decreases by the shaded area minus the crosshatched area of Fig. 6.2b. This leads to a significant increase in efficiency, as Example 6.2 will illustrate. The disadvantage of raising the boiler pressure is that the quality of the steam exiting the turbine may become too low (less than 95 percent), resulting in
Figure 6.2 Effect of increased pressure on the Rankine cycle.
Figure 6.3 Effect of (a) increased maximum temperature and
(b) decreased condenser pressure.
severe water droplet damage to the turbine blades and impaired turbine efficiency. However, this can be overcome, as we shall see.
Increasing the maximum temperature also results in an improvement in ther- mal efficiency of the Rankine cycle. In Fig. 6.3a the net work is increased by the crosshatched area and the heat input is increased by the sum of the crosshatched area and the shaded area, a smaller percentage increase than the work increase. Since the numerator of Eq. (6.4) realizes a larger percentage increase than the denominator, there will be a resulting increase in efficiency. This will be illustrated in Example 6.3. Another advantage of raising the boiler temperature is that the quality of state 4 is obviously increased, thereby reducing water droplet formation in the turbine.
A decrease in condenser pressure, illustrated in Fig. 6.3b, will also result in increased Rankine cycle efficiency. The net work will increase a significant amount, represented by the crosshatched area, and the heat input will increase a slight amount; the net result will be an increase in the Rankine cycle efficiency, as demonstrated in Example 6.4.
EXAMPLE 6.2
Increase the boiler pressure of Example 6.1 to 4 MPa while maintaining the maximum temperature and the minimum pressure. Neglect the work of the pump and calculate the percentage increase in the thermal efficiency.
Solution
The enthalpy his essentially equal to h≅ h2= 192 kJ/kg. At 400°C and 4 MPa
the enthalpy and entropy are h= 3214 kJ/kg and s3= 6.769 kJ/kg · K. State 4 is in the quality region. Using s= s3, the quality is found to be
Observe that the moisture content has increased to 18.4 (x4 = 81.6%) percent, an undesirable result. The enthalpy of state 4 is then
EXAMPLE 6.3
Increase the maximum temperature in the cycle of Example 6.1 to 600°C, while maintaining the boiler pressure and condenser pressure, and determine the per- centage increase in thermal efficiency.
Solution
At 600°C and 2 MPa the enthalpy and entropy are h = 3690 kJ/kg and s3 = 7.702 kJ/ kg·K. State 4 remains in the quality region and, using s4 = s3, we have
Note here that the moisture content has been decreased to 6.0 percent, a desirable result. The enthalpy of state 4 is now found to be h = 192 + (0.940)(2393) = 2441 kJ/kg. This allows us to calculate the thermal efficiency as
where h is taken from Example 6.1. The percentage increase is
In addition to a significant increase in efficiency, the quality of the steam exiting the turbine is 94 percent, a much-improved value.
EXAMPLE 6.4
Decrease the condenser pressure of Example 6.1 to 4 kPa while maintaining the boiler pressure and maximum temperature, and determine the percentage increase in thermal efficiency.
Solution
The enthalpies h2 = 192 kJ/kg and h3 = 3248 kJ/kg remain as stated in Example 6.1. Using s = s4= 7.128, with P4= 4 kPa, we find the quality to be
Note that the moisture content of 16.7 percent has increased from that of Example 6.1, as expected. The enthalpy of state 4 is h = 121 + (0.8327)(2433) = 2147 kJ/kg. The thermal efficiency is then
The percentage increase is found to be
This is the largest percentage increase which shows why the lowest possible condenser pressure is used.
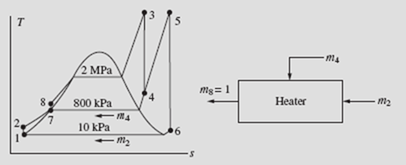
6.3 The Reheat Cycle
It is apparent from the previous section that when operating a Rankine cycle with a high boiler pressure or a low condenser pressure it is difficult to prevent liquid droplets from forming in the low-pressure portion of the turbine. Since most metals cannot withstand temperatures above about 600°C, the reheat cycle is often used to prevent liquid droplet formation: the steam passing through the turbine is reheated at some intermediate pressure, thereby raising the temperature to state 5 in the T-s diagram of Fig. 6.4. The steam then passes through the low-pressure section of the turbine and enters the condenser at state 6. This controls or completely eliminates the moisture problem in the turbine. The reheat cycle does not significantly influence the thermal efficiency of the cycle, but it does result in a significant additional work output.
Figure 6.4 The reheat cycle.
EXAMPLE 6.5
High-pressure steam enters a turbine at 2 MPa and 400°C. It is reheated at a pressure of 800 kPa to 400°C and then expanded to 10 kPa. Determine the cycle efficiency.
Solution
At 10 kPa saturated water has an enthalpy of h1 = h2 = 192 kJ/kg. From Table C.3 we find = 3248 kJ/kg and s3 = 7.128 kJ/kg ⋅ K. Setting s4 = s3 we interpolate,obtaining
At 800 kPa and 400°C we have
In the quality region use s6 = s5 and find
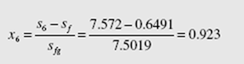Thus, h = 192 + (0.923)(2393) = 2401 kJ/kg. The energy input and output are
The thermal efficiency is then calculated to be
Note: The fact that state 6 is in the mixture region is not of concern since x is
quite close to the saturated vapor state. As we will see in Sec. 6.7, turbine losses are able to move state 6 into the superheat region.
6.4 The Regenerative Cycle
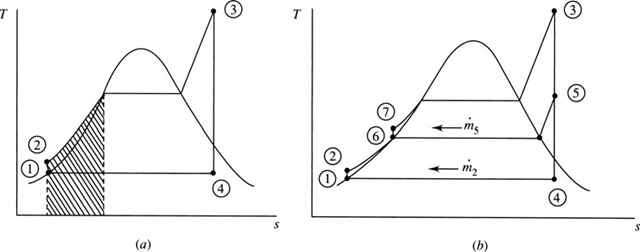
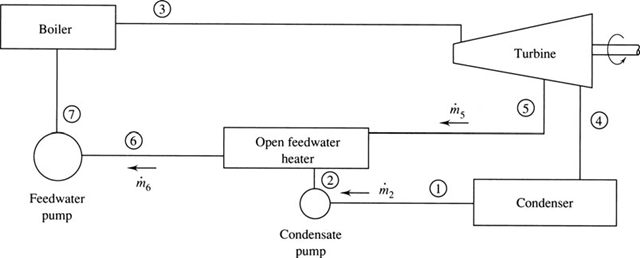
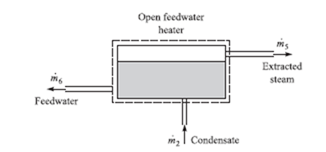
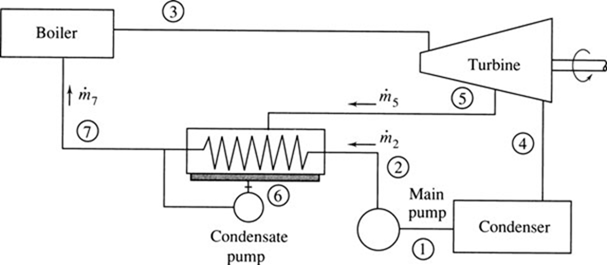
In the conventional Rankine cycle, as well as in the reheat cycle, a considerable percentage of the total energy input is used to heat the high-pressure water from T to its saturation temperature. The crosshatched area in Fig. 6.5a represents this necessary energy. To reduce this energy, the water is preheated before it enters the boiler by intercepting some of the steam as it expands in the turbine (for example, at state 5 of Fig. 6.5b) and mixing it with the water as it exits the first of the pumps, thereby preheating the water from T2 to T6. This would avoid the necessity of condensing all the steam, thereby reducing the amount of energy lost from the con- denser. A cycle that utilizes this type of heating is a regenerative cycle, and the process is referred to as regeneration. A schematic representation of the major elements of such a cycle is shown in Fig. 6.6. The water entering the boiler is often referred to as feedwater, and the device used to mix the extracted steam and the condenser water is called a feedwater heater. When the condensate is mixed directly with the steam, it is done so in an open feedwater heater, as sketched in Fig. 6.6.
When analyzing a regenerative cycle we must consider a control volume surrounding the feedwater heater; see Fig. 6.7. A mass balance would result in
m· 6 = m· 5 + m· 2 (6.5)
An energy balance, assuming an insulated heater, neglecting kinetic and poten-
tial energy changes, gives
Figure 6.5 The regenerative cycle.
Figure 6.6 The major elements of the regenerative cycle.
Combining the above two equations gives the mass flux of intercepted steam:
A closed feedwater heater that can be designed into a system using only one main pump is also a possibility, as sketched in Fig. 6.8. The closed feedwater heater is a heat exchanger in which the water passes through in tubes and the steam surrounds the tubes, condensing on the outer surfaces. The condensate thus formed, at temperature T , is pumped with a small condensate pump into the main feedwater line, as shown. Mass and energy balances are also required when analyzing a closed feedwater heater; if pump energy requirement is neglected in the analysis, the same relationship [see Eq. (6.7)] results.
The pressure at which the steam should be extracted from the turbine is approximated as follows. For one heater the steam should be extracted at the point that
Figure 6.7 An open feedwater heater.
Figure 6.8 A closed feedwater heater.
allows the exiting feedwater temperature T to be midway between the saturated steam temperature in the boiler and the condenser temperature. For several heaters the temperature difference should be divided as equally as possible. It is not uncommon to combine a reheat cycle and a regenerative cycle, thereby avoiding the moisture problem and increasing the thermal efficiency. Ideal efficiencies significantly higher than for nonregenerative cycles can be realized with such a combination cycle.
There is an additional technique to effectively increase the “efficiency” of a power plant. There are special situations where a power plant can be located strategically so that the rejected steam from the condenser can be utilized to heat or cool (absorption refrigeration) buildings or it can be used in various industrial processes. This is often referred to as cogeneration. Often one-half of the rejected heat can be effectively used, almost doubling the “efficiency” of a power plant. The power plant must be located close to an industrial site or a densely populated area. A college campus is an obvious candidate for cogeneration, as are most large industrial plants. Several major cities are using rejected heat in downtown areas for cooling as well as heating.
EXAMPLE 6.6
The high-temperature cycle of Example 6.3 is to be modified by inserting an open feedwater heater with an extraction pressure of 200 kPa. Determine the percentage increase in thermal efficiency.
Solution
We have from Example 6.3 and the steam tables
Now, locate state 5. Using s5 = s3 = 7.702 kJ/kg ⋅ K, we interpolate and find, at 200 kPa,
We now apply conservation of mass and the first law to a control volume surrounding the feedwater heater. For every kilogram of steam passing through the boiler, i.e., m m= 1 kg, we use Eq. (6.7) to find m5:
The work output from the turbine is
The energy input to the boiler is q= h3– h7= 3690 − 505 = 3185 kJ/kg. The thermal efficiency is calculated to be
The increase in efficiency is
EXAMPLE 6.7
The open feedwater heater shown is added to the reheat cycle of Example 6.5. Steam is extracted where the reheater interrupts the turbine flow. Determine the efficiency of this reheat-regeneration cycle.
Solution
From the steam tables or from Example 6.5,
Continuity, using m = 1 kg and the first law applied to the heater, gives
The turbine work output is then
The efficiency is calculated to be
Note the improvement of 7.4% in cycle efficiency over that of the basic Rankine cycle of Example 6.1.
6.5 Effect of Losses on Power Cycle Efficiency
The preceding sections dealt with ideal cycles assuming no pressure drop through the pipes in the boiler, no losses as the superheated steam passes over the blades in the turbine, no subcooling of the water leaving the condenser, and no pump losses during the compression process. The losses in the combustion process and the inefficiencies in the subsequent heat transfer to the fluid in the pipes of the boiler are not included here; those losses, which are in the neighborhood of 15 percent of the energy con- tained in the coal or oil, would be included in the overall plant efficiency.
There is actually only one substantial loss that must be accounted for when we calculate the actual cycle efficiency: the loss that occurs when the steam is expanded
through the rows of turbine blades in the turbine. As the steam passes over a turbine blade, there is friction on the blade and the steam may separate from the rear por- tion of the blade. These losses result in a turbine efficiency of 80 to 89 percent. Turbine efficiency is defined as.
where wa is the actual work and ws is the isentropic work. The definition of pump efficiency is
where the isentropic work input is obviously less than the actual input.
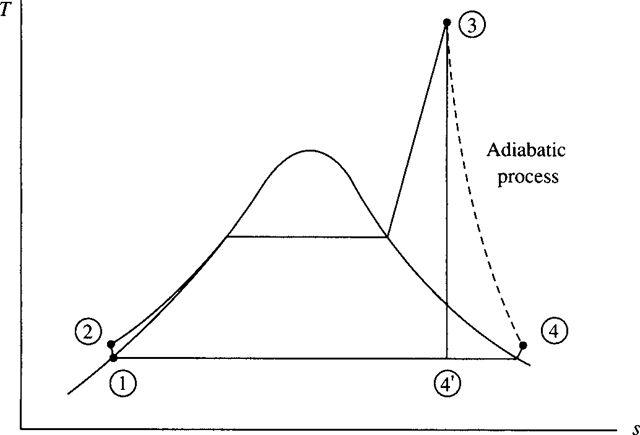
There is a substantial loss in pressure, probably 10 to 20 percent, as the fluid flows from the pump exit through the boiler to the turbine inlet. The loss can be overcome by simply increasing the exit pressure from the pump. This does require more pump work, but the pump work is still less than 1 percent of the turbine output and is thus negligible. Consequently, we ignore the boiler pipe losses. The condenser can be designed to operate such that the exiting water is very close to the saturated liquid condition. This will minimize the condenser losses so that they can also be neglected. The resulting actual Rankine cycle is shown on the T–s diagram in Fig. 6.9; the only significant loss is the turbine loss. Note the increase in entropy of state 4 as com- pared to state 3. Also, note the desirable effect of the decreased moisture content of state 4; in fact, state 4 may even move into the superheated region, as shown.
Figure 6.9 The Rankine cycle with turbine losses.
EXAMPLE 6.8
A Rankine cycle operates between pressures of 2 MPa and 10 kPa with a maxi- mum temperature of 600°C. If the insulated turbine has an efficiency of 80 per- cent, calculate the cycle efficiency and the temperature of the steam at the turbine outlet.
Solution
From the steam tables we find h1 = h2 = 192 kJ/kg, h3 = 3690 kJ/kg, and s3 = 7.702 kJ/kg ⋅ K. Setting s4′ = s3 we find the quality and enthalpy of state 4′ (see Fig. 6.9) to be
From the definition of turbine efficiency,
The cycle efficiency is then
Note the substantial reduction from the ideal cycle efficiency of 35.7 percent, as calculated in Example 6.3. The adiabatic process from state 3 to state 4 allows us to write
At 10 kPa we find state 4 in the superheated region. The temperature is interpolated in the superheat region at 10 kPa to be
Obviously, the moisture problem has been eliminated by the losses in the turbine; the losses tend to act as a small reheater.