Power and Refrigeration Gas Cycles
Several cycles utilize a gas as the working substance, the most common being the Otto cycle and the diesel cycle, used in internal combustion engines. The word “cycle” used in reference to an internal combustion engine is technically incorrect since the working fluid does not undergo a thermodynamic cycle; air enters the engine, mixes with a fuel, undergoes combustion, and exits the engine as exhaust gases. This is often referred to as an open cycle, but we should keep in mind that a thermodynamic cycle does not really occur; the engine itself operates in what we could call a mechanical cycle. We do, however, analyze an internal combustion engine as though the working fluid operated on a cycle; it is an approximation that
allows us to predict influences of engine design on such quantities as efficiency and fuel consumption. It also allows us to compare the features of the various cycles.
7.1 The Air-Standard Cycle
In this section we introduce engines that utilize a gas as the working fluid. Spark- ignition engines that burn gasoline and compression-ignition (diesel) engines that burn fuel oil are the two most common engines of this type.
The operation of a gas engine can be analyzed by assuming that the working fluid does indeed go through a complete thermodynamic cycle. The cycle is often called an air-standard cycle. All the air-standard cycles we will consider have cer- tain features in common:
• Air is the working fluid throughout the entire cycle. The mass of the small quantity of injected fuel is negligible.
• There is no inlet process or exhaust process.
• A heat transfer process replaces the combustion process with energy transferred from an external source.
• The exhaust process, used to restore the air to its original state, is replaced with a constant-volume process transferring heat to the surroundings so that no work is accomplished, as in an actual cycle.
• All processes are assumed to be in quasiequilibrium.
• The air is assumed to be an ideal gas with constant specific heats.
A number of the engines we will consider make use of a closed system with a piston-cylinder arrangement, as shown in Fig. 7.1. The cycle shown on the P-v and T-s diagrams in the figure is representative. The diameter of the piston is called the bore, and the distance the piston travels in one direction is the stroke. When the piston is at top dead center (TDC), the volume occupied by the air in the cylinder is at a minimum; this volume is the clearance volume. When the piston moves to bot- tom dead center (BDC), the air occupies the maximum volume. The difference between the maximum volume and the clearance volume is the displacement volume. The clearance volume is often implicitly presented as the percent clearance c, the ratio of the clearance volume to the displacement volume. The compression ratio r is defined to be the ratio of the volume in the cylinder with the piston at BDC to the clearance volume, that is, referring to Fig. 7.1,
Figure 7.1 The cycle of a piston-cylinder gasoline engine.
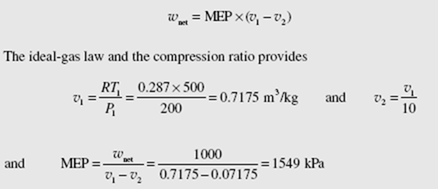
The mean effective pressure (MEP) is another quantity that is often used when rating piston-cylinder engines; it is the pressure that, if acting on the piston during the power stroke, would produce an amount of work equal to that actually done during the entire cycle. Thus,
In Fig. 7.1 this means that the enclosed area of the actual cycle is equal to the area under the MEP dotted line.
EXAMPLE 7.1
An engine operates with air on the cycle shown in Fig.7.1 with isentropic processes 1 → 2 and 3 → 4. If the compression ratio is 12, the minimum pressure is 200 kPa,
and the maximum pressure is 10 MPa, determine the percent clearance and the MEP, assuming constant specific heats.
Solution
The percent clearance is given by
But the compression ratio is r = V1 /V2 = 12. Thus,
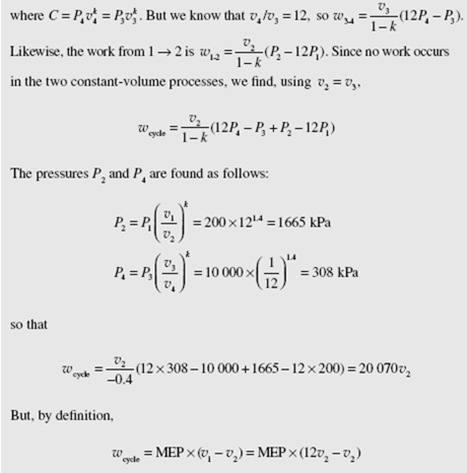
To determine the MEP we must calculate the area under the P-v diagram (the work), a rather difficult task. The work from 3 → 4 is, using Pvk = C,
Equating the two expressions yields
7.2 The Carnot Cycle
This ideal cycle was treated in detail in Chap. 5. Recall that the thermal efficiency of a Carnot engine,
![]() exceeds that of any real engine operating between the given temperatures. We will use this efficiency as an upper limit for all engines operating between T and T .
exceeds that of any real engine operating between the given temperatures. We will use this efficiency as an upper limit for all engines operating between T and T .
7.3 The Otto Cycle
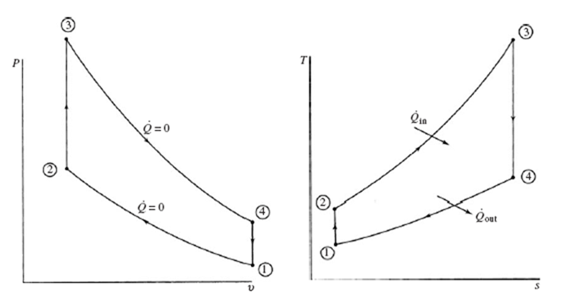
The four processes that form the Otto cycle are displayed in the T-s and P-v diagrams of Fig. 7.2. The piston starts at state 1 at BDC and compresses the air until it reaches TDC at state 2. Instantaneous combustion then occurs, due to spark ignition, resulting in a sudden jump in pressure to state 3 while the volume remains constant (a quasiequilibrium heat addition process). The process that follows is the power stroke as the air (the combustion products) expands isentropically to state 4. In the final process, heat transfer to the surroundings occurs and the cycle is completed.
The thermal efficiency of the Otto cycle is found from
Noting that the two heat transfer processes occur during constant-volume processes, for which the work is zero, there results
Figure 7.2 The Otto cycle.
where we have assumed each quantity to be positive. Then
 Thus, Eq. (7.7) gives the thermal efficiency as
Thus, Eq. (7.7) gives the thermal efficiency as
We see that the thermal efficiency in this Otto cycle is dependent only on the compression ratio r: higher the compression ratio, higher the thermal efficiency.
EXAMPLE 7.2
An Otto cycle is proposed to have a compression ratio of 10 while operating with a low air temperature of 227°C and a low pressure of 200 kPa. If the work output is to be 1000 kJ/kg, calculate the maximum possible thermal efficiency and com- pare with that of a Carnot cycle. Also, calculate the MEP. Assume constant specific heats.
Solution
The Otto cycle provides the model for this engine. The maximum possible ther- mal efficiency for the engine would be
The Otto cycle efficiency is less than that of a Carnot cycle because the heat transfer processes in the Otto cycle are highly irreversible.
The MEP is found by using the equation
7.4 The Diesel Cycle
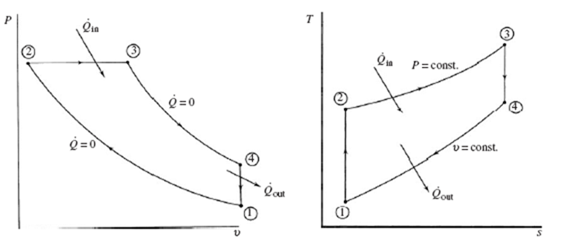
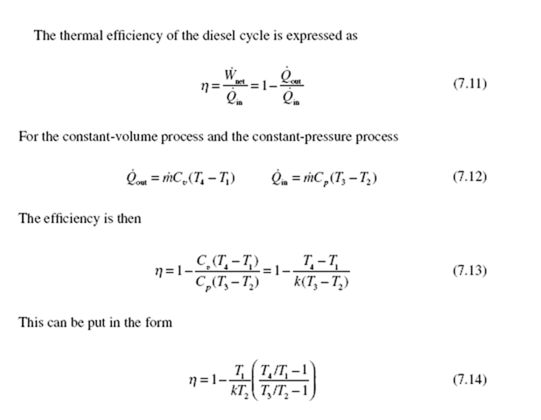
If the compression ratio is large enough, the temperature of the air in the cylinder when the piston approaches TDC will exceed the ignition temperature of diesel fuel. This will occur if the compression ratio is about 14 or greater. No external spark is needed; the diesel fuel is simply injected into the cylinder and combustion occurs because of the high temperature of the compressed air. This type of engine is referred to as a compression-ignition engine. The ideal cycle used to model the engine is the diesel cycle, shown in Fig. 7.3. The difference between this cycle and the Otto cycle is that, in the diesel cycle, the heat is added during a constant-pressure process.
The cycle begins with the piston at BDC, state 1; compression of the air occurs isentropically to state 2 at TDC; heat addition takes place (this models the injection and combustion of fuel) at constant pressure until state 3 is reached; expansion occurs isentropically to state 4 at BDC; constant-volume heat rejection completes the cycle and returns the air to the original state. Note that the power stroke includes the heat addition process and the expansion process.
This expression for the thermal efficiency is often written in terms of the compression ratio r and the cutoff ratio r which is the ratio of volumes from TDC to the
end of combustion. We then have
 From this expression we see that, for a given compression ratio r, the efficiency of the diesel cycle is less than that of an Otto cycle. For example, if r = 8 and rc = 2, the
From this expression we see that, for a given compression ratio r, the efficiency of the diesel cycle is less than that of an Otto cycle. For example, if r = 8 and rc = 2, the
Otto cycle efficiency is 56.3 percent and the diesel cycle efficiency is 49.0 percent.As r ncreases, the diesel cycle efficiency decreases. In practice, however, a compression ratio of 20 or so can be achieved in a diesel engine; using r = 20 and rc = 2, we would find η = 64.7 percent. Thus, because of the higher compression ratios, a diesel engine typically operates at a higher efficiency than a gasoline engine.
EXAMPLE 7.3
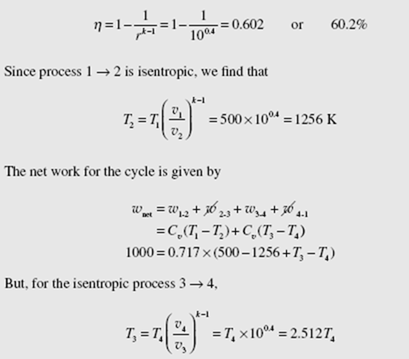
A diesel cycle, with a compression ratio of 18, operates on air with a low pressure of 200 kPa and a low temperature 200°C. If the high temperature is limited to 2000 K, determine the thermal efficiency and the MEP. Assume constant specific heats.
Solution
The cutoff ratio rc is found first. We have
The thermal efficiency is now calculated as
EXAMPLE 7.4
Rework Example 7.3 using Table E.1; do not assume constant specific heats. Calculate the thermal efficiency, the MEP, and the errors in both quantities as calculated in Example 7.3.
Solution
Equation (7.10) that gives the thermal efficiency of the Otto cycle was obtained assuming constant specific heats; so it cannot be used. We must calculate the work output and the heat added. Refering to Table E.1:
Hence, at state 4 we find from Table E.1 that T4 = 915 K and u4 = 687.5 kJ/kg. The first law results in
The errors are substantial primarily due to large temperature differences experienced in the diesel cycle. However, the constant specific heat analyses are of interest particularly in parametric studies where various designs are considered.



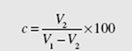
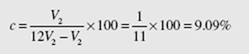
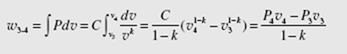

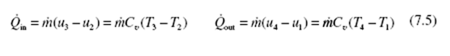
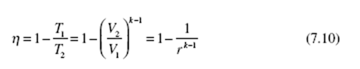

![image_thumb[1] image_thumb[1]](http://lh4.ggpht.com/-fxcdbqufgZ0/VFEz6v60oqI/AAAAAAAAtUA/NKJNwaEVIDY/image_thumb%25255B1%25255D_thumb.png?imgmax=800)
![image_thumb[2] image_thumb[2]](http://lh3.ggpht.com/-u2vyWvg9U-c/VFEz_G-RqgI/AAAAAAAAtUQ/d2bmzJ9DNi8/image_thumb%25255B2%25255D_thumb.png?imgmax=800)
![image_thumb[3] image_thumb[3]](http://lh4.ggpht.com/-QYR0KFR1ZdY/VFE0EQnRF9I/AAAAAAAAtUg/FGD8pCMiO1M/image_thumb%25255B3%25255D_thumb.png?imgmax=800)
![image_thumb[4] image_thumb[4]](http://lh4.ggpht.com/-y1yD0ibq4Cg/VFE0IIIi45I/AAAAAAAAtUw/52wxNZiKSeM/image_thumb%25255B4%25255D_thumb.png?imgmax=800)
![image_thumb[5] image_thumb[5]](http://lh4.ggpht.com/-i7TFIQVgcYI/VFE0NDWaGkI/AAAAAAAAtVA/Zpujkt3OWWI/image_thumb%25255B5%25255D_thumb.png?imgmax=800)