The First Law of Thermodynamics
The first law of thermodynamics is commonly called the law of conservation of energy. In previous courses, the study of conservation of energy may have emphasized changes in kinetic and potential energy and their relationship to work. A more general form of conservation of energy includes the effects of heat transfer and internal energy changes. This more general form is usually called the first law of thermodynamics. Other forms of energy could also be included, such as electro- static, magnetic, strain, and surface energy, but, in a first course in thermodynamics, problems involving these forms of energy are typically not included. We will present the first law for a system and then for a control volume.
4.1 The First Law Applied to a Cycle
We are now ready to present the first law of thermodynamics. A law is not derived or proved from basic principles but is simply a statement that we write based on our
observations. Historically, the first law of thermodynamics, simply called the first law, was stated for a cycle: the net heat transfer is equal to the net work done for a system undergoing a cycle. This is expressed in equation form by
∑W = ∑Q (4.1)
or, using the symbol to represent integration around a complete cycle,![]()
The first law can be illustrated by considering the following experiment. Let a weight be attached to a pulley-paddle-wheel setup, such as that shown in Fig. 4.1a. Let the weight fall a certain distance thereby doing work on the system, contained in the tank shown, equal to the weight multiplied by the distance dropped. The temperature of the system (the substance in the tank) will immediately rise an amount ΔT. Now, the system is returned to its initial state (the completion of the cycle) by transferring heat to the surroundings, as implied by the Q in Fig. 4.1b. This reduces the temperature of the system to its initial temperature. The first law states that this heat transfer will be exactly equal to the work that was done by the falling weight.
EXAMPLE 4.1
A linear spring, with spring constant K = 100 kN/m, is stretched a distance of 0.8 m and attached to a paddle wheel. The paddle wheel then rotates until the spring is unstretched. Calculate the heat transfer necessary to return the system to its initial state.
Figure 4.1 The first law applied to a cycle.
Solution
The work done by the linear spring on the system is given by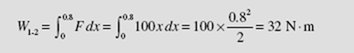
Since the heat transfer returns the system to its initial state, a cycle results.The first law then states that
4.2 The First Law Applied to a Process
The first law of thermodynamics is often applied to a process as the system changes from one state to another. Realizing that a cycle results when a system undergoes two or more processes and returns to the initial state, we could consider a cycle composed of the two processes represented by A and B in Fig. 4.2. Applying the first law to this cycle, Eq. (4.2) takes the form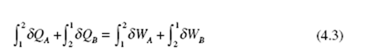
We interchange the limits on the process from 1 to 2 along B and write this as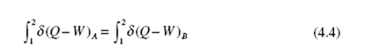
Figure 4.2 A cycle composed of two processes.
That is, the change in the quantity Q − W from state 1 to state 2 is the same along path A as along path B; since this change is independent of the path between states 1 and 2 we let
dQ − dW = dE (4.5)
where dE is an exact differential.
The quantity E is an extensive property of the system and represents the energy of the system at a particular state. Equation (4.5) can be integrated to yield
Q1-2 − W1-2 = E2 − E1 (4.6)
where Q1-2 state 2, W is the heat transferred to the system during the process from state 1 tois the work done by the system on the surroundings during the process,and E1-2 and E are the values of the property E. More often than not the subscripts will be dropped on Q and W when working problems. The property E represents all of the energy: kinetic energy KE, potential energy PE, and internal energy U, which includes chemical energy and the energy associated with the molecules and atoms. Any other form of energy is also included in the total energy E. Its associated intensive property is designated e.
The first law of thermodynamics then takes the form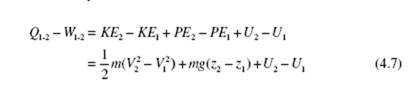
If we apply the first law to an isolated system, one which is not in contact with its
surroundings so that Q1-2 = W1-2= 0, the first law becomes the conservation of energy; that is,
E2 = E1 (4.8)
The internal energy U is an extensive property. Its associated intensive property is the specific internal energy u; that is, u = U/m. For simple systems in equilibrium, only two properties are necessary to establish the state of a pure substance, such as air or steam. Since internal energy is a property, it depends only on, say, pressure and temperature; or, for steam in the quality region, it depends on quality and temperature (or pressure). For steam, its value for a particular quality would be
u = u f + x(ug − u f ) (4.9)
We can now apply the first law to systems involving working fluids with tabulated property values. Before we apply the first law to systems involving substances such as ideal gases or solids, we will introduce several additional properties that will simplify that task in the next three articles, but first some examples.
EXAMPLE 4.2
A 5-hp fan is used in a large room to provide for air circulation. Assuming a well insulated, sealed room, determine the internal energy increase after 1 hour of operation.
Solution
By assumption, Q = 0. With ΔPE = ΔKE = 0 the first law becomes −W = ΔU. The work input is
W = (−5 hp )(1 hr)(746 W/hp )(3600 s/hr) = −1.343 × 107 J
where W = J/s.
The negative sign results because the work is input to the system. Finally, the internal energy increase is
ΔU = − (−1.343 × 107) = 1.343 × 107 J
EXAMPLE 4.3
A frictionless piston is used to provide a constant pressure of 400 kPa in a cylinder containing steam originally at 200°C with a volume of 2m3. Calculate the final temperature if 3500 kJ of heat is added.
Solution
The first law of thermodynamics, using ΔPE = ΔKE = 0, is Q − W = ΔU. The
work done during the motion of the piston is
W = ∫ PdV = P(V2 − V1 ) = 400(V2 − V1 )
The mass before and after remains unchanged. Using steam table C.3, this is expressed as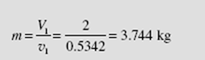
The volume V2 is written as V2= mv= 3.744 v . The first law is then, finding u1 from the steam tables in App. C,
3500 − 400(3.744v2 − 2) = (u2 − 2647) × 3.744
This requires a trial-and-error process. One plan for obtaining a solution is toguess a value for v2 and calculate u2 from the equation above. If this value checks with the u from the steam tables at the same temperature, then the guess is the correct one. For example, guess v2 = 1.0 m /kg. Then the equation gives u2 =3395 kJ/kg. From the steam tables, with P = 0.4 MPa, the u2 value allows us to nterpolate T= 653°C and the v2 gives T 600°C. Therefore, the guess must be revised. Try v2 = 1.06 m /kg. The equation gives u2 = 3372 kJ/kg. The tables are interpolated to give T = 640°C; for v , T = 647°C. The actual v is a little less than 1.06 m3/kg, with the final temperature being approximately
T2 = 644°C
4.3 Enthalpy
In the solution of problems involving systems, certain products or sums of proper- ties occur with regularity. One such combination of properties we define to be enthalpy H:
H = U + PV (4.10)
This property will come in handy, especially in a constant-pressure process, but also in other situations, as we shall see in examples and applications in future chapters. The specific enthalpy h is found by dividing by the mass: h = H /m. From Eq. (4.10), it is
h = u + Pv (4.11)
Enthalpy is a property of a system and is also found in the steam tables. The energy equation can now be written, for a constant-pressure equilibrium process, as
Q1–2 = H2 − H1 (4.12)
In a nonequilibrium constant-pressure process Δ H would not equal the heat transfer.
It is only the change in enthalpy or internal energy that is important; hence, we can arbitrarily choose the datum from which to measure h and u. We choose saturated liquid at 0°C to be the datum point for water; there h = 0 and u = 0.
EXAMPLE 4.4
Using the concept of enthalpy, solve the problem presented in Example 4.3.
Solution
The energy equation for a constant-pressure process is (with the subscript on the heat transfer omitted),
Q = H2 − H1 or 3500 = m(h2 − 2860)
Using steam table C.3, as in Example 4.3, the mass is
From the steam tables this interpolates to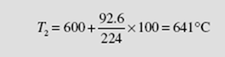
Obviously, enthalpy was very useful in solving this constant-pressure problem. Trial and error was unnecessary, and the solution was rather straightforward. We illustrated that the quantity we invented, enthalpy, is not necessary, but it is quite handy. We will use it often in calculations.
4.4 Latent Heat
The amount of energy that must be transferred in the form of heat to a substance held at constant pressure in order that a phase change occurs is called latent heat. It is the change in enthalpy of the substance at the saturated conditions of the two phases. The heat that is necessary to melt a unit mass of a substance at constant
pressure is the heat of fusion and is equal to h= h − h , where h is the enthalpy of saturated solid (see Table C.5 for ice) and h is the enthalpy of saturated liquid. The heat of vaporization is the heat required to completely vaporize a unit mass of saturated liquid; it is equal to h = h − h . When a solid changes phase directly to a gas, sublimation occurs; the heat of sublimation is equal to h = hg– hi. The heat of fusion and the heat of sublimation are relatively insensitive to pressure or temperature changes. For ice, the heat of fusion is approximately 330 kJ/kg and the heat of sublimation is about 2040 kJ/kg. The heat of vaporization of water is included in Tables C.1 and C.2 and is very sensitive to pressure and temperature.
4.5 Specific Heats
Since only two independent variables are necessary to establish the state of a simple system, the specific internal energy can be expressed as a function of temperature and specific volume; that is,
u = u(T , v) (4.13)
Using the chain rule from calculus, the differential can be stated as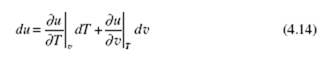
Joule’s classical experiment showed that u = u(T) for an ideal gas, so that ∂u /∂v We define the constant-volume specific heat C as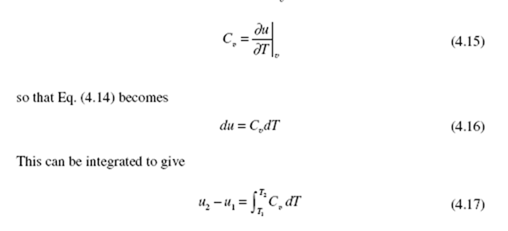
For a known C (T ), this can be integrated to find the change in internal energy over any temperature interval for an ideal gas.
Likewise, considering specific enthalpy to be dependent on the two variables T and P, we have
where we have used the ideal-gas equation of state. Since u is only a function of T, we see that h is also only a function of T for an ideal gas. Hence, ∂h/∂ P T = 0 and we have, from Eq. (4.18), for an ideal gas,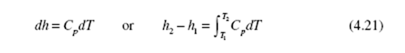
It is often convenient to specify specific heats on a per-mole, rather than a per-mass, basis; these molar specific heats are Cv and Cp . Clearly, we have the relations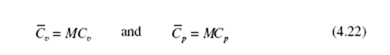
where M is the molar mass. Thus, Cv and Cp may be simply derived from the values of M and Cv and Cp listed in Table B.2. (The common “overbar notation” for a molar quantity is used throughout thermodynamics.)
The equation for enthalpy can be used to relate, for an ideal gas, the specific heats and the gas constant. In differential form Eq. (4.20) takes the form
dh = du + d(Pv) (4.23)
Introducing the specific heat relations and the ideal-gas equation, we have
Cp = Cv + R (4.24)
This relationship, or its molar equivalent, allows C to be determined from tabulated values or expressions for C . Note that the difference between C and C for an ideal gas is always a constant, even though both are functions of temperature.
The specific heat ratio k is also a property of particular interest; it is defined as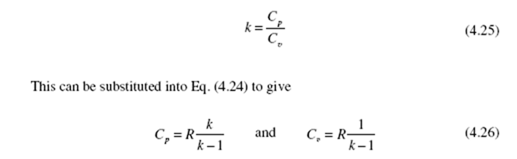
Obviously, since R is a constant for an ideal gas, the specific heat ratio k will depend only on the temperature.
For gases, the specific heats slowly increase with increasing temperature. Since they do not vary significantly over fairly large temperature differences, it is often acceptable to treat C and C as constants. For such situations there results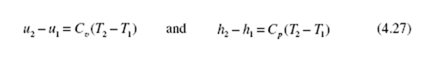
For air, we will use Cv = 0.717 kJ/kg ⋅ K and Cp = 1.00 kJ/kg ⋅ K, unless otherwise stated.1 For more accurate calculations with air, or other gases, one should consult ideal-gas tables, such as those in App. E, which tabulate h(T ) and u(T ), or integrate, using expressions for C (T ) found in text books. Once C is found, C can be calcu- p p v lated using Cv = R − Cp .
For liquids and solids the specific heat C is tabulated in Table B.4. Since it is quite difficult to maintain constant volume while the temperature is changing, C values are usually not tabulated for liquids and solids; the difference Cp − Cv is quite small. For most liquids the specific heat is relatively insensitive to temperature change. For water we will use the nominal value of 4.18 kJ/kg ⋅ K. For ice, the specific heat in kJ/kg ⋅ K is approximately C= 2.1 + 0.0069T, where T is measured in °C. The variation of specific heat with pressure is usually quite slight except forspecial situations.
EXAMPLE 4.5
The specific heat of superheated steam at approximately 150 kPa can be determined by the equation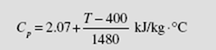
(a) What is the enthalpy change between 300 and 700°C for 3 kg of steam?
Compare with the steam tables.
(b)What is the average value of C between 300 and 700°C based on the equation and based on the tabulated data?
Solution
(a) The enthalpy change is found to be ![image_thumb[1] image_thumb[1]](http://lh5.ggpht.com/-TiIfeZ7XO0s/VE_vsLbRysI/AAAAAAAAsbQ/_q_BtrVo4N0/image_thumb%25255B1%25255D_thumb.png?imgmax=800)
From the tables we find, using P = 150 kPa, ![image_thumb[2] image_thumb[2]](http://lh3.ggpht.com/-L0ZNYV0y81o/VE_vxkwVd3I/AAAAAAAAsbg/n7MsuBlUnF4/image_thumb%25255B2%25255D_thumb.png?imgmax=800)
(b) The average value Cp, avg is found by using the relation ![]()
The integral was evaluated in part (a); hence, we have ![image_thumb[4] image_thumb[4]](http://lh6.ggpht.com/-l2jBC-R-vQA/VE_v7g-sGhI/AAAAAAAAscA/s8RNAnGmipY/image_thumb%25255B4%25255D_thumb.png?imgmax=800)
Using the values from the steam table, we have ![image_thumb[5] image_thumb[5]](http://lh3.ggpht.com/-y8TT99lbscw/VE_v_UnqX0I/AAAAAAAAscQ/KL-O3Ov-kiE/image_thumb%25255B5%25255D_thumb.png?imgmax=800)
Because the steam tables give the same values as the linear equation of this exam- ple, we can safely assume that the C (T) relationship for steam over this temperature range is closely approximated by a linear relation. This linear relation would change, however, for each pressure chosen; hence, the steam tables are essential.
EXAMPLE 4.6
Determine the enthalpy change for 1 kg of nitrogen which is heated from 300 to 1200 K by (a) using the gas tables, and (b) assuming constant specific heat.
Solution
(a) Using the gas table in App. E, find the enthalpy change to be
Δh = 36 777 − 8723 = 28 054 kJ/kmol or 28 054/28 = 1002 kJ/kg
(b) Assuming constant specific heat (found in Table B.2) the enthalpy change is found to be
Δh = Cp ΔT = 1.042 × (1200 − 300) = 938 kJ/kg
Note: the enthalpy change found by assuming constant specific heat is in error by over 6 percent. If T were closer to 300 K, say 600 K, the error would be much smaller. So, for large temperature differences, the tables should be used.
4.6 The First Law Applied to Various Processes
THE CONSTANT-TEMPERATURE PROCESS
For the isothermal process, tables may be consulted for substances for which tabulated values are available. Internal energy and enthalpy vary slightly with pressure for the isothermal process, and this variation must be accounted for in processes involving many substances. The energy equation is
Q − W = ΔU (4.28)
For a gas that approximates an ideal gas, the internal energy depends only on the temperature and thus ΔU = 0 for an isothermal process; for such a process
Q = W (4.29)
Using the ideal-gas equation PV = mRT, the work for a quasiequilibrium isothermal
THE CONSTANT-VOLUME PROCESS
The work for a constant-volume quasiequilibrium process is zero, since dV is zero. For such a process the first law becomes
Q = ΔU (4.31)
If tabulated values are available for a substance, we may directly determine ΔU. For a gas, approximated by an ideal gas with constant C , we would have
Q = mCv (T2 − T1 ) or q = Cv (T2 − T1 ) (4.32)
If nonequilibrium work, such as paddle-wheel work, is present, that work must be accounted for in the first law.
THE CONSTANT-PRESSURE PROCESS
The first law, for a constant-pressure quasiequilibrium process, was shown in Sec. 4.3 to be
Q = ΔH (4.33)
Hence, the heat transfer for such a process can easily be found using tabulated values, if available.
For a gas that behaves as an ideal gas, we have ![image_thumb[7] image_thumb[7]](http://lh3.ggpht.com/-2LRN0hukE-I/VE_wIy0AmxI/AAAAAAAAscw/6Zpwf6vamqk/image_thumb%25255B7%25255D_thumb.png?imgmax=800)
For a process involving an ideal gas for which Cp is constant there results
Q = mCp (T2 − T1 ) (4.35)
For a nonequilibrium process the work must be accounted for directly in the first
law and it cannot be expressed as P(V2 − V1 ). For such a process the above three
equations would not be valid.
THE ADIABATIC PROCESS
There are numerous examples of processes for which there is no, or negligibly small, heat transfer. The study of such processes is, however, often postponed until after the second law of thermodynamics is presented. This postponement is not necessary for an ideal gas, and because the adiabatic quasiequilibrium process is quite common, it is presented here.
The differential form of the first law for the adiabatic process is
−δW = du (4.36)
or, for a quasiequilibrium process,
du + Pdv = 0 (4.37)
To utilize this equation for substances such as water or refrigerants, we must use
tabulated properties, an impossible task at this point because we do not know a property that remains constant for this process. However, if an ideal gas can be assumed, Eq. (4.37) takes the form ![image_thumb[8] image_thumb[8]](http://lh5.ggpht.com/-y9NXv644p2g/VE_wMSNwVRI/AAAAAAAAsdA/lV7Pq1sVXic/image_thumb%25255B8%25255D_thumb.png?imgmax=800)
Rearrange and integrate between states 1 and 2, assuming constant C :![image_thumb[9] image_thumb[9]](http://lh3.ggpht.com/-pdBvdFmMRxU/VE_wPmcNt6I/AAAAAAAAsdQ/ryfYiYiHefI/image_thumb%25255B9%25255D_thumb.png?imgmax=800)
The adiabatic process for a real substance such as water, steam, or a solid will be
treated in detail in Chap. 5.
THE POLYTROPIC PROCESS
A careful inspection of the special quasiequilibrium processes for an ideal gas presented in this chapter suggests that each process can be expressed as
PV n = const. (4.41)
The work is calculated as follows: ![image_thumb[11] image_thumb[11]](http://lh6.ggpht.com/-kGTA95nZMFo/VE_wWi9oPaI/AAAAAAAAsdw/qhPv8skzLso/image_thumb%25255B11%25255D_thumb.png?imgmax=800)
except Eq. (4.30) is used if n = 1, since the above is undefined for n = 1. The heat
transfer follows from the first law.
Each quasiequilibrium process of an ideal gas is associated with a particular value for n as follows:
Isothermal: n = 1
Constant-volume: n = ∞
Constant-pressure: n = 0
Adiabatic (Cp= const): n = k
If the constant n [in Eq. (4.41)] is none of the values ∞, k, 1, or 0, then the process can be referred to as a polytropic process. For such a process involving an ideal gas, any of the equations in (4.40) can be used with k simply replaced by n; this is convenient in processes in which there is some heat transfer but which do not maintain constant temperature, pressure, or volume.
EXAMPLE 4.7
Determine the heat transfer necessary to increase the pressure of 70 percent quality steam from 200 to 800 kPa, maintaining the volume constant at 2 m3. Assume a quasiequilibrium process.
Solution
For the constant-volume quasiequilibrium process the work is zero. The first law reduces to Q = m(u2 − u1). The mass must be found. It is ![image_thumb[12] image_thumb[12]](http://lh6.ggpht.com/-9rnZ4DNbzSY/VE_waOb-X_I/AAAAAAAAseA/YqZVydhaFek/image_thumb%25255B12%25255D_thumb.png?imgmax=800)
The constant-volume process demands that v2 = v1 = 0.6203 m /kg. From the
steam tables at 800 kPa we find, by interpolation, that ![image_thumb[13] image_thumb[13]](http://lh5.ggpht.com/-0TkZmi1CGHQ/VE_we7wqkiI/AAAAAAAAseU/I-gu0Dx7n1g/image_thumb%25255B13%25255D_thumb.png?imgmax=800)
The heat transfer is then
EXAMPLE 4.8
A piston-cylinder arrangement contains 0.02 m3 of air at 50°C and 400 kPa. Heat
is added in the amount of 50 kJ and work is done by a paddle wheel until the
temperature reaches 700°C. If the pressure is held constant, how much paddle-
wheel work must be added to the air? Assume constant specific heats.
Solution
The process cannot be approximated by a quasiequilibrium process because of the paddle-wheel work. Thus, the heat transfer is not equal to the enthalpy change. The first law may be written as
To find m we use the ideal-gas equation. It gives us ![image_thumb[16] image_thumb[16]](http://lh4.ggpht.com/-VC8Y18S9E-4/VE_wpcoPUTI/AAAAAAAAsfE/9kHc-R9EU10/image_thumb%25255B16%25255D_thumb.png?imgmax=800)
From the first law the paddle-wheel work is found to be ![image_thumb[17] image_thumb[17]](http://lh3.ggpht.com/-8rdIzyZ-hcc/VE_wuLnPhtI/AAAAAAAAsfU/qtTJjy29DoQ/image_thumb%25255B17%25255D_thumb.png?imgmax=800)
EXAMPLE 4.9
Calculate the work necessary to compress air in an insulated cylinder from a volume of 2 m3 to a volume of 0.2 m3. The initial temperature and pressure are
20°C and 200 kPa, respectively.
Solution
We will assume that the compression process is approximated by a quasiequilibrium process, which is acceptable for most compression processes, and that the process is adiabatic due to the presence of the insulation (we usually assume an adiabatic process anyhow since heat transfer is assumed to be negligible). The first law is then written as ![image_thumb[18] image_thumb[18]](http://lh4.ggpht.com/-aLg2yu-SdHw/VE_wxzWFD-I/AAAAAAAAsfk/u3HxfKPcQz8/image_thumb%25255B18%25255D_thumb.png?imgmax=800)
The final temperature T2 is found for the adiabatic quasiequilibrium process from Eq. (4.40); it is



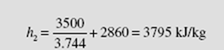
![image_thumb[6] image_thumb[6]](http://lh5.ggpht.com/--qF4w6AeYks/VE_wF_IShdI/AAAAAAAAscg/_J1LwpTn-wI/image_thumb%25255B6%25255D_thumb.png?imgmax=800)
![image_thumb[10] image_thumb[10]](http://lh5.ggpht.com/-T3I-rTJBXJc/VE_wTm97lFI/AAAAAAAAsdg/mlDG_7gm1Ak/image_thumb%25255B10%25255D_thumb.png?imgmax=800)
![image_thumb[15] image_thumb[15]](http://lh6.ggpht.com/-JukKRKrVLwc/VE_wlyG_lqI/AAAAAAAAse0/DJNrVewhPbU/image_thumb%25255B15%25255D_thumb.png?imgmax=800)
![image_thumb[19] image_thumb[19]](http://lh6.ggpht.com/-wrj6v08JU2A/VE_w0wKblDI/AAAAAAAAsf0/XxBUri5hRVo/image_thumb%25255B19%25255D_thumb.png?imgmax=800)