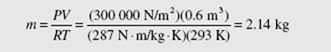Properties of Pure Substances
In this chapter the relationships between pressure, specific volume, and temperature will be presented for a pure substance. A pure substance is homogeneous, but may exist in more than one phase, with each phase having the same chemical composition. Water is a pure substance; the various combinations of its three phases (vapor, liquid, ice) have the same chemical composition. Air in the gas phase is a pure substance, but liquid air has a different chemical composition. Air is not a pure substance if it exists in more than one phase. In addition, only a simple compressible substance, one that is essentially free of magnetic, electrical, or surface tension effects, will be considered.
2.1 The P-v-T Surface
It is well known that a substance can exist in three different phases: solid, liquid, and gas. Assume that a solid is contained in a piston-cylinder arrangement such that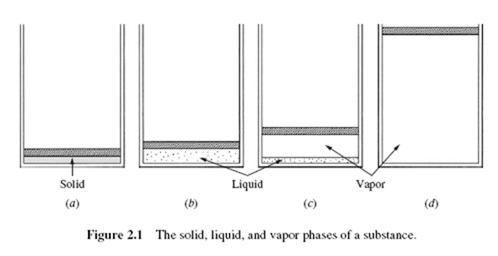
the pressure is maintained at a constant value; heat is added to the cylinder, causing the substance to experience all three phases, as in Fig. 2.1. We will record the temperature T and specific volume v during the experiment. Start with the solid at some low temperature, as in Fig. 2.2a; then add heat until it is all liquid (v does not increase very much). After all the solid is melted, the temperature of the liquid again rises until vapor just begins to form; this state is called the saturated liquid state. During the phase change from liquid to vapor,1 called vaporization, the temperature remains constant as heat is added. Finally, all the liquid is vaporized and the state of saturated vapor exists, after which the temperature again rises with heat addition. Note, the specific volumes of the solid and liquid are much less than the specific volume of vapor at relatively low pressures.
If the experiment is repeated a number of times using different pressures, a T-v diagram results, shown in Fig. 2.2b. At pressures that exceed the pressure of the critical point, the liquid simply changes to a vapor without a constant-temperature vaporization process. Property values of the critical point for various substances are included in Table B.3.
The experiment could also be run by holding the temperature fixed and decreasing the pressure, as in Fig. 2.3a (the solid is not displayed). The solid would change to a liquid, and the liquid to a vapor, as in the experiment that led to Fig. 2.2. The T–v diagram, with only the liquid and vapor phases shown, is displayed in Fig. 2.3b.
The process of melting, vaporization, and sublimation (the transformation of a solid directly to a vapor) are shown in Fig. 2.3c. Distortions are made in all three diagrams so that the various regions are displayed. The triple point is the point where all three phases exist in equilibrium together. A constant pressure line is
Figure 2.2 The T–v diagram.
shown on the T-v diagram and a constant temperature line on the P-v diagram; one of these two diagrams is often sketched in problems involving a phase change from a liquid to a vapor.
Primary practical interest is in situations involving the liquid, liquid-vapor, and vapor regions. A saturated vapor lies on the saturated vapor line and a saturated liquid on the saturated liquid line. The region to the right of the saturated vapor line is the superheated region; the region to the left of the saturated liquid line is the compressed liquid region (also called the subcooled liquid region). A supercritical state is encountered when the pressure and temperature are greater than the critical values.
Figure 2.3 The (a) P-v, (b) T-v, and (c) P-T diagrams.
2.2 The Liquid-Vapor Region
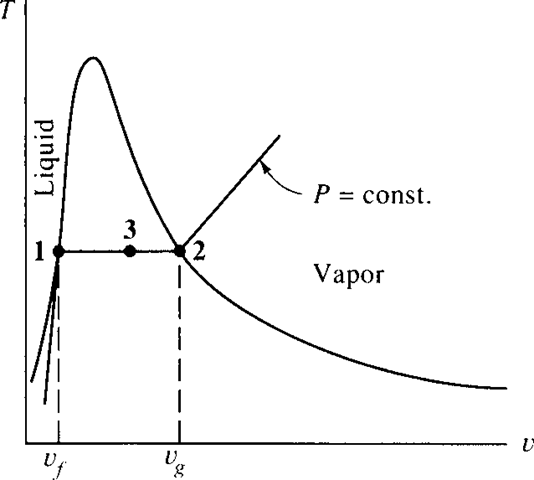
At any state (T, v) between saturated points f (state 1) and g (state 2), shown in Fig. 2.4, liquid and vapor exist in equilibrium. Let vf and vg represent, respectively, the specific volumes of the saturated liquid and the saturated vapor. Let m be the total mass of a system, mthe amount of mass in the liquid phase, and m the amount of mass in the vapor phase. Then, for a state of the system represented by any (T, v), such as state 3, the total volume of the mixture is the sum of the volume occupied by the liquid and that occupied by the vapor, or
V = Vf + Vg or mv = m f v f + mg vg (2.1)
The ratio of the mass of saturated vapor to the total mass is called the quality of the mixture, designated by the symbol x; it is
x = mg/m (2.2)
We often refer to the region under the saturation lines as the quality region, or the mixture region, or the wet region; it is the only region where quality x has a meaning.
Recognizing that m = mf + mg we may write Eq. (2.1), using our definition of quality, as
v = v f + x(vg − v f ) (2.3)
Figure 2.4 A T-v diagram showing the saturated liquid and saturated vapor points.
Because the difference in saturated vapor and saturated liquid values frequently appears in calculations, we often let the subscript “fg” denote this difference; that is,
v fg = vg − v f (2.4)
Thus, Eq. (2.3) can be written as
v = v f + xv fg (2.5)
The percentage liquid by mass in a mixture is 100(1 – x), and the percentage vapor is 100x.
2.3 The Steam Tables
Tabulations have been made for many substances of the thermodynamic properties P, v, and T and additional properties to be identified in subsequent chapters. Values are presented in the appendix in both tabular and graphical form. Table C.1 gives the saturation properties of water as a function of saturation temperature; Table C.2 gives these properties as a function of saturation pressure. The information contained in the two tables is essentially the same, the choice of which to use being a matter of convenience. We should note, however, that in the mixture region pressure and temperature are dependent. Thus, to establish the state of a mixture, if we specify the pressure, we need to specify a property other than temperature. Conversely, if we specify temperature, we must specify a property other than pressure.
Table C.3 lists the properties of superheated steam. To establish the state in the superheated region, it is necessary to specify two properties. While any two may be used, the most common procedure is to use P and T since these are easily measured. Thus, properties such as v are given in terms of the set of independent properties P and T.
Table C.4 lists data pertaining to compressed liquid. At a given T the specific volume v of of 100°C in Table C.1, the specific volume vf of liquid is 0.001044 m /kg at a pressure of l00 kPa. At a pressure of 10 MPa, the specific volume is 0.001038 m /kg,
less than 1 percent decrease in specific volume. Thus, it is common in calculations to assume that v (and other properties, as well) of a compressed liquid is equal to vf at the same temperature. Note, however, that vf increases significantly with temperature, especially at higher temperatures.
Table C.5 gives the properties of a saturated solid and a saturated vapor for an equilibrium condition. Note that the value of the specific volume of ice is relatively
insensitive to temperature and pressure for the saturated-solid line. Also, it has a greater value (almost 10 percent greater) than the minimum value on the saturated- liquid line.
Appendix D provides the properties of refrigerant R134a, a refrigerant used often in air conditioners and commercial coolers.
EXAMPLE 2.1
Determine the volume change when 10 kg of saturated water is completely vaporized at a pressure of (a) 1 kPa, (b) 260 kPa, and (c) 10 000 kPa.
Solution
Table C.2 provides the necessary values. The quantity being sought is ΔV = mvfg where vfg = vg – vf . Note that P is given in MPa.
(a) 1 kPa = 0.001 MPa. Thus, vfg = 129.2 – 0.001 = 129.2 m3 /kg.
... ΔV = 1292 m3
(b) At 0.26 MPa we must interpolate2 if we use the tables. The tabulated values are used at 0.2 MPa and 0.3 MPa:
The value for vf is, to four decimal places, 0.0011 m3 /kg at 0.2 MPa and at 0.3 MPa; hence, no need to interpolate for vf . We then have
v fg = 0.718 − 0.0011 = 0.717 m3 / kg. ∴ ΔV = 7.17 m3
(c) At 10 MPa, vfg = 0.01803 − 0.00145 = 0.01658 m3 /kg so that
ΔV = 0.1658 m3
Notice the large value of vfg at low pressure compared with the small value of vfg as the critical point is approached. This underscores the distortion of the ufgT-v diagram in Fig. 2.4.
EXAMPLE 2.2
Four kilograms of water are placed in an enclosed volume of 1 m3. Heat is added until the temperature is 150°C. Find the (a) pressure, (b) mass of the vapor, and (c) volume of the vapor.
Solution
Table C.1 is used. The volume of 4 kg of saturated vapor at 150°C is 0.3928 × 4 = 1.5712 m3. Since the given volume is less than this, we assume the state to be in the quality region.
(a) In the quality region, the pressure is given as P = 475.8 kPa, the value next to the temperature.
(b) To find the mass of the vapor we must determine the quality. It is found from Eq. (2.3), using v = 1/4 = 0.25 m3/kg:
v = v f + x(vg − v f )
0.25 = 0.00109 + x(0.3928 − 0.00109) ∴ x = 0.6354
Using Eq. (2.2), the vapor mass is
mg = mx = 4 × 0.6354 = 2.542 kg
(c) Finally, the volume of the vapor is found from
Vg = vg mg = 0.3928 × 2.542 = 0.998 m3
Note: In mixtures where the quality is not close to zero, the vapor phase occupies most of the volume. In this example, with a quality of 63.5 percent, it occupies 99.8 percent of the volume.
EXAMPLE 2.3
Two kilograms of water are heated at a pressure of 220 kPa to produce a mixture with quality x = 0.8. Determine the final volume occupied by the mixture.
Solution
Use Table C.2. To determine the appropriate values at 220 kPa, linearly interpolate between 0.2 and 0.25 MPa. This provides, at 220 kPa,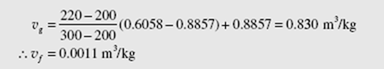
Note, no interpolation is necessary for vf since, for both pressures, vf is the same to four decimal places. Using Eq. (2.3), we now find
The total volume occupied by 2 kg is
V = mv = 2 kg × 0.662 m3 /kg = 1.32 m3
Two kilograms of water are contained in a constant-pressure cylinder held at 2.2 MPa. Heat is added until the temperature reaches 800°C. Determine the final volume of the container.
Solution
Use Table C.3. Since 2.2 MPa lies between 2 MPa and 2.5 MPa, the specific volume is interpolated to be
v = 0.2467 + 0.4(0.1972 − 0.2467) = 0.227 m3 / kg
The final volume is then
V = mv = 2 × 0.227 = 0.454 m3
The linear interpolation above results in a less accurate number than the numbers in the table. So, the final number has fewer significant digits.
2.4 Equations of State
When the vapor of a substance has relatively low density, the pressure, specific volume, and temperature are related by an equation of state,
Pv = RT (2.6)
where, for a particular gas, the gas constant is R. A gas for which this equation is valid is called an ideal gas or sometimes a perfect gas. Note that when using the above equation of state the pressure and temperature must be expressed as absolute quantities.
The gas constant R is related to a universal gas constant R, which has the same value for all gases, by the relationship
where M is the molar mass, values of which are tabulated in Tables B.2 and B.3. The mole is that quantity of a substance (i.e., that number of atoms or molecules) having a mass which, measured in grams, is numerically equal to the atomic or molecular weight of the substance. In the SI system it is convenient to use instead the kilomole (kmol), which amounts to x kilograms of a substance of molecular weight x. For instance, 1 kmol of carbon (with a molecular weight of 12) is a mass of 12 kg; 1 kmol of oxygen is 32 kg. Stated otherwise, M = 32 kg/kmol for O2.
The value R = 8.314 kJ/kmol·K. For air M is 28.97 kg/kmol, so that for air R is 1.287 kJ/ kg·K, or 287 J/kg·K, a value used extensively in calculations involving air.
Other forms of the ideal-gas equation are
PV = mRT P = pRT PV = n R T (2.8)
where n is the number of moles.
Care must be taken in using this simple convenient equation of state. A low- density can result from either a low pressure or a high temperature. For air, the ideal- gas equation is surprisingly accurate for a wide range of temperatures and pressures; less than 1 percent error is encountered for pressures as high as 3000 kPa at room temperature, or for temperatures as low as −130°C at atmospheric pressure.
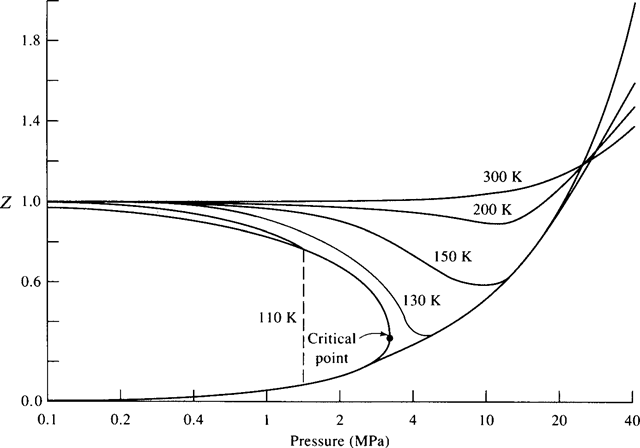
The compressibility factor Z helps us in determining whether or not the ideal-gas equation can be used. It is defined as
and is displayed in Fig. 2.5 for nitrogen. This figure is acceptable for air also, since air is composed mainly of nitrogen. If Z = 1, or very close to 1, the ideal-gas equation can be used. If Z is not close to 1, then Eq. (2.9) may be used.
The compressibility factor can be determined for any gas by using a generalized compressibility chart presented in App. G. In the generalized chart the reduced pressure P and reduced temperature T must be used. They are calculated from
Figure 2.5 The compressibility factor.
EXAMPLE 2.5
An automobile tire with a volume of 0.6 m3 is inflated to a gage pressure of 200 kPa. Calculate the mass of air in the tire if the temperature is 20°C using the ideal-gas equation of state.
Solution
Air is assumed to be an ideal gas at the conditions of this example. In the ideal-gas equation, PV = mRT, we use absolute pressure and absolute temperature. Thus, using Patm = 100 kPa (to use a pressure of 101 kPa is unnecessary; the difference of 1 percent is not significant in most engineering problems):
The mass is then calculated to be
Be careful to be consistent with the units in the above equation.