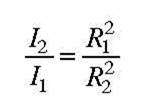Waves and Sound
In This Chapter:
✔ Waves
✔ Wave Properties
✔ Logarithms
✔ Sound
✔ Doppler Effect
Waves
A wave is, in general, a disturbance that moves through a medium. An exception is an electromagnetic wave, which can travel through a vacuum. Examples are light and radio waves. A wave carries energy, but there is no transport of matter. In a periodic wave, pulses of the same kind follow one another in regular succession.
In a transverse wave, the particles of the medium move back and forth perpendicular to the direction of the wave. Waves that travel down a stretched string when one end is shaken are transverse (Figure 10-1).
In a longitudinal wave, the particles of the medium move back and forth in the same direction as the wave. Waves that travel down a coil spring when one end is pulled out and released are longitudinal (Figure 10-2). Sound waves are also longitudinal.
Figure 10-2
Wave Properties
The period T of a wave is the time required for one complete wave to pass a given point. The frequency f is the number of waves that pass that point per second [Figure 10-3(a)], so
f = 1/T
Frequency =1/period
The wavelength l (Greek letter lambda) of a periodic wave is the dis- tance between adjacent wave crests (Figure 10-3(b)). Frequency and wavelength are related to wave velocity by
The amplitude A of a wave is the maximum displacement of the particles of the medium through which the wave passes on either side of their equilibrium position. In a transverse wave, the amplitude is half the distance between the top of a crest and the bottom of a trough (Figure 10-3).
The intensity I of a wave is the rate at which it transports energy per unit area perpendicular to the direction of motion. The intensity of a mechanical wave (one that involves moving matter, in contrast to, say, an electromagnetic wave) is proportional to f 2, the square of its frequency, and, to A2 the square of its amplitude.
Solved Problem 10.1 The velocity of sound in seawater is 1531 m/s. Find the wavelength in seawater of a sound wave whose frequency is 256 Hz.
Logarithms
Although logarithms have many other uses, their chief application in ap- plied physics is in connection with the decibel, which is described in the next section. Logarithms are discussed here only to the extent required for this purpose.
The logarithm of a number N is the power n to which 10 must be raised in order that 10n = N. That is,
N = 10n therefore log N = n
(Logarithms are not limited to a base of 10, but base-10 logarithms are the most common and are all that are needed here.) For instance,
|
1000 = 103 |
therefore |
log 1000 = 3 |
|
0.01 = 10−2 |
therefore |
log 0.01 = −2 |
To find the logarithm of a number with a calculator, enter the value of the number and press the LOG button.
The antilogarithm of a quantity n is the number N whose logarithm it is. That is,
If log N = n then antilog n = N
To find the antilogarithm with a calculator, enter the value of the logarithm and press the INV LOG button.
Because of the way logarithms are defined, the logarithm of a product equals the sum of the logarithms of the factors:
log xy = log x + log y
Other useful relations are
log (x/y) = log x − log y
log x n = n log x
Sound
Sound waves are longitudinal waves in which al- ternate regions of compression and rarefaction move away from a source. Sound waves can travel through solids, liquids, and gases. The velocity of sound is a constant for a given material at a given pressure and temperature; in air at 1atm pressure and 20 °C, it is 343 m/s = 1125 ft/s.
When sound waves spread out uniformly in space, their intensity de- creases inversely with the square of the distance R from their source. Thus, if the intensity of a certain sound is I1 at the distance R1, its intensity I2 at the distance R2 can be found from
The response of the human ear to sound intensity is not proportion- al to the intensity, so doubling the actual intensity of a certain sound does not lead to the sensation of a sound twice as loud but only of one that is slightly louder than the original. For this reason, the decibel (dB) scale is used for sound intensity.
An intensity of 10−12 W/m2, which is just audible, is given the value 0 dB; a sound 10 times more intense is given the value 10 dB; a sound 102 times more intense than 0 dB is given the value of 20 dB; a sound 103 times more intense than 0 dB is given the value of 30 dB; and so forth. More formally, the intensity I dB of a sound wave whose intensity is I W/m2 is given by
where I o= 10−12 W/m2. Normal conversation might be 60 dB, city traffic noise might be 90 dB, and a jet aircraft might produce as much as 140 dB (which produces damage to the ear) at a distance of 100 ft. Long-term exposure to intensity levels of over 85 dB usually leads to permanent hearing damage.
Solved Problem 10.2 How many times more intense is a 50-dB sound than a 40-dB sound? Than a 20-dB sound?
Solution. Each interval of 10 dB represents a change in sound intensity by a factor of 10. Hence a 50-dB sound is 10 times more intense than a 40-dB sound and 10 × 10 × 10 = 1000 times more intense than a 20-dB sound.
Doppler Effect
When there is relative motion between a source of waves and an observer, the apparent frequency of the waves is different from their frequency fS at the source. This change in frequency is called the Doppler effect. When the source approaches the observer (or vice versa), the observed frequency is higher; when the source recedes from the observer (or vice versa), the observed frequency is lower. In the case of sound waves, the frequency f that a listener hears is given by
In this formula, v is the velocity of sound, vL is the velocity of the listener (considered positive for motion toward the source and negative for motion away from the source), and vS is the velocity of the source (considered positive for motion toward the listener and negative for motion away from the listener).
The Doppler effect in electromagnetic waves (light and radio waves are examples) obeys the formula
Here c is the velocity of light (3.00 × 108 m/s), and v is the relative velocity between source and observer (considered positive if they are approaching and negative if they are receding).

![Waves and Sound ]_Page_073_Image_0001 Waves and Sound ]_Page_073_Image_0001](http://lh3.ggpht.com/-jiAuKSpul9Q/VE5ZZvjK48I/AAAAAAAAre4/xoXewRh7ZEk/Waves%252520and%252520Sound%252520%25255D_Page_073_Image_0001_thumb.jpg?imgmax=800)
![Waves and Sound ]_Page_073_Image_0002 Waves and Sound ]_Page_073_Image_0002](http://lh3.ggpht.com/-Y_xPT0Sw4Ts/VE5Zou0yCuI/AAAAAAAArfI/WOrapG5pGsg/Waves%252520and%252520Sound%252520%25255D_Page_073_Image_0002_thumb.jpg?imgmax=800)
![Waves and Sound ]_Page_074_Image_0001 Waves and Sound ]_Page_074_Image_0001](http://lh3.ggpht.com/-tjXmkzAYM0o/VE5Z_V-joXI/AAAAAAAArfo/apkdINwGGW8/Waves%252520and%252520Sound%252520%25255D_Page_074_Image_0001_thumb%25255B1%25255D.jpg?imgmax=800)