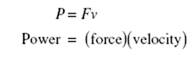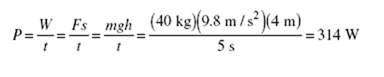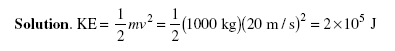Energy
In This Chapter:
✔ Work
✔ Power
✔ Kinetic Energy
✔ Potential Energy
✔ Conservation of Energy
Work
Work is a measure of the amount of change (in a general sense) that a force produces when it acts on a body. The change may be in the velocity of the body, in its position, or in its size or shape.
By definition, the work done by a force acting on a body is equal to the product of the force and the distance through which the force acts, provided that F and s are in the same direction. Thus
W = Fs
Work = (force)(distance)
Work is a scalar quantity; no direction is associated with it.
If F and s are not parallel but F is at the angle θ with respect to s,
then
W = Fs cos θ
Since cos 0° = 1, this formula becomes W = Fs when F is parallel to s. When F is perpendicular to s, θ = 90° and cos 90° = 0. No work is done in this case (Figure 4-1).
Figure 4-1
The unit of work is the product of a force unit and a length unit. In SI units, the unit of work is the joule ( J).
SI units: 1 joule ( J) = 1 newton-meter = 0.738 ft•lb
Solved Problem 4.1 A horizontal force of 420 N is used to push a 100- kg crate for 5 m across a level warehouse floor. How much work is done?
Solution. The mass of the crate does not matter here. Since the force is parallel to the displacement,
W = Fs = (420 N)(5 m) = 2100 J = 2.1 kJ
Power
Power is the rate at which work is done by a force. Thus
The more power something has, the more work it can perform in a given time.
Two special units of power are in wide use, the watt and the horse- power, where
I watt (W) = 1 J/s = 1.34 × 10−3 hp
I horsepower (hp) = 550 ft·lb/s = 746 W
When a constant force F does work on a body that is moving at the constant velocity v, if F is parallel to v, the power involved is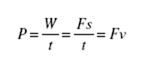 because s/t = v; that is
because s/t = v; that is
Solved Problem 4.2 A 40-kg woman runs up a staircase 4 m high in 5 s. Find her minimum power output.
Solution. The minimum downward force the woman’s legs must exert is equal to her weight mg. Hence
Energy is that property something has that enables it to do work. The more energy something has, the more work it can perform. Two general categories of energy are kinetic energy and potential energy.
Note!
The units of energy are the same as those of work, namely the joule and the foot-pound.
The energy a body has by virtue of its motion is called kinetic energy. If the body’s mass is m and its velocity is v, its kinetic energy is
Kinetic energy = KE =(1/2) mv2
Solved Problem 4.3 Find the kinetic energy of a 1000-kg car whose velocity is 20 m/s.
Solution.
KE =(1/2) mv2 = (1/2) (1000 kg)(20 m / s)2 = 2 × 105 J
Potential Energy
The energy a body has by virtue of its position is called potential energy. A book held above the floor has gravitational potential energy because the book can do work on something else as it falls; a nail held near a magnet
has magnetic potential energy because the nail can do work as it moves toward the magnet; the wound spring in a watch has elastic potential energy because the spring can do work as it unwinds.
The gravitational potential energy of a body of mass m at a height h
above a given reference level is:
Gravitational potential energy = PE = mgh
where g is the acceleration due to gravity.
Solved Problem 4.4 A 1.5-kg book is held 60 cm above a desk whose top is 70 cm above the floor. Find the potential energy of the book (a) with respect to the desk, and (b) with respect to the floor.
Solution.
(a) Here h = 60 cm = 0.6 m, so
PE = mgh = (1.5 kg)(9.8 m/s2)(0.6 m) = 8.8 J
(b) he book is h = 60 cm + 70 cm = 130 cm = 1.3 m above the floor, so its PE with respect to the floor is
PE = mgh = (1.5 kg)(9.8 m/s2)(1.3 m) = 19.1 J
Conservation of Energy
According to the law of conservation of energy, energy cannot be creat- ed or destroyed, although it can be transformed from one kind to anoth- er. The total amount of energy in the universe is constant. A falling stone provides a simple example: More and more of its initial potential energy turns to kinetic energy as its velocity increases, until finally all its PE has become KE when it strikes the ground. The KE of the stone is then trans- ferred to the ground as work by the impact.
In general,
Work done on an object = change in object’s KE + change in object’s PE + work done by object
Work done by an object against friction becomes heat.