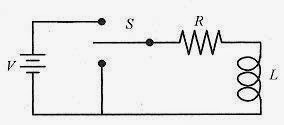
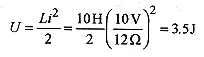How To Solve Physics Problems R-L Circuits problems and solutions

R-L Circuits

Our first look at R-L circuits is with a simple word description of the behavior of the current in the circuit of Fig. 39-1. After this general description of how the circuit behaves, we will take a closer look at the mathematical description. The important point to keep in mind in this discussion is that the voltage across an inductor is proportional to the rate of change in current.

Increasing Current

Fig. 39-1

When the switch is closed to place the resistor and inductor in series with the battery, current begins to flow in the circuit. Because of the self-induced emƒf in the inductor, that is proportional to the rate of change of current, all of the battery voltage does not instantaneously appear across the resistor. The current in the circuit rises in an exponential manner to (its final value of) V/R according to equation 39-1.

This function is consistent with our understanding of inductors: as time goes on the current rises to V/R.

When the battery voltage is applied to R and L in series, voltages across these components vary with time. We can, however, write a Kirchhoff-type voltage statement that is valid for all time.

which can be solved for i. The solution is similar to the one for the R-C circuit done in an earlier chapter. A review of that more detailed analysis may be helpful before continuing. 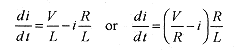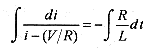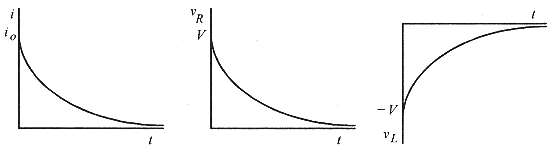Rewrite this equation as Switching to a more convenient form for integration Integrating with a change of variable as before Switching to exponentials The constant, K, can be evaluated in either of these equations by imposing the initial condition that at t = 0, i = 0, so K = –V/R and The time constant in the circuit is L/R. The rate of change of current in the circuit is There are three graphs (Fig. 39-2) that describe how the voltage and current vary in the circuit.

The graph of i = (V/R)(1-e–Rt/L) versus t shows how the current in the circuit varies with time.

The graph of vR = iR = V(1-e-Rt/L) versus t shows the exponential rise of voltage across R.

The graph of vL = L(di/dt) = Ve-Rt/L versus t shows the exponential decay of voltage across L.

Fig. 39-2

As an exercise show that the Kirchhoff equation V = iR + L(di/dt) is satisfied by substituting the expressions for i and di/dt. The analysis is also verified by the graphs: the voltage across the resistor is growing in a one minus exponential manner while the voltage across the inductor is decaying in an exponential manner.

39-1 Place a 60V battery in series with an inductor of 50×10-3H and a resistor of 180Ω. What is the current and the rate of change of current at t = 0?

Solution: Using and it is clear that at t =0, i = 0,

but

<><><><><><><><><><><><>

39-2 For the same circuit, what is the time constant?

Solution: The time constant

<><><><><><><><><><><><>

39-3 For the same circuit, what is the rate of current increase at t = 1.0×10-4 s?

Solution: Using

<><><><><><><><><><><><>

39-4 For the same circuit described in problem 39-3, what is the rate of current increase when i has reached 90% of its final value?

Solution: Translated into mathematics, the question reads, “Find di/dt when i =0.90(V/R).” First find t when i = 0.90(V/R). Set i = 0.90V/R in equation 39-1 and solve for t. Solve for t by switching to logarithms: t = 2.8×10-4 1n10 = 6.4×10-4 s

This is not a common type of problem, so go back over these equations and be sure you know how to manipulate the exponents and logarithms.

Now place this time in equation 39-2.

<><><><><><><><><><><><>

39-5 Construct the circuit of Fig. 39-1 with an inductor of 10 H, a resistor of 12 Ω, and a 10 V battery. Calculate the time constant, the rate of change of current, and the voltages across the resistor and inductor at one time constant.

Solution: The time constant is The rate of change of current is The voltage across the resistor is vR = iR = V(1-e-t/0.83s) = 10(1-e-1)V = 6.3 V. The voltage across the inductor is

Decreasing Current.

Referring back to Fig. 39-1, suppose that the current has reached the steady state condition, the R and L are placed in series, and the battery removed. At the instant the battery is removed there is no voltage across the inductor. The circuit has reached steady state and (di/dt) = 0 so L(di/dt) = vL is zero. There is, however, full battery voltage across the resistor. This voltage drops as the current in the circuit drops in an exponential manner according to equation 39-3. In this situation the loop equation is 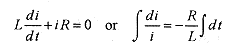which has solution The constant can be obtained by imposing the initial conditions. At t = 0 (in decay mode), i = io. The io has the value V/R (initially all the battery voltage is across the resistor). With the constant evaluated and The voltage across the resistor is vR = iR=Ve-Rt/L.

The voltage across the inductor is Graphs of i,vR, and vL are shown in Fig. 39-3.

Fig. 39-3

39-6 For the circuit described in problem 39-5 operating in decreasing current mode, calculate the time for the voltage to decay to one-half the steady state value. Then find the voltages across R and L at this time.

Solution: The time constant, calculated earlier, is 0.83s. The time for the current to drop to onehalf the steady state value is found by replacing i in equation 39-3 with 0.50io/sub>. A more convenient form for logarithms is Taking logarithms and remembering that 1n(1/2)= 1n1 – 1n2 = 0-1n2 = -1n2, The voltage across the inductor is The voltage across the resistor is The voltages vR + vL add to zero as they must since they are the entire circuit!

Energy Storage

The energy stored in an inductor is, from Chapter 38, Inductance, 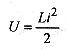In this circuit in the increasing current mode, energy is being stored in the magnetic field associated with the current in the coil. In the decreasing current mode the energy stored in the magnetic field of the coil leaves as the magnetic field and current collapse. This energy is dissipated as heat in the resistor.