Faraday’s Law
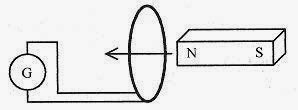
There are two basic experiments demonstrating Faraday’s law. The first experiment involves passing a magnet through a loop of wire. If a magnet is passed through a loop of wire connected to a galvanometer as shown in Fig. 37-1 then three things are observed:
Fig. 37-1
1) a current is observed in the loop when the magnet is moving,
2) the direction of the current depends on the direction of the magnet,
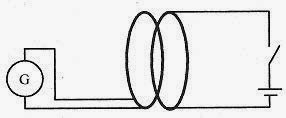
3) the magnitude of the deflection (current) is proportional to the strength of the magnet and its velocity. The second Faraday experiment involves two loops of wire. If the current in the primary loop (the one with the battery) is changed, then there is a current in the secondary loop (the one with the galvanometer).
Fig. 37-2
If the switch connecting the battery to the loop is opened and closed three things are observed:
1) a current in the galvanometer loop is observed when the switch is closed,
2) a current in the opposite direction is observed when the switch is opened,
3) no current in the galvanometer loop is observed when there is steady or zero current in the battery loop.
These two experiments are the basis for a statement of Faraday’s law. An emf and current is induced in a loop when there is a change in magnetic field in the loop. The magnitude of the induced emf is proportional to the rate of change in magnetism.
The amount of magnetism is conveniently envisioned as lines of flux φ. For a constant magnetic field, and area normal to the field, flux is related to magnetic field through ![]()
The magnetic field can be envisioned as a collection of (magnetic) lines (of flux) with a higher magnetic field being associated with a higher density of flux lines (more lines of flux per square meter). Picture a square frame one meter on a side oriented normal to the direction of a magnetic field. A stronger (higher Tesla) field is viewed as one with more lines passing through this frame. There are no physical lines of flux. This is a convenient construct that allows us to visualize magnetic fields.
The formal definition of flux involves an integral that looks difficult, but in concept it is relatively easy to understand and in practice is often done by inspection. The vector ds is a vector normal to a surface. B is the vector representing the field passing through this surface. If B and ds are pointing in the same direction the integral reduces to equation 37-1. Even when an integral is required most of the time B is at a fixed angle with respect to ds so the integral involves the area and an angle without any formal integration.
The unit of flux is Tesla-meter square which has a special name, Weber. One Weber’s worth of flux passing through a square meter produces a field strength of one Tesla.
The Faraday’s law specifically states that the emf induced in a closed loop is the change in flux per time.
The delta form of this statement implies that the emf is the average emf over the time interval. The minus sign is a reminder of the convention for determining the direction of the emf. For multiple turns of a coil the emf is just the number of turns times this change in flux over time. The direction of the induced emf is such as to produce a magnetic field in opposition to the field that created the emf. This will be discussed in detail in the context of a problem. Now look at several means for generating emf’s in wires.
This induced emf is different from the emf produced by a battery. In the case of a battery, a terminal voltage can be measured and the emf of the battery can be associated with the battery raising the potential of charges passing through it. In the case of a loop of wire there are no terminals, yet there is a current so we say that this current must be due to an electromotive force, a force that makes electric charges move. While there are no terminals to measure voltage in the loop, the current in the loop due to magnetic induction is just as real as the current produced by a battery.
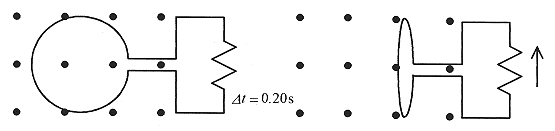
37-1 Place a 20 turn coil of radius 0.050m inside a solenoid where the magnetic field is changed from 40 × 10-3 Tin one direction to 40 × 10-3T in the other direction in 60 × 10-3s. Find the induced emf.
Fig. 37-3
Solution: Inside the coil the flux is Φ= BA = 40 × 10-3 Tπ(0.05m)2=3.1 × 10-4 Wb.
The change in flux is ΔΦ = 6.2 × 10-4 Wb, and this occurs over 60 × 10-3 s in the coil of 20 turns so the induced emf is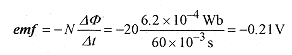
Lenz’s Law
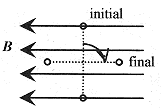
To determine the direction of the induced current look at the coil and the direction of the initial and final magnetic fields through the coil. The field originally pointing to the right collapses to zero and then grows to the left to its final value. The current is physically constrained by the wire to go only in one of two directions. If the current in the coil were such that the field produced by this current grew in the same direction as the field that initiated the current then the current would continue to grow (because the field would continue to change in the same direction). This would mean that any time a field changed in a loop of wire the current in the loop would grow without limit. This is clearly contrary to nature. Lenz’s law, applied to this situation, states that an induced current is in a direction so as to produce a field that opposes the changing field that initiated the current. This will be shown in subsequent problems.
37-2 A circular loop 0.040m in radius is perpendicular to a magnetic field of 0.50T. The loop is stretched, across a diameter, so that its area goes to zero in 0.20s. What is the induced voltage and direction of the current through the resistor?
Solution: The flux through the loop is Φ=BA=0.50T.Δ(0.04m)2 =2.5×10-3Wb.
The emf is
Fig. 37-4
The current is such as to produce a magnetic field out of the page (or in opposition to the collapsing magnetic field). The current is counterclockwise in the stretched loop and up on the resistor.
<><><><><><><><><><><><>
37-3 Take a 50 turn rectangular coil of dimensions 0.10m by 0.20m and rotate it from a position perpendicular to a field of 0.50T to parallel to the field in 0.10s and calculate the induced emf.
Fig. 37-5
Solution: The flux Φ=BA. In addition, ΔΦ=BA since the flux goes from maximum to zero. ![]() The coil is 50 turns, so the induced emf is
The coil is 50 turns, so the induced emf is 
37-4 Now instead of flipping the coil from perpendicular to parallel, rotate it with uniform angular velocity.
Solution: The expression for the flux, assuming the rotation is clockwise is ![]()
The coil rotates through an angle of Δ/2 in 0.10s so φ=0.50T.2.0 × 10-2 m2 cos(Δt/0.20s)
The units in this calculation are helpful in understanding the emf generated in a loop. A T.m2 is a Weber, a measure of the number of lines of flux. Webers per second is the number of flux lines per second that are changed within a loop. One Weber’s worth of flux lines per second generates an emf of one Volt in the loop.
<><><><><><><><><><><><>
37-5 Now find the average emf induced over this time. This is the average value of the instantaneous emf function over this one-quarter cycle.
Solution: This requires the concept of the average value of a function from integral calculus. Review this procedure in the Introduction, Mathematical Background, if necessary. This integral is ![]() The integral is done over θ for convenience rather than over ωt and a time interval. The average value of the first quarter cycle of the sine function is the same as the average value for the first half cycle of the sine function and the same as the absolute value of the average value of the entire sine function. This is an excellent example of a problem where the average emf can be calculated using either ΔΦ/Δt (problem 37-3) or the average value of the function for emf.
The integral is done over θ for convenience rather than over ωt and a time interval. The average value of the first quarter cycle of the sine function is the same as the average value for the first half cycle of the sine function and the same as the absolute value of the average value of the entire sine function. This is an excellent example of a problem where the average emf can be calculated using either ΔΦ/Δt (problem 37-3) or the average value of the function for emf.
<><><><><><><><><><><><>
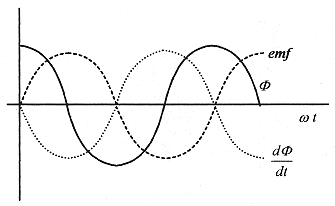
37-6 For the coil and magnetic field situation of Fig. 37-5 consider continuous rotation at some angular velocity ω. The maximum flux through the coil is Φ0.
Solution: The flux is Φ=Φ0cosωt.’
The time rate of change of flux is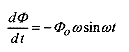
These three quantities are shown in Fig. 37-6.
Fig. 37-6
<><><><><><><><><><><><>
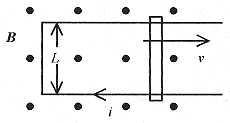
Consider a rectangular loop of wire being pulled through a magnetic field. The field is strength B, width of the loop L, length of the loop in the field x, and velocity with which the loop is being pulled through the field v. 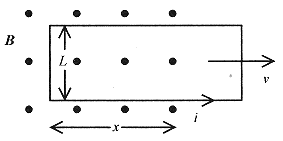 Fig. 37-7
Fig. 37-7
The total flux within the loop is Φ=BLx
As the loop is being pulled through the field there is a decrease in the number of lines of magnetic flux pointing out of the page. Therefore the induced current will be in a direction so as to create more flux out of the page. This requires a counterclockwise current around the loop. Interestingly this current produces forces on the sides of the loop. Apply the right-hand rule to the forces and note that the force on the bottom section of the wire is down, while the force on the top section of the wire is up. These two forces are equal and opposite and so add to zero. The force on the vertical section of the wire is to the left in opposition to the force producing the velocity. The force on the wire due to the induced current is BiL. The work performed by this force is (in analog with mechanics) force times distance or F.x=BiLx.
The power (again in analog with mechanics) is force times velocity or P=F.v=BiLv.
37-7 Pull a loop of width 0.20m, length 0.80m, and resistance 200Ωthrough a magnetic field of 0.40T at 0.20m/s. First find the induced emf and current. Then find the force necessary to pull the loop, the work performed, and the power, the rate of doing the work.
Solution: The induced emf is emf=-BLv =-0.40T.0.20m.0.20m/s=-1.6× 10-2 V.
The induced current (in the direction shown on Fig. 37-7) is ![]()
The force necessary to pull the wire out of the field is ![]()
The work performed in completely removing the loop is this force times the length of the loop.![]() The power delivered to the loop is P=F.v=6.4× 10-6 N.0.20m/s=1.3 × 10-6 W.
The power delivered to the loop is P=F.v=6.4× 10-6 N.0.20m/s=1.3 × 10-6 W.
<><><><><><><><><><><><>
37-8 A variation of the problem done above is one where the loop is replaced by a “U”-shaped piece of wire with a sliding piece along the arms of the “U.” Take a 0.60T field and a “U”shaped piece with width 0.30 m. The entire circuit has resistance of 20 Ω and the sliding bar is moving to the right at 6.0m/s.
Solution: The emf generated in the wire and moving rod is ![]()
The current in the loop is i = emf/R=1.1 V/20 Ω=0.054 A.
Fig. 37-8
When the bar is moving to the right, the number of lines of flux (amount) is increasing out of the page, so the current is clockwise so as to produce a field pointing into the page, i.e., a field opposing the increasing field, within the “loop” causing the current.
The work performed in moving the bar is ![]() Compare this with the Joule heat production H = i2R = (0.054 A)2 (20Ω)=0.058J.
Compare this with the Joule heat production H = i2R = (0.054 A)2 (20Ω)=0.058J.
<><><><><><><><><><><><>
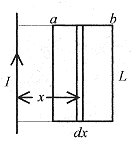
Calculation of the total flux through a rectangular frame placed with its long side parallel to a current-carrying wire is very helpful in understanding how to apply calculus to a physics problem.
Fig. 37-9
The geometry is shown in Fig. 37-9. What is desired is the total flux in the rectangle of width b-a and height L. The magnetic field at any point, x, a radial distance away from the current, is B= μ 0I/2πx.
The incremental flux over the strip of width dx is the flux at the point x times the incremental area ![]()
The total flux over the frame is the integral of dφ from a to b.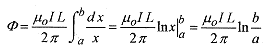
37-9 For the situation described above the current in the wire increases from zero to 20 A in 0.10s. Find the induced emf over a rectangle with a = 0.20cm, b = 0.60 cm, and L = 1.0 cm.
Solution: emf = ΔΦ/Δt and ΔΦ is the total flux, so ![]()
Time Varying Magnetic Fields.
There is another aspect of Faraday’s law associated with time varying magnetic fields. Place a conducting loop of wire in a magnetic field that varies with time and make the field increase out of the page so that the flux contained within the loop increases as shown in Fig. 37-10.
The emf generated in the loop is d Φ B/dt. In the wire there must be an electric field. In equations involving time varying magnetic fields the magnetic flux is often indicated with φB to avoid possible confusion with electric
Fig. 37-10
In this instance potential has no meaning (cannot be defined). Further, a physical wire is not necessary for the existence of the electric field caused by this changing flux. With the emf defined by the changing flux and the integral of the electric field, we can make the identification ![]() and remembering that ΦB = BA we have as a solution
and remembering that ΦB = BA we have as a solution![]()
37-10 Calculate how an electron placed at 0.30m radius would be accelerated in a changing magnetic field of 50 × 10-6 T/s.
Solution: The force on the electron would be eE = ma so ![]() Accelerating devices using this principle are called synchrotrons.
Accelerating devices using this principle are called synchrotrons.