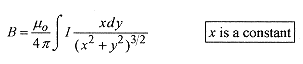Biot-Savart Law
Ampere’s law allows convenient calculation of the magnetic field surrounding a straight current-carrying wire. While the law is written in calculus terms the application is quite easy and involves little formal calculus for most problems. The Biot-Savart law is more general than Ampere’s law and allows calculation of the magnetic field in the vicinity of curved wires. Application of the Biot-Savart law involves considerable calculus. If you are interested only in the application of the formula for the magnetic field on the axis of a current carrying coil you may want to skip directly to the problems involved with these calculations. For the physics student with a reasonable background in calculus the Biot-Savart law is very good for learning how to interpret a differential law written in vector format.
The magnetic field due to a moving charge or a current is given by the Biot-Savart law.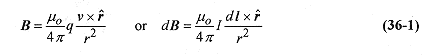 
These are vector equations. They allow calculation of the magnitude of the magnetic field and show the direction of that field. The equation on the left applies to moving charges and states that the magnetic field at ar has magnitude μ oqv/4πr2 and direction determined by the cross product of v and the unit vector . The equation on the right applies to currents in wires and states that the contribution of a length of wire dlto the magnetic field is μoIdl/4Πr2 and the direction is the direction determined by the cross product of dl and the unit vector . The following problem will help in visualizing the cross product.
36-1 Calculate the magnetic field a distance x radially out from a wire carrying a current I.
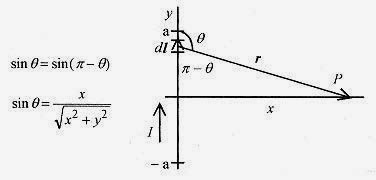
Solution: Figure 36-1 shows the geometry for calculating the magnetic field due to an element of current in the wire. Use the differential form of the Biot-Savart law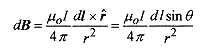 
and integrate. The vector dl is in the direction of 1, and the angle θ is the angle between the vectors dl and r. Cross dl (in the direction of I) with r to see thatB, at the point
P, is into the paper.
Fig. 36-1
Integrate from -a to a and then let a go to infinity to find the expression for the magnetic field due to a long straight wire. This specific integral was done in problem 25-5 in Chapter 25, Electric Field. Follow along the steps in problem 25-5 and work through this problem to confirm that the magnetic field outside a long currentcarrying wire is μoI/2πr, in conformity to Ampere’s law.
<><><><><><><><><><><><>
36-2 Calculate the magnetic field on the axis of a circular loop of current.
Fig. 36-2
Solution: The Biot-Savart law is most convenient for solving this problem. The cross product of dl and gives the direction of the field along the axis as being at right angles to rand in the plane defined by x and r. The loop of current and dl are in the y-z plane. Start with the law in differential form (equation 36-1) and write the x and y components of the field.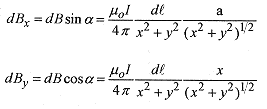
For every differential increment of length on the loop there is another differential increment of length across a diameter also producing a contribution to the field. Looking at the components of these fields, the x components add while the y components add to zero leaving only the x component that contributes to the resultant field. The field then reduces to the integral of the x component. 
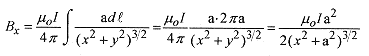At the center of the loop, x = 0 and the expression for the field reduces to 
For a coil of N loops the current is multiplied by N.
<><><><><><><><><><><><>
36-3 What is the magnetic field at the center of a circular coil of radius 5.0 × 10-2m carrying a current of 0.25 A and having 40 turns?
Solution: The field is perpendicular to the plane of the loop at the center and has value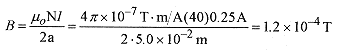 
<><><><><><><><><><><><>
36-4 For problem 36-3, what is the field 5.0 × 10-1m distance along the axis?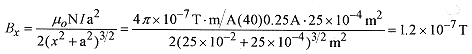 
36-5 A 3.0μC charge at x = 0, y = 1.0m has a velocity vx = 5.0 × 107 m/s. A -4.0μC charge at x = 2.0m, y = 0 has a velocity vy = 8.0 × 107 m/s. What is the magnetic field at the origin due to the motion of these charges?
Solution: For the first charge the vector points down (from the charge to the origin) and v × shows the magnetic field at the origin as into the page. The magnitude of this field is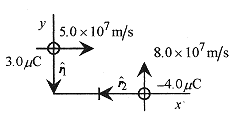
Fig. 36-3
For the second charge the vector points to the left (from the charge toward the origin) and v × is a vector pointing out of the page, but the negative charge makes the field point into the page. This magnetic field has magnitude 
The total magnetic field is 2.1 × 10-5 T directed into the page.
<><><><><><><><><><><><>
36-6 What is the magnetic field at the center of a semicircular piece of wire with radius 0.20m and carrying 150A of current?
Solution: Start with equation 36-1 in differential form, and referring to Fig. 36-4, form dl× that shows B into the page at the center of the semicircle. Integrating equation 36-1 produces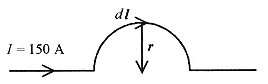 
Fig. 36-
The connecting wires are on radii out from the center of the semicircle, so dl× = 0 and they contribute no magnetic field at the center of the semicircle