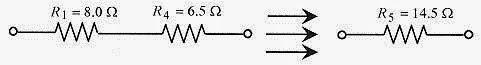Resistors in D.C. Circuits
In this chapter we will look first at series and parallel resistors, then at how series and parallel combinations are analyzed by writing successively simpler equivalent circuits.
Series Resistors
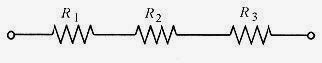
Take three resistors in series as shown in Fig. 30-1.
Fig. 30-1
If a voltage, V, is applied to this combination the current through each resistor is the same. The voltage statement for each of the resistors is V1 = IR1, V2 = IR2, V3 = IR3. The individual voltages across each resistor must add to the total so V = V1 + V2 + V3 = I(R1 + R2 + R3). The equivalent resistor for this combination is R = R1 + R2 + R3. Resistors in series add linearly.
30-1 What is the equivalent resistance of 3.0 Ω and 4.0 Ω resistors placed in series? What is the current in each resistor if 10 V is applied to the combination?
Solution: The equivalent resistance is Req = 3.0Ω+4.0Ω=7.0Ω. The current is the same in each resistor and has value I = V/Req = 10 V/7.0 Ω = 1.4 A.
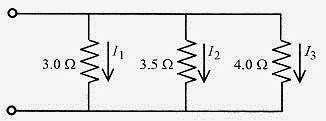
Parallel Resistors Place three resistors in parallel as shown in Fig. 30-2. If a voltage is applied to this combination, the voltage across each resistor is this applied voltage and the currents are determined by V = I1R1, V = I2R2, and V = I3R3. These currents must add up to the total current delivered to the combination.
Fig. 30-2
An equivalent resistor (for this combination) then would have the form![]()
Resistors in parallel add reciprocally.
30-2 What is the current in each resistor for a parallel combination of 3.0 Ω, 3.5 Ω, and 4.0 Ω resistors with 8.0V?
Fig. 30-3
Solution:
The total current delivered by the power source is I = 2.7A + 2.3A + 2.0A = 7.0A. Now check the total by using the equivalence 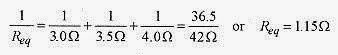
Using the equivalent resistance (1.15Ω) the total current is I = V/Req = 8.0 V/1.15Ω = 7.0A.
Series-Parallel Combinations
30-3 Calculate the equivalent resistance of the series-parallel combination shown in Fig. 30-4.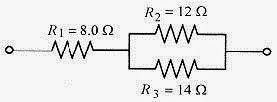
Fig. 30-4
Solution: The first step in this circuit analysis is to find the equivalent of the parallel combination.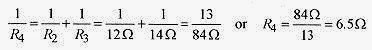
The circuit then becomes two series resistors and they add to a single resistor.
NOTE: The equivalent resistance of any two resistors is lower than either of the two alone.
Fig. 30-5
<><><><><><><><><><><><>
30-4 Apply 20 V across this network and find the voltage across and current through each resistor.
Solution: In order to find the current in a resistor in the original network, it is necessary to work back through the equivalent circuits. Start with the last circuit. Apply 20V to a 14.5Ω resistor for a current of I = V/R = 20 V/14.5Ω = 1.4 A. Working backwards, this current through R1 produces a voltage across this resistor of V1=(1.4A)R1=(1.4A)(8.0Ω)=11V. This current passes through the equivalent resistor R4 producing a voltage across it of V4=(1.4A)R4=(1.4 A)(6.5Ω) = 9.1 V. V1 and V4 add to 20 V, the total applied, to within round off error range. The 1.4A divides between R2 and R3. The voltage across R2 and R3 is V4 = 9.1 V so![]()
Again this total adds to 1.4A, within round off error. This analysis produces the voltage across and current through each resistor in the network. The numbers are internally consistent (the currents through R2 and R3 add up to the current through their equivalence) giving confidence in the calculation.
<><><><><><><><><><><><>
30-5 In the circuit of Fig. 30-6 calculate the voltage across, current through, and power requirements of the 8.0Ω resistor when 15V is applied to the network.
Fig. 30-6
Solution: The first step in the analysis is to find the equivalent of R2 and R3.![]()
A similar analysis on R4 and R5 yields ![]() Now the equivalent can be drawn.
Now the equivalent can be drawn. 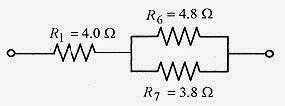
Fig. 30-7
The parallel combination of R6 and R7 produces another equivalent. 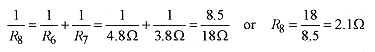
Fig. 30-8
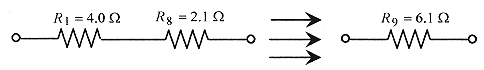
The total current is I =V/R9 = 15V/6.1Ω=2.46A.
This current through the 2.1Ω resistor produces V8=(2.46A)R8=(2.46A)(2.1Ω)=5.16V.
Look back through the equivalent circuits and notice that this voltage is across R8, R6, and R2.
The current through R2 then is I2=V8/R2=V2/R2=5.16V/8.0Ω=0.64A.
The power dissipated in this resistor is I2R=(0.64A)2(8.0Ω)=3.3W.
<><><><><><><><><><><><>
The general procedure for circuit analysis is to look at the circuit and find parts of it that can be replaced with simpler equivalents and just keep applying the process until the circuit is reduced to one resistor, perform V = IR on that resistor, and move back through the equivalents to obtain the desired information.