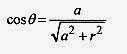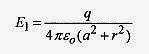The Electric Field
The electric field is defined as the force per unit charge E = F/qo and as such is a vector. Electric fields can be superposed added as vectors. The units of the electric field are N/C or Volt/meter. In terms of charges the electric field has magnitude
and direction, the direction of a unit positive charge placed at r.
Discrete Charges
A classic problem that illustrates the vector nature of electric fields is the calculation of the E field on the axis of a dipole.
25 1 An electric dipole is depicted as equal and opposite charges separated by a distance 2a as shown in Fig. 25 1. Find the electric field at a distance r along the bisector of the center line of the charges.
Fig. 25 1
Solution: First draw the diagram and note the direction of the electric field vectors. The horizontal components of E1 and E2 add to zero. The vertical component is made up of two vectors of equal magnitude, E1cosθ. The magnitude of the vertical vector is 2E1cosθ
From the geometry,
and
so
This is the magnitude of the electric vector. The direction of the vector is down or parallel to a line drawn from +q to q. If r is large compared to a (often the case) then 
and for large distances the field falls off as 1/r3. The product 2aq is called the dipole moment p = 2aq. It is interesting to note that for a point charge the E field is radial (in or out) and falls off as 1/r2 while for the dipole E is tangential to the radius and falls off as 1/r3.
<><><><><><><><><><><><>
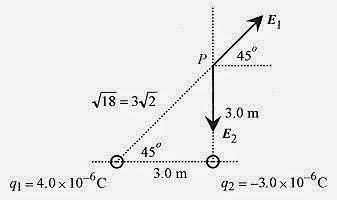
25 2 Calculate the electric field due to two charges, q1 = 4.0 x 10 6C and q2 = 3.0 x 10 6C, separated by 3.0 m and at a point 3.0 m opposite q2 as shown in Fig. 25 2.
Fig. 25 2
Solution: The electric field due to the first charge is
The electric field due to the second charge is 
The direction of the fields due to these two charges is shown on the diagram. The vectors are written in component form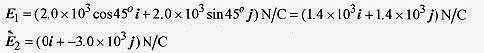 
with resultant ER = (1.4 x 103i+ 1.6 x103j)N/C
As an exercise verify that in number plus angle form this vector is . 
<><><><><><><><><><><><>
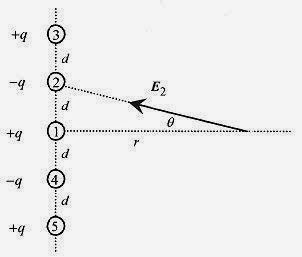
25 3 Find an expression for the electric field due to a collection of charges of equal magnitude all placed in a line and separated by a distance d. The charges have alternate signs, and the field to be calculated is the field on a line normal to the line of charges and opposite the central positive charge. Additionally, look for approximate expressions for long distances away from the line of charges.
Fig. 25 3
Solution: The electric fields are labeled to correspond with the charges. The fields at a point r distance away from the line of charge are: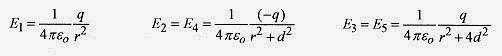
Look at the geometry associated with E2 and notice that this vector can be written in terms of a horizontal and a vertical component.
The vector E4 also has vertical and horizontal components. The vertical components of E2 and E4 add to zero while the horizontal components add along r. The same is true of vectors E3 and E5. The horizontal component of E2 is E2 times the cosine of the angle θ, thus the contribution of E2 and E4 is two times the magnitude of E2 times the cosine of the angle θ
E2 and E4 contribute 
pointed in along r.
Similarly E3 and E5 contribute
also pointed out along r
Combining the contributions from all the charges 
If r is comparable to d then this formula is appropriate. However if r is large compared to d, then the r terms predominate in the denominators and the expression is approximately
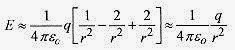This formula shows that at long distances the array looks like one positive charge.
Linear Charge.
So far we have looked at the electric fields that result from discrete charges. If the charges are spread out, such as over a surface, then the definition of electric field has to be modified to a calculus definition where the contribution of each element of charge to the total field is summed.
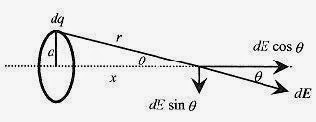
25 4 A problem that illustrates this is the calculation of the electric field on the axis of a ring of positive charge. Set up the problem as shown in Fig. 25 4.
Solution: Each element of charge dq produces an element of electric field dE at a point on the bisector of a diameter of the ring. This dE has components dE cosθ along the direction of the bisector and dE sin θ perpendicular to the bisector. An element of dq at one spot on the ring produces dE sin θ, and across the diameter of the ring a similar element (of dq) produces a dE sin θ component that cancels this one. Because of this symmetry the dE sin θ components add to zero.
Fig. 25 4
The magnitude of the electric field due to each element of charge can be written as 
but it is dE cosθ which ultimately produces the field pointing outward from the center of the ring.
From the geometry ,
so the differential electric field component is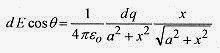
This expression can be summed (integrated) without the need for formal integration. The left side is just the total electric field, the sum of the dE cosθ contributions. The right side is the sum of all the elements of dq, which is just q, so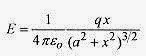
Notice that at x = 0, E = 0, as it should and that for , 
again as it should. Far away the ring of charge should look like a point.
Surface Charge
25 5 Calculate the electric field vertically out from a uniformly charged disk of radius R and charge density (charge/area) ƒÐ.
Solution: The calculus definition of the electric field is that each element of charge produces an element of electric field according to equation 25 4 where r is the distance from the element to the point where dE is calculated. Because of the symmetry of the problem the dE is produced by a dq in the form of a ring of charge of radius r and width dr. Refer to Fig. 25 5 and note that the dE produced by this ring of charge is a cone with horizontal components adding to zero. A piece of the ring producing a piece of dE has a corresponding piece across a diameter. The horizontal components of these corresponding pieces add to zero. The vertical
component is dE cosθ with cosθ determined from the geometry as Summing the contributions over r produces the electric field pointing away (and perpendicular to) the charged disk. The differential element of charge is dq = (2πrdr)ƒÐ (2πrdr is the differential element of area), thus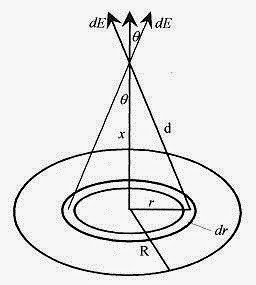
Fig. 25 5
Remember the integral is over r and not x. Make a change of variable with u = x2 + r2 and du = 2rdr. The integral transforms according to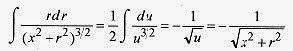
Changing the sign and switching the limits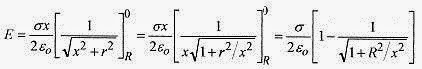
When R is large compared to x, the bracket approaches one and the electric field is
Applications
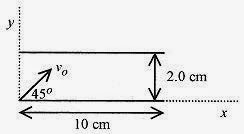
25 6 Consider an electron ballistics problem where an electron is projected between two plates (as shown in Fig. 25 6) at an initial velocity of 6.0 x 106 m/s and angle of 45‹. The electric field is 2.0 x 103N/C and directed up. The length of the plates is 10 cm and separation 2.0 cm. Will the electron strike the plates? And if so, where?
Fig. 25 6
Solution: This is a projectile problem. Look through Chapter 4, Projectile Motion, and refresh your memory on the general approach to projectile motion problems before continuing with this problem. The electronic charge and mass are given on the constants page.
First calculate the acceleration. The force on the electron comes from the electric field, F = eE, and this force makes the electron accelerate leading to a = eE/m. Using the values for e and m for the electron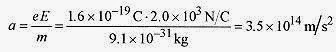 
The electric field is directed up, which would be the direction of acceleration of a positive charge. For the negatively charged electron the acceleration is down. Using this logic argument to determine the direction of the acceleration is easier than carrying minus signs throughout the problem.
The most convenient origin for the coordinate system is the edge of the plate where the initial velocity and direction are known with x positive to the right and y positive up. Now write the six equations describing the motion.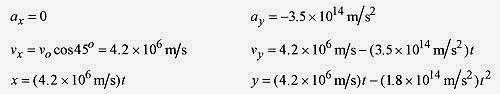 
To determine if the electron hits the upper plate, set y = 2.0 x 10 2 m and solve for t. Without units
and 
The smallest time is taken as this corresponds to the electron striking the top plate. If the solution for t had contained an imaginary number, this would have indicated that the electron would never reach the top plate.
Now with this time calculate the position in x where the electron strikes the plate.
Another way to solve the problem is to go back to the six equations describing the motion and solve for y as a function of x. First solve the x equation for t and substitute this expression for t into the y equation to obtain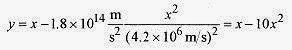
When y = 0.020 this equation becomes 10×2 x + 0.020 = 0 with solution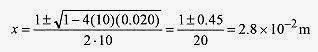
Here again be sure to take the value of x corresponding to the smallest positive time.
Dipoles
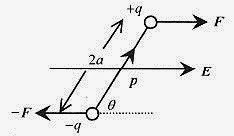
A dipole placed in an electric field experiences a torque. The dipole moment, defined earlier as p = 2aq, can be expanded to define the dipole moment vector of magnitude 2aq pointing from q to +q. The force on each charge is qE, and they add to zero so the dipole does not translate.
Fig. 25 7
It does, however, rotate due to the torque of magnitude 2aF sin θ. When θ= 0 and the dipole is lined up with the field, the torque is zero. But when θ= 90‹ the torque is 2aF. Multiply the torque by q/q to obtain
which is a cross product, or 
25 7 A dipole with charge 6.0 x 10 6C and separation 4.0 x 10 3 m is immersed in an E field of 3.0 x 106N/C. Calculate the maximum torque.
Solution: 
<><><><><><><><><><><><>
If a dipole is subjected to an E field and the dipole is rotated by that electric field, then work is done on the dipole. The work done by the field must be
Be careful with the signs and angles.
25 8 Take the original position θ0 = 70‹, and the final position θ1 = 20‹ and calculate the work performed on the dipole and the energy stored in the system.
Solution: 
The electric field has done 0.086J of work on the dipole, and this is the amount of energy stored in the dipolefield system.