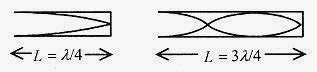Standing Waves (Strings and Pipes)
A string stretched between two fixed end points appears to vibrate in a sinusoidal manner when plucked. Blowing across the top of a partially filled bottle of liquid produces a specific frequency sound with the frequency depending on the length of the air column above the liquid. The wave on the string is transverse. the elements of the string move up and down while the wave moves back and forth along the string. The wave in the partially filled bottle is longitudinal: pulses move up and down the column of air in the same direction as the wave. These two very different waves can be analyzed in a similar fashion.
Waves on Strings
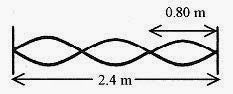
When a stretched string is plucked, the pattern appears as a sinusoidal standing wave. The wave pattern is sinusoidal because the only solution to the wave equation (previous chapter) is a sinusoidal function. The wavelength of the waves is determined by the length between the fixed end points of the string. A sine wave on a string reflected at a fixed boundary produces a standing wave where the fixed length of the string is an integral number of half wavelengths. Figure 22 1 depicts the sinusoidal standing waves on strings as observed in laboratory experiments with vibrating strings.
Fig. 22 1
Positions on the string where there is no motion are called nodes and positions where there is maximum motion are called antinodes or loops. The standing wave on the string is produced by two waves, one moving to the right 
and another moving to the left 
both with the same wavelength and period.
The standing wave is the sum of these two waves
Using two identities from the Introduction, Mathematical Background, the sum is
The cos2 πt/T term gives the time dependent motion of a piece of the string at a specific value of x. The (2A)sin2 πx/λ term is the amplitude of the up and down motion of the piece of the string at the specific value of x.
Where x is equal to 0 and λ/2, the sin 2 πx/λ term is zero, and the string doesn’t move. The allowed wavelengths are determined by requiring x/λ to equal 1/2, 1, 3/2 c, that is, where the sine function is equal to zero. This corresponds to x = λ/2, λ, 3λ/2 c.
For a string fixed at end points L distance apart, the allowed wavelengths are then L = nλ/2(n = 1,2,3 c). Indexing λ for the allowed wavelengths
The allowed frequencies for this stretched string are from v = λf or v = λnfn, so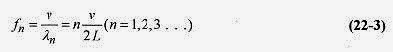
Remember that for the stretched string, v is a constant equal to .
The first frequency (longest wavelength) is called the fundamental and the next frequency the second harmonic or first overtone with subsequent frequencies labeled in a similar manner.
When a stringed musical instrument is plucked, all of these allowed frequencies are prevalent though the overtones are of successively lower amplitude. The difference in sound of stringed instruments plucked at the same fundamental frequency is due to the design of the instrument to enhance or suppress certain of these overtones.
22 1 The third harmonic is excited on a 2.4m length of stretched string. What are the positions of the loops and nodes?
Solution: To produce the third harmonic there are two nodes at 0.80 and 1.6m so as to divide the string into three segments. The loops are half way between the nodes at 0.40, 1.2, and 2.0m.
Fig. 22 2
<><><><><><><><><><><><>
22 2 An 0.040kg string 0.80m long is stretched and vibrated in a fundamental mode with a frequency of 40 Hz. What is the speed (of propagation) of the wave and the tension in the string?
Solution: The relationship v = λ.f is used to calculate the speed of the wave. Since the string is vibrating in fundamental mode, the wavelength is 1.6m (see Fig. 22 1), so 
Having determined the velocity from this basic relation, the tension can be determined from . The linear density of the string is μ = 0.040 kg/0.80m = 0.050 kg/m so 
<><><><><><><><><><><><>
22 3 For the situation described in problem 22 2, add that the amplitude (maximum displacement from the center line of the string) is 2.0mm. What is the displacement at 0.20, 0.40, and 0.60mm, and the time for one of these oscillations?
Solution: The oscillation is described in both space and time by equation 22 1 at x = 0.20m. The equation is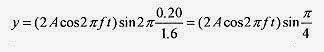
The sin π/4= 0.71 term specifies the fraction of the 2.0mm (amplitude) of the oscillation at
x = 0.20m. This maximum displacement is 1.4mm. This is the displacement measured from the center line of the string. The height of the envelope or total (up and down) excursion of the piece of string is 2.8mm.
At x = 0.40mm the sine term becomes sin π/2 = 1 and the total excursion is 4.0mm.
Because of symmetry the maximum excursion at 0.60m is the same as at 0.20m.
The stretched string goes up and down together, that is, all points on the string are vibrating with the same frequency of 40 Hz, so the time for one oscillation is 1/40s= 0.025s.
<><><><><><><><><><><><>
22-4 One string on a cello is tightened to produce concert A (440 Hz) when the string is not touched (shortened by placing a finger on it). The length of the vibrating string is 60cm and mass is 2.0gm. How much must the player shorten the string to play a 660 Hz note?
Solution: The tension (velocity) is adjusted so that the frequency is 440 Hz for a wavelength of 1.2m. The velocity is 
If the tension is not changed, the velocity is not changed so the wavelength to produce 660 Hz is
or 
The length of the string to produce this frequency is one half of a wavelength or 40cm. The player needs to shorten the string by 20cm (from 60cm to 40cm). This is done by pressing the string onto the fingerboard.
Waves in Pipes
If a longitudinal wave is propagated down a gas filled tube, the wave will be reflected at a closed end in much the same manner as the wave on a string is reflected at a rigid boundary thereby setting up standing waves in the tube. Reflection at this end implies no motion and a displacement node. If the end of the pipe is open, then this end is a displacement antinode. The air is free to move at an open end. Similarly a string with an end free to vibrate has an antinode at the free end.
The simplest example of a standing wave in a tube is an organ pipe. Air is blown across the open end of the organ pipe. The opposite end can be closed or open.
Look first at a closed (at one end) pipe. The open end is a loop (displacement antinode)
Fig 22 3
and the closed end a displacement node, so the first fundamental frequency has wavelength 4L (see Fig. 22 3) The next allowed wave, the second harmonic, as shown in Fig. 22 3, is 4L/3. The general expression for the wavelengths is 
and
Next look at an open (both ends) pipe. Both ends have to be loops (displacement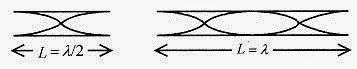
Fig. 22 4
antinodes), and the simplest situation is shown in Fig. 22 4. The second harmonic is the next simplest situation (also Fig. 22 4) with the general expression for the wavelength 
and frequency 
22 5 Standing waves are excited in a 1.0m long pipe open at one end, closed at the other. Take the speed of the waves as 340 m/s and calculate the frequency for the fundamental and the first harmonic.
Solution: The wavelength is determined by the geometry, so look to Fig. 22 3 where the left diagram shows the fundamental wave. If the pipe is 1.0m then the wavelength is 4.0m and the frequency is 
The next wavelength is shown in the right diagram and requires a π = (4/3) m and 
<><><><><><><><><><><><>
22 6 What is the lowest possible frequency of a 5.0m long organ pipe?
Solution: The lowest frequency corresponds to the longest wavelength, and the longest wavelength is for a closed at one end open at the other pipe (see Figs. 22 3 and 22 4). In this situation the wavelength is 20m corresponding to a frequency of 
This frequency is below what most people can hear.
<><><><><><><><><><><><>
22 7 An open at both ends organ pipe has two successive harmonics of 567 Hz and 850 Hz. What is the length of the pipe? Take the speed as 340m/s.
Solution: For an open at both ends pipe the wavelengths are given by equation 22 6. For successive harmonics (n and n +1) two equations can be written in the form v = λf.
and 
or
Refer to Fig. 22 4. These frequencies correspond to the second and third harmonics. The lowest frequency (567 Hz) corresponds to the longest wavelength and using v =λf
The pipe then is 0.60m long.