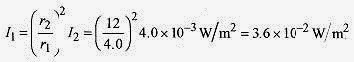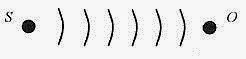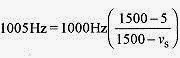Sound.
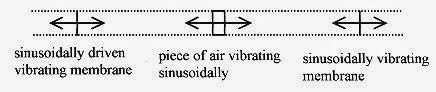
Longitudinal waves in air is called sound. The simplest physical picture of sound is a thin membrane vibrating (being driven) sinusoidally with the vibrations being transmitted through the air to another membrane that is set into sinusoidal motion.
Fig. 23 1
Picture the source as an electrically driven speaker and the detector as your ear that detects the vibrations and transmits their frequency and intensity to your brain.
If the sending membrane executes one sinusoidal oscillation, a region of high pressure followed by a region of low pressure, is propagated through the air to the sensor which executes a similar sinusoidal oscillation as the variations in pressure pass by. (Regions of low pressure are called rarefactions.) Remember that the individual air molecules or slices of air between source and detector move only about an equilibrium position while the pressure rarefaction pulse moves from source to detector.
Take the source as vibrating according to
Remember that y is in the same direction as x, and y represents the displacement of a narrow slice of air while x represents the motion of the pulse from source to detector. Go over the picture of sound in your mind as a pressure rarefaction pulse traveling from source to detector (see Fig. 231) until this last sentence becomes clear to you. When you understand this statement you have a good physical image of sound.
The pressure maximum is coincident with the maximum rate of change of y with respect to x.
The pressure variation, which is proportional to (Ýy / Ýx), is a cosine function. The slope of y versus x is a maximum (positive) at x = 0, and maximum (negative) at π/2 . Pressure, (Ýy / Ýx) is 90‹ out of phase with displacement.
The ear is very sensitive to pressure variations, capable of detecting pressures of 10 5 Pa which is 10 10 of an atmosphere!
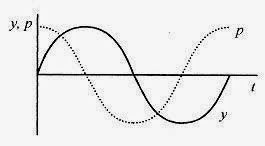
23 1 At some arbitrary position x graph displacement and pressure as a function of time on the same axis and explain the relationship.
Solution:
Fig. 23 2
Pick a point x where the displacement versus time is a sine curve (equation 23 1). The pressure curve (equation 23 2) is a cosine curve. Maximum pressure occurs when y is moving to the right with maximum speed. This corresponds to maximum positive slope or the point t = 0 on the graph. Minimum pressure (a rarefaction) occurs 180‹ later where the slope of y versus t has its largest negative value.
Intensity and the Inverse Square Law
Sound waves transport energy. Most sound sources radiate spherically symmetric patterns while most detectors intercept only a certain portion (solid angle) of the radiated energy. Therefore in sound measurements it is convenient to specify intensity, the power per unit area, rather than total power.
The power transmitted by a wave on a string is proportional to the square of the amplitude. With techniques similar to those used to determine the power transmitted by a wave on a string, the intensity of a sound wave is
where p is the density of air.
For a source of sound, the total power is the intensity times the spherical area corresponding to the point (at a radius out from the source) where the intensity is measured. 
23 2 What is the total power output of a source with intensity 0.050W/m2 at a distance of 3.0m from the source?
Solution: The total power is the intensity times the area of the sphere with radius 3.0m.
Fig. 23 3
<><><><><><><><><><><><>
23 3 What is the intensity of a 4.0W source at 2.0m?
Solution: The power is P = 4 π2 I so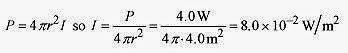
<><><><><><><><><><><><>
Consider a spherically symmetric source and intensity measurements on two different concentric spheres. The total power is the same so the power collected over one sphere is the same as the power collected over any other sphere. In equation form this statement is
leading to
which is the inverse square law.
23 4 If the intensity from a source is 4.0 ~ 10 3 W/m2 at 12m, what will the intensity be at 4.0m?
Solution: Assume a spherically symmetric radiator and equate the powers at the two radii.
P1 = P2 requires
Be careful on problems like this that you don’t get the ratio upside down. Develop the habit of checking for the obvious. When you get closer to a source the intensity increases and vice versa.
<><><><><><><><><><><><>
23 5 How far away can a 50 W tornado siren be heard? Assume the minimum detectable intensity is 5.0 ~ 10 6 W/m2
Solution: The power is P = 4π2 I so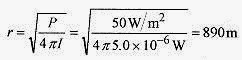 
The Decibel
The human ear is responsive to sound intensities over a wide dynamic range, 1010 or more in intensity levels. A person’s perception of gloudnessh is that increasing intensity by a factor of 10 approximately doubles the perceived gloudness.h This property of the human ear suggests that sound intensity, or level, should be measured with a logarithmic scale. The logarithmic decibel scale for measuring loudness is defined as
where Io = 1.0 ~ 10 12 W/m2 is the approximate threshold for human hearing.
23 6 Most people experience pain at sound levels above 100dB. What is the intensity associated with 100dB?
Solution: Equation 23 6 needs to be solved for I, and this requires switching from a logarithmic to an exponential equation. See the Introduction, Mathematical Background for a review of logarithmic and exponential equations.
First write ƒÀ/10=log(I/Io) and remember that x=logy has the solution y=10x. The use of glogh implies base 10.
Switch from a logarithmic equation to an exponential one and write I/Io = 10ƒÀ/10.
For ƒÀ = 100,
Practice switching logarithmic equations to exponential equations and vice versa and solving for the various variables. This is not a common algebraic operation, and you need sufficient practice to develop proficiency with exponents and logarithms.
<><><><><><><><><><><><>
23 7 What is the dB loss when you double your distance from a spherically symmetric constantoutput power source?
Solution: Since the source is constant power output and spherically symmetric the inverse square law applies. Take r1 and r2 = 2r1 and apply power equals power.
and
The dB levels at r1 and r2 are 
and 
and the difference is
Doubling the distance from the source results in a 6.0 dB loss.
The Doppler Effect
The Doppler effect is the apparent increase or decrease in frequency of a source when the source and observer are moving toward or away from one other.
Fig. 23 4
First consider a stationary source and a moving observer. In Fig. 23 4 pressure pulses are depicted as equally spaced and moving radially out from the source. An observer at rest would observe vt/λ waves passing in a time t (vt is the length of the collection of waves or gwave trainh traveling from source to observer and λ is the wavelength, so the quotient is the number of waves). The number of waves divided by the time is the frequency f = vt/μt resulting in v = μf.
If the observer is moving toward the source with a velocity vo he will intercept vot/μ more waves, and the apparent frequency is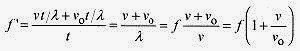 
If the observer is moving away from the source, the sign of vot/μ is negative. The apparent frequency then corresponds to the negative sign in equation (23 8). 
23 8 What is the apparent frequency of a siren emitting a 300 Hz sinusoidal signal that you are approaching at 30m/s?
Solution: Use equation 23 8 with the plus sign because you are approaching the source and intercepting more waves than if you were stationary. 
<><><><><><><><><><><><>
23 9 What is the apparent frequency of the siren in problem 23 8 after you have passed it and are moving away?
Solution: When you are moving away the frequency is lower because you are intercepting fewer wave fronts than if you were stationary so use the minus sign in equation 23 7.
<><><><><><><><><><><><>
Now consider a moving source and a stationary observer. Figure 23 5 depicts the pressure pulses as packed closer together in the direction the source is moving. This effectively shortens the wavelength to a stationary observer. 
Fig. 23 5
The effective wavelength is λ’= (v vs)/f, and the apparent frequency is 
For a source moving away from an observer the effective wavelength is (v + vs)/f, so combining 
The two expressions for source and observer moving (equations 23 7 and 23 8) can be combined 
The way to keep the signs straight is to remember that on approach the frequency is increased and on departure the frequency is decreased.
23 10 A railroad train traveling at 30m/s emits a note at 400 Hz. What frequency is heard by an observer a) on the train, b) standing beside the track, and c) on another train approaching at 35m/s?
Solution: a) On the train the observer hears the 400 Hz.
b) Beside the track with the train approaching the observer hears a higher frequency 
And with the train moving away the observer hears a lower frequency
c) On the other train the observer hears a higher frequency due to the source approaching, (v vs) term, and observer approaching, (v + vo) term
<><><><><><><><><><><><>
23 11 Submarines use 1000 Hz signals to avoid collisions. A submarine traveling at 5.0 m/s observes another submarine on the same course behind it and detects the collision avoidance signal from this submarine at 1005Hz. What is the speed of the other submarine? The velocity of sound in water is 1500m/s.
Solution: This is a Doppler effect problem with both source and observer moving. The hard part of the problem is getting the signs correct. First note that the frequency is higher than emitted so the submarines are closing. If the observer were stationary the formula would be
f’=f[v/(v vs)]. If the observer were moving away from a stationary source the formula would be f’= f[(v vo)/v]. So combining, the correct formula is 
and putting in the numbers 
or vs=12m/s.