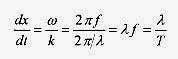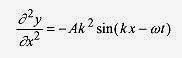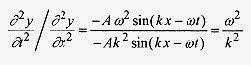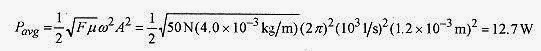Mechanical Waves
Mechanical waves travel in a medium. The medium and the mechanism for producing the wave determine its properties. There are two types of mechanical waves.
Transverse waves are those where the mechanical motion is perpendicular to the direction of the wave. A wave snapped on a rope in tension is a transverse wave: pieces of the rope go up and down, and the wave travels along the rope. Waves on water are transverse: the water goes up and down, and the wave travels along the surface of the water.
Longitudinal waves are waves in the direction of the motion. An audio loudspeaker creates a varying pressure wave by physically moving the (column of) air between the speaker and your ear.
Waves move with a speed determined primarily by the medium. Waves also transport energy.
Transverse Waves on a String.
First consider the periodic sinusoidal waves that propagate on a stretched string. If we took a gsnapshoth (Fig. 21 1) of the wave we would see a sine wave with amplitude, the maximum displacement from equilibrium, and wavelength, the length for one complete cycle, or from crest to crest or trough to trough. The velocity of the wave is the wavelength divided by the time for the wave to pass a point on the string.
Fig. 21 1
This time for the wave to pass a point on the string, or for a piece of the string to execute one sine wave, is called the period. The reciprocal of the period is called the frequency.
21 1 The end of a stretched rope is being vibrated up and down at 120Hz. A gsnapshoth of the wave indicates a wavelength of 0.50m. What is the speed of the wave?
Solution: The wave has velocity given by ν = λ. = 0.50m(120Hz) = 60m/s.
<><><><><><><><><><><><>
Take a stretched string being vibrated with an amplitude A at a frequency of 10 Hz, or period 0.10s. In this discussion do not be concerned with how the stretched string is terminated. The displacement of the end of the string is![]()
The displacement is a sine function, and sine functions are periodic in 2π, so when t has gone from zero to 0.10s, the time for one period, the oscillation has gone through one (2π) cycle. One In cycle is shown in Fig. 21 1. Writing the argument of the sine function as 2π.t is more compact, and replacing 2π. by ω is even more convenient.
From the basic definition of ν = x/t, the time for a wave to move a distance is x/v.
The displacement of a piece of the rope at any distance along the rope and at any time is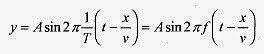
The velocity ν is also equal to λ. so another often convenient form is
In many problems the wave number, k = 2π/λ, is often used and combining with ω = 2 πf![]()
This equation describes the wave on the string at any particular time. It also describes the motion of a piece of the string at any position on the string. The minus sign is for a wave moving in the positive direction. A plus sign would be for a wave moving in the negative direction.
21 2 A sinusoidal wave is propagated on a string with amplitude 0.050m, wavelength 0.20m,
and frequency of 40 Hz. Write an expression for the displacement of the string as a function of the time and position on the string.
Solution: The period of the wave is 1/40 s= 0.025s. The displacement is according to equation 21 2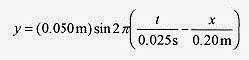
<><><><><><><><><><><><>
21 3 For the situation described in problem 21 2, what is the expression for the time variation of a point on the string at x = 0.010m?
Solution: The expression for y with x = 0.10m is 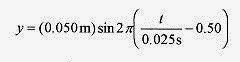
If at t = 0, a wave is initiated from the point x = 0 in the positive direction, then according to this expression, it takes t =(0.025s)(0.50)= 0.0125s for the wave to reach 0.010m. After this 0.0125s time interval, the point x = 0.010m oscillates in a sinusoidal manner. Look back at the numbers for wavelength and period in problem 21 2 and notice that it takes 1/2 of a period for the wave to travel 1/2 of a wavelength.
<><><><><><><><><><><><>
21 4 For the situation of problem 21 2, what is the expression for the space variation of the wave at t = 0.25s?
<><><><><><><><><><><><>
There are two speeds associated with this description of a wave on a string. The speed that the wave moves along the string is called the phase velocity. This is thevelocity of a crest of the wave as it moves in the positive direction.
For a wave traveling at constant speed, the displacement must be a constant as a function of space and time, so sin(ωt kx) must equal a constant, and therefore ωt kx must equal a constant. Taking the total derivative of ωt kx yields the phase velocity dx/dt.
The speed of the wave along the string is the length of the wave divided by the time for one oscillation.
The transverse velocity is the up and down velocity of a piece of the string. This is Ýy/Ýt. We use the Ý notation rather than the d because it is possible to take derivatives of y with respect to both x and t. For a sinusoidal wave described by equation 21 3 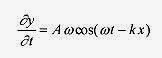
This derivative shows that the up and down motion of a piece of the string is sinusoidal. The maximum velocity (of a piece of the string) occurs at its equilibrium position, where
cos(ω kx) = 1, and is equal to Aω.
Wave Equation
If the equation for the wave y = Asin(ωt kx) is differentiated twice with respect to t and twice with respect to x a very interesting result is obtained: 
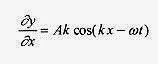
But ω/k is ν, the velocity, so 
which is called the wave equation, a most important equation in physics. Any function of x and t that satisfies this relation propagates in the positive x direction with velocity v. This analysis is equally valid with longitudinal waves (sound) with the added complication that y describes the displacement of the particle from its equilibrium but in the direction of the wave.
Transverse Wave Speed
The speed of a transverse wave on a string depends on the tension and the mass per unit length. Common experience is that a wave gsnappedh on a string moves faster when the string is under greater tension and slower when a heavier (mass per unit length) string is used.
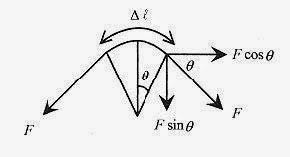
Look at the crest of the wave as forming part of a circle and follow this crest as it propagates along the string. Take the arc of the circle as length Δl and the string of mass per unit length μ. Thus the mass of the length Δl is μΔl. Next look at the forces on thispiece of string. The tension in the string, F, has the components as shown in Fig. 21 2.
Fig. 21 2
The force, on the piece of string Δl, directed toward the center of the circle is 2Fsin ƒÆ.
This center directed force is the centripetal force![]()
Set this center directed force equal to![]()
21 5 What is the speed and wavelength of a 20Hz frequency wave on a string of 0.20kg/m under tension of 80 N?
Solution: The velocity of the wave is![]()
Use the basic relation ν = λ. to find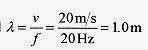
Power
When a stretched string is displaced (plucked), a pulse travels along the string. There is a force associated with this displacement. This force acting on an element of the string to produce the displacement and return to equilibrium is the work performed on the element of string or the energy associated with the pulse. This energy per time is the power transported along the string. 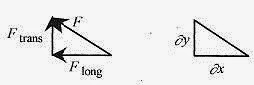
Fig. 21 3
In a stretched string the tension, or force, has longitudinal and transverse components. The components of the force are proportional to the slope as shown in Fig. 21 3. The slope is written in the partial derivative notation. (Remember y is a function of both x and t.)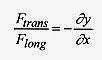
For small displacements the longitudinal component of the tension is the static tension in the string
(Flong, = F, or small displacements). While the pulse is moving along the string the transverse force is always opposite the slope of the pulse. The transverse force that moves the pulse is opposite (in sign) to the displacement. ![]()
The power, again from mechanics, is this transverse force times the transverse velocity, or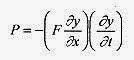
The average value of the cos2 function is 1/2, w=νk, and ν= F/μ, so
21 6 A steel wire of length 1.0m and mass 4.0gm is stretched with a force of 50N. Waves of
amplitude 1.2 x 10 3 m and frequency 1000 Hz are traveling on the wire. What is the average power of these waves?
<><><><><><><><><><><><>
21 7 What happens to the power if the frequency is increased by a factor of 10?
Solution: Since the frequency appears in the formula as the square, the power increases by a factor of one hundred.