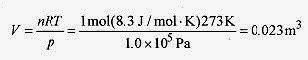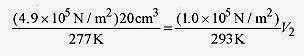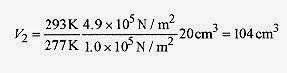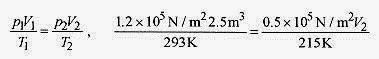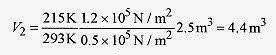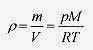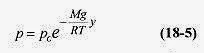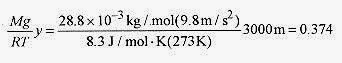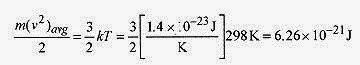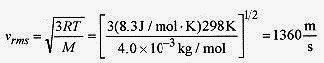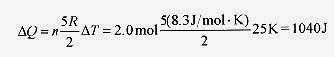Kinetics and The Gas Laws
A complete description of a gas is given by the pressure, volume, temperature, and amount (total mass or number of moles) of the gas. What is called the equation of state (equation 18 1) describes the relation between p, V, T and m or n. These four properties completely describe a gas just as position, velocity, and acceleration completely describe the motion of a particle.
Early experiments on gases produced Boyle’s law, pV = constant (at constant temperature), Charles’ law, p = constant . T (at constant volume), and finally, the ideal gas equation of state pV = nRT with n the number of moles and R the gas constant equal to 2.0cal / mol.K or 8.3 J / mol.K. A mole of a gas contains a specific number of molecules. The number of molecules per mole is Avogadro’s number, NA = 6.0x1023 molecule / mole. The mass of a mole of gas depends on the atomic weight of the gas. The mole and Avogadro’s number are defined as the number of carbon 12 atoms in 12g of carbon 12. For helium (atomic weight 4.0) one mole of the gas contains Avogadro’s number of helium atoms and has mass of 4.0g.
The ideal gas law not only describes the p, V, T relationship but allows that for the same amount of gas the relationship between two different states is
allowing a large number of problems to be solved. In applying this formula, Kelvin temperatures must be used throughout, and the pressure and volume must be written in the same units.
18-1 What is the volume of a mole of ideal gas at standard temperature and pressure (STP)?
Solution: Standard temperature is 0‹C = 273K. Standard pressure is latm = 1.0 x 105 Pa. From the ideal gas law
This is a 23 liter container or a box 0.28m on a side Remember that a Pascal is a N/m2.
<><><><><><><><><><><><>
18 2 An air bubble at the bottom of a lake has a volume of 20cm3, pressure of 4.9Pa, and temperature 4‹C. The bubble rises to the surface where the temperature is 20‹C and the pressure 1.0 Pa. Find the volume as the bubble reaches the surface. Also find the number of moles of the gas.
Fig. 18 1
remembering that T is written in kelvin.
or
To find the number of moles use pV/T = nR and the given conditions at the bottom of the lake.
<><><><><><><><><><><><>
18 3 A weather balloon is inflated with helium at just over atmospheric pressure of 1.2x105 Pa, temperature 20‹C, and volume 2.5m3. When the balloon rises to where the pressure is 0.50x105 Pa and temperature is 58‹C, what is the volume?
Solution: Using
or
As an exercise find the number of moles of this gas.
Variation of Pressure with Elevation.
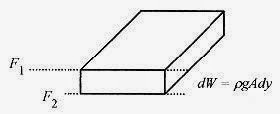
Consider a slab of air in a column of air and note that the increase in force between the top and bottom of the slab is due to the weight of the slab. Envision this as holding your hand horizontally at two different heights. Higher up in the column of air there is less air above your hand and thus a lower pressure.
Fig. 18 2
Writing this difference with differentials, F2 = F1 + dW
Since p = F / A and dW = pgAdy = pgdV, this statement can be written as
where dp is the difference, top to bottom, of the slab. The minus sign serves to remind us that as we go up in the atmosphere (column) the pressure goes down (there is less air in the column).
Going back to pV = nRT and the relation mass equals moles times molecular mass (m = nM) (remember that each mole of Helium is 4.0g) 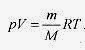
, so equation 18 3 can be written as 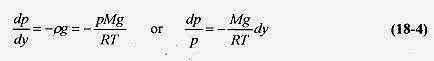
with ln A the constant of integration.
At y = 0 (on the ground), p = po, ln po = ln A, and 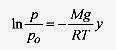
Note that in this derivation the temperature is assumed constant. The density of the air is not assumed constant because V in the density relation ρ = (m/V) was replaced with nRT / p.
18 4 What is the pressure at 3000m elevation above the earth?
Solution: Assume T = 0‹C = 273K. T decreases with height because the air is heated, primarily by radiation from the earth, so consider this an average temperature over the 3000m. Air is mostly oxygen and nitrogen and has an average molecular weight of 28.8g/mole or 28.8x10 3 kg / mole. The gravitational constant varies only slightly over this distance.
then the pressure p = poe 0.374 = 1.0atm(0.69) = 0.69atm
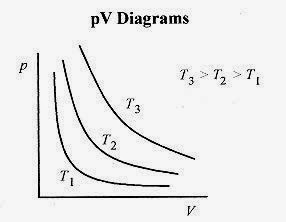
Fig. 18 3
The pV / T = constant relationship for an ideal gas is depicted in Fig. 18 3 as a collection of pV = constant curves for different temperatures. Each line of this family of hyperbolas is called an isotherm, a curve of constant temperature. A gas at constant temperature has its pV relation defined by these curves. For example, if a gas is expanded at constant temperature, the pressure follows the pV isotherm for that temperature.
Kinetic Theory
Thermodynamics is a very neat and compact subject in that the equation of state pV = nRT relation can be derived with some simple assumptions and the application of basic force, momentum, and energy concepts. The kinetic theory of gases leads directly to the equation of state for a gas.
Start withN identical molecules in a cube of volume V and sides d, and make the following assumptions and restrictions on their behavior.
1. The molecules are small compared to the dimensions of the container and the distance between molecules.
2. Only collisions with the walls are considered; collisions between molecules are ignored.
3. Collisions with the walls are elastic, and the momentum transferred is manifest as pressure on the walls.
4. The molecules and the walls are in thermal equilibrium. There is no energy transfer between the molecules and the walls.
Fig. 18 4
A molecule with velocity v and components vx, vy, vz makes an elastic collision (momentum conserved) with the x wall. The change in momentum of the molecule is numerically equal to the momentum imparted to the wall (Δp)mom = 2mvx.
This is the same expression you would write for a tennis ball bouncing off a wall. The subscript identifies this as momentum and not pressure. The time between collisions with the same wall is the time for the molecule to go to the opposite wall and return, or 2d/vx = Δt. Therefore the average force exerted on the wall by this molecule is 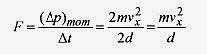
To find the force over the entire area of the wall, we need to sum all the molecular velocities. ![]()
symbolism for this summation is . The means sum the velocities, and the index, i, means to take that sum over all the molecules. The average value of the velocity in the x direction then is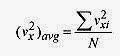
The total force on the wall due to all the molecules is ![]()
The velocity is the same in all three directions so![]()
is one third (v2) avg.
Again, the total force on the wall is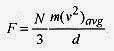
The pressure is the force per unit area, d2 in this case, so 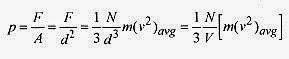
Adding a factor 2 over 2, the pressure can be written with familiar terms
where the expression m(v2)avg/2 is identified as the average translational kinetic energy of the molecule. Writing equation 18 6 another way
where (KE)tr is the total translational kinetic energy of all N molecules.
Equation 18 7 can be compared to pV = nRT, the equation of state for the gas. However, it is more instructive to rework the equation of state. The expression nR is the product of the number of moles of the gas and the gas constant, in joule per mole kelvin. The number of moles times the gas constant per mole is the same as number of molecules times a gas constant per molecule. Specifically nR = Nk, where k is the gas constant per molecule as opposed to R which is the gas constant per mole. For one mole N is NA in nR = Nk and k is easily calculated. The k is the Boltzmann constant of value 1.4x10 23 J / K. Writing the equation of state with Nk rather than nR produces
Comparing equations 18 6 and 18 8 
This identification shows that the temperature of the gas is directly proportional to the kinetic energy of the molecules. If a container of gas is heated, the pressure increases due to the molecules moving faster!
Rearrange this equation to read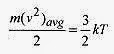
A gas molecule acting as a mass point confined to a box is viewed as being able to move in three mutually perpendicular directions. In the language of kinetic theory the molecule has three degrees of freedom. Looking at this equation then (1/2)kT of energy is associated with each degree of freedom.
The root mean square speed is defined as the square root of (v2)avg, which is 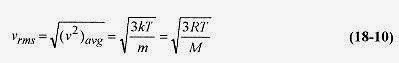
Look back to equation 18 6 and note that for the specific conditions that the gas and the walls be in thermal equilibrium, pV is a constant. This is Boyle’s law.
Again looking to equation 18 6, the pressure for constant volume is proportional to (v2)avg and in equation 18 9 (v2)avg is proportional to temperature. Pressure being proportional to temperature at constant volume is Charles’ law.
18 5 Two moles of helium are in a tank at 25‹C. Find the total translational kinetic energy, the kinetic energy per molecule, and the rms speed of the atoms.
Solution: The total translational kinetic energy is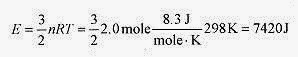
<><><><><><><><><><><><>
18 6 Isotopes of uranium are sometimes separated by gaseous diffusion. The lighter isotope, having a higher speed diffuses more rapidly than the heavier isotope. What is the ratio of the rms speeds of U 235 and U 238?
. The ratio of these speeds is 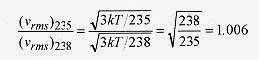
Heat Capacity of Gases
In the previous section we took the energy in the gas as the translational motion, with kT / 2 or nRT / 2 the amount of energy associated with each degree of freedom. For a container where the gas has three degrees of freedom, then the energy associated with the motion of the molecules is ![]()
and the change in energy with temperature is ΔKEtr = (3/2) nRΔT
The quantity of heat associated with a rise in temperature for n moles of a gas is ![]()
where CV is the molar heat capacity, the heat to raise one mole of the gas one kelvin. The units of CV are J / mole K. It is important to differentiate between the heat capacity for constant volume and for constant pressure. More heat is required to raise the temperature of a gas one kelvin at constant pressure than at constant volume because the change in volume requires (additional) work to be performed in the expansion. Associating ΔKEtr with ΔQ, CV should equal 3R/2. Measurements on monatomic gases verify this relation. Fig. 18 5 
Figure 18 5
The diatomic gases have additional degrees of freedom. illustrates a diatomic molecule in translation, then in rotation, first in the x y plane and then in the x z plane, and finally with the molecule in vibration. The rotation adds two new degrees of freedom and the vibration adds another two degrees of freedom.
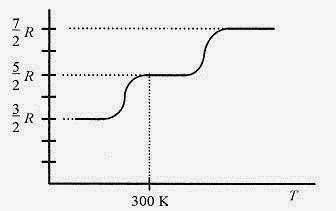
Experimental measurements on diatomic molecules show that for low temperatures (three translational degrees of freedom only) CV = 3R / 2. As the temperature of the gas is increased the diatomic molecule begins to rotate (adding two more degrees of freedom) and the CV climbs to 5R / 2. At even higher temperatures the molecule begins to vibrate (adding another two degrees of freedom) and the CVreaches 7 R / 2. This is shown in Fig. 18 6.
Fig. 18 6
At sufficiently high temperatures diatomic molecules require more heat to raise their temperatures one kelvin than do monatomic molecules because diatomic molecules are not only translators but rotators and possibly vibrators. Most diatomic molecules are rotators at room temperature.
18 7 How much heat is required to raise the temperature of 2.0 mole of monatomic gas 25K starting at room temperature.
Solution: Use ΔQ = nCVΔT with CV for a diatomic gas as 3 R / 2 so 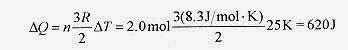
<><><><><><><><><><><><>
18 8 For the previous problem find the heat required if the gas were diatomic.
Solution: Assume that at room temperature the diatomic molecule is a translator and rotator (see Fig. 18 6) so that CV = 5 R / 2. Then