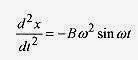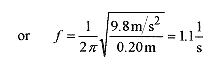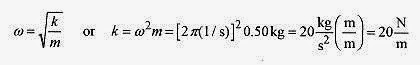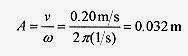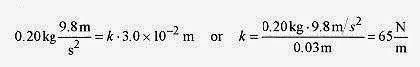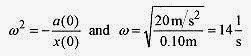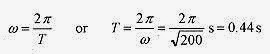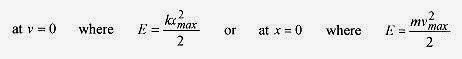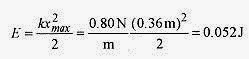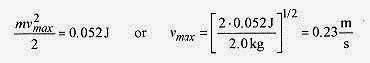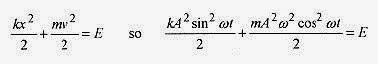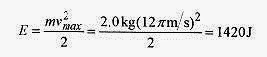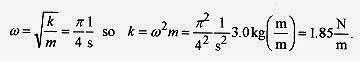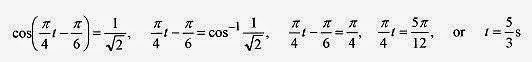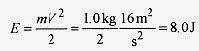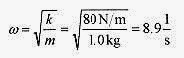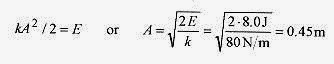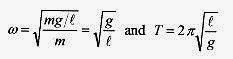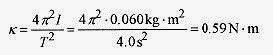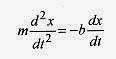Simple Harmonic Motion
Simple harmonic motion is the phrase used to describe a repetitive harmonic motion such as the motion of a mass oscillating up and down on the end of a spring, the rotation of a point moving at constant speed along a circle, or a pendulum in small (amplitude) oscillation. The most convenient visualization of an oscillating system is the mass spring system. This is also the one most analyzed. Simple harmonic motion is often characterized by the period, the time for one oscillation, and the amplitude, the maximum excursion from equilibrium. (Period is the time for one repetition and is measured in seconds, while the frequency is the reciprocal of the period and is measured in Hertz or 1/s.) More complete analysis usually involves writing statements about the total energy of the system.
Fig. 15 1
For a first look at simple harmonic motion consider the analysis of a mass spring system. The kinetic energy is (1/2)mv2, the energy associated with the moving mass, and the potential energy of the system is (1/2)kx2, the energy associated with the elongation and compression of the spring. Here we assume the spring is a Hook’s law spring obeying F = kx. You may want to review the discussion of the energy stored in a Hooke’s law spring in Chapters 7 and 8.
To describe the motion of the mass from a force point of view, take the force as kx and equate that to ma with a written as the second derivative of position.
If you don’t have much experience in calculus, this may look like a formidable equation. It is not. It has a very simple and obvious (once you’ve seen it) solution. Acceleration is the time derivative of velocity (a=dv/dt), and velocity is the time derivative of displacement (v=dx/dt) as defined in Chapter 1. Acceleration, then, can be written as the second derivative of the displacement.
The solution to this equation requires that the second derivative of a function of x with respect to time be proportional to the negative of the function. This reduces to the question: What function differentiated twice yields the negative of itself? The answer is a sine or cosine function or a linear combination of both. If you have not encountered this yet, see the table of derivatives in the Introduction, Mathematical Background.
The derivative of the sine function is the cosine function and the derivative of the cosine function is the negative of the sine function. Two derivatives of the sine function yield the negative of itself. This is also true of the cosine function. The solution can also be written as the sine or cosine of a constant times t plus a phase angle. Acosωt + Bsinωt and C(cosorsin)(ωt + θ) are equivalent solutions.
Take as a general solution x = Acosωt + Bsinωt
If the starting position (t = 0) is taken as an extreme of the motion, corresponding to maximum compression of the spring, then B = 0, and A is the amplitude of the motion. If the starting position were taken at the midpoint of the motion, then at t=0 the amplitude would have to be zero; therefore, A = 0 and B would be the amplitude. (Remember sin0 = 0 and cos0=1.) The choice of initial condition determines whether the motion is described by a sine or cosine function.
Use as a solution x=Bsinωt. Take two time derivatives to obtain
and substitute in the force law statement, kx= m(d2x/dt2)
In the statement x=Bsinωt, ω is 2π/T. The 2π effectively scales the sine function, and the ratio t/T determines the fraction of 2π required for determining the value of the function. For example, at t=T (one period) the sine function has gone through one cycle. At t=T/2 (one half period) the sine function has gone through one half cycle. Because of the 2π factor, ω is called the angular frequency. The term frequency applies to f in the relation
In solving problems be careful to differentiate between frequency, f, and angular frequency, ω.
15 1 A mass spring system has mass 2.0kg, spring with constant 3.0N/m, and amplitude of vibration 0.10m. Calculate the angular frequency and frequency. Write expressions for the position, velocity, and acceleration as a function of time. Take t=0 at the equilibrium position. Graph position, velocity, and acceleration as a function of time.
Solution: The angular frequency is
The frequency is
If t=0 at the equilibrium position, then the displacement must be given by x=Bsinωt.
At t=0 the sine function is zero, so this is the appropriate function to describe the motion. For an amplitude of 0.10m this expression should read
The velocity is the time derivative of x or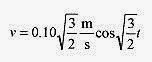
At t=0 the velocity, as the mass passes through the equilibrium position, is a maximum. The acceleration is another time derivative of the velocity or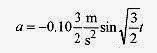
At t=0 the acceleration is zero; the spring is neither elongated nor compressed (at the equilibrium position), so the force (and acceleration) of the mass is zero.
Fig. 15 2
These three curves graphed one under the other in Fig. 15 2 give a good picture of the motion from the position, velocity, and acceleration point of view.
Look at these graphs and visualize the motion. At the equilibrium position, displacement is zero, the velocity is a maximum, and acceleration is zero. As the mass moves to the positive maximum displacement, the velocity decreases to zero and the acceleration (down) increases to a maximum. At the maximum displacement, the velocity is zero and the acceleration is a maximum. As the mass starts down, the velocity is negative reaching maximum again as the mass passes through equilibrium. Go over this picture of the motion until the relationship between position, velocity, and acceleration is clear in your mind.
<><><><><><><><><><><><>
15 2 A piece of metal rests on top of a piston executing simple harmonic motion in the vertical plane with an amplitude of 0.20m. At what frequency will the piece of metal (float) at the top of the cycle?
Solution: Take the motion to be described by y=(0.20m)cosωt.
Two derivatives produces the acceleration a= (0.20m)ω2cosωt.
The maximum acceleration, which is the constant (0.20m)ω2, is at the extreme of the motion where the cosine term is one. At the bottom of the cycle, the piece of metal will experience an acceleration greater than g and at the top an acceleration less than g. The piece will gfloath when that acceleration is greater than g
<><><><><><><><><><><><>
15 3 A mass spring system has a mass of 0.50kg and period of 1.0s. The maximum velocity is 0.20m/s. Find the frequency, angular frequency, constant of the spring, and displacement for this velocity and period.
Solution: The frequency is the reciprocal of the period or f=1.0(1/s), and the angular frequency is 2πf or 2π(1/s). The spring constant comes from the relation
The general expression for velocity is v=Aωcosωt where Aω is the maximum velocity. It makes no difference whether the function is a sine or cosine. The maximum velocity is still Aω. The amplitude for this particular motion then is
<><><><><><><><><><><><>
15 4 An 0.80kg mass hangs from a spring. When an additional 0.20kg mass is added the spring elongates another 3.0cm What is the period of oscillation of the spring?
Solution: First determine the force constant of the spring from F= kx
The period is the reciprocal of the frequency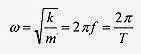
<><><><><><><><><><><><>
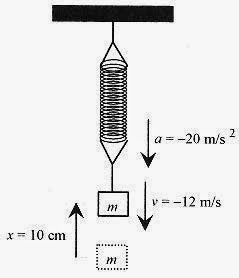
15 5 An oscillating mass spring system has displacement 10cm, velocity 12m/s, and acceleration 20m/s2. What is the period of the system?
Solution: Since the displacement is not zero at t=0, the motion cannot be described with a sinωt function. Since the velocity is not zero at t=0, the motion in not at an extreme, and cannot be described with a cosωt function. Because of the initial conditions, a sine or cosine function of ωt plus a phase angle must be used. It makes no difference whether a sine or cosine function is used since the phase angle can absorb the 90‹ difference between a sine and a cosine function. Look at Fig. 15 4 and go over the logic in this paragraph until it is clear in your mind.
Take x=Acos(ωt+θ) then v= Aωsin(ωt+θ) and a= Aω2cos(ωt+θ)
Using the equations for x and a, at t=0,
The phase angle comes from v(0) and a(0).
The specific functional relations are
Fig. 15 4
Look at these relations and relate them to what the system is doing at t=0. The amplitude is past its maximum positive excursion and on the way down corresponding to the cosine function at 83‹ The velocity is negative and heading toward its most negative value as is the negative sine function at 83‹. The acceleration is negative and tending toward zero just as is the negative cosine function at 83‹.
Energy Analysis.
Simple harmonic motion can be analyzed from an energy point of view. The energy stored in a spring is kx2/2, and the energy stored in the mass by virtue of its velocity is mv2/2. A total energy statement for the system then is
which is most easily evaluated by looking at the extrema of the motion,
15 6 Find the maximum velocity of a mass spring system with mass 2.0kg, spring constant 0.80N /m, and amplitude of oscillation 0.36m.
Solution: All the energy of the system is stored in the spring at maximum displacement so the total energy is
This is the total energy in the system, and at zero displacement all this energy is in kinetic energy so
It is relatively easy, and quite instructive, to show that the total energy of an oscillating system is a constant. Take an oscillating system with x=Asinωt and velocity v=Aωcosωt. Now place these expressions for x and v into the total energy statement
But from the previous analysis ω 2=k/m so the statement reads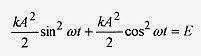
which shows that the total energy does not vary over time. The energy does not vary over one cycle, it goes back and forth from kinetic to potential in a manner such that the total (energy) remains a constant.
<><><><><><><><><><><><>
15 7 Find the amplitude, angular frequency, and frequency for a mass spring system with mass 2.0kg that is oscillating according to x=(2.0m)cos(6πt/s). Write and graph the expressions for position, velocity, and acceleration. Finally, calculate the total energy of the system.
Solution: The amplitude, angular frequency, and frequency are determined by comparing x=(2.0m)cos(6πt/s) to the standard form x=Acosωt=Acos2πft.
The amplitude is 2.0m. The angular frequency is 6π(1/s). The frequency is 3(1/s).
The expressions for position, velocity (first derivative), and acceleration (second derivative) are shown in Fig. 15 5 associated with the graphs.
In Fig. 15 5, the units are dropped in the argument of trigonometric functions, and the maximum values for the velocity and acceleration are placed on the graphs.
Fig. 15 5
The conservation of energy statement is![]()
For a 2.0kg mass the total energy can be evaluated
when x is zero and the velocity maximum.
<><><><><><><><><><><><>
15 8 For a 3.0kg mass spring system executing simple harmonic motion according to y=(4.0cm)cos(πt/4 π/6), what is the total energy and the y position for equal division of the energy between kinetic and potential?
Solution: The general expression for displacement is y=Acos(ωt+θ). Looking at the two expressions,
Knowing k, the total energy is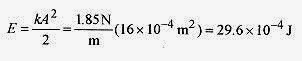
The potential energy due to position is ky2/2 and y=Acos(ωt+θ) so that the energy due to position is kA2cos2(ωt+θ)/2. The energy due to position is 1/2 the total when the factor .
For this particular system, the time for equal division of the energy is when
The position of the mass for equal division of the energy is
As an exercise, calculate the velocity at this time.
<><><><><><><><><><><><>
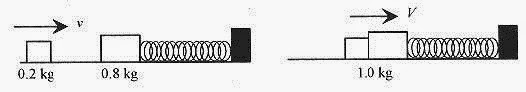
15 9 A 0.20kg block traveling at 20m/ s slides into and sticks to an 0.80kg block resting on a frictionless surface and connected to a spring with force constant 80N/m. What is the angular frequency, frequency, and displacement as a function of time? Also what fraction of the original energy in the moving block appears in the system?
Solution: The collision is inelastic; the blocks stick together, so applying conservation of momentum 0.20kg(20m/s)=(1.0kg)V. The velocity of the 1.0kg combination is V=4.0m/s. This is the velocity of the system at zero displacement. The analysis of this collision is similar to the ballistic pendulum problem in the chapter on collisions.
The total energy of the oscillating system is
Fig. 15 6
The frequency is from ω=2πf or f=ω/2π=1.4(1/s)
The general expression for the displacement is y=Asinωt. The sine function without a phase angle completely describes the motion because it starts (t=0) at the equilibrium position with maximum velocity. The amplitude of the motion is obtained from
so the specific expression for the displacement is y=(45cm)sin(1.4t/s)
The initial energy is all in the small block
The energy in the oscillating system is 8.0J, so the fraction of the original energy that appears in the oscillating system is F=8.0J/40J=0.20
Applications
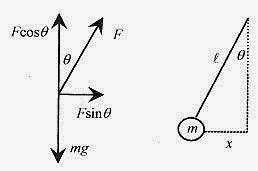
The oscillating simple pendulum can be analyzed in terms of force. For small oscillations the tension in the cable can be written in components; one vertical and the other the grestoring force,h the force that returns the mass to equilibrium. For small θ, cosθ.1 and sinθ.x/l, with x being the displacement, approximately equal to the arc length.
Fig. 15 7
For small angle oscillations Fcosθ.F and Fsinθ. mgx/l= (mg/l)x. Notice that in this equation mg/l plays the same role as k in the mass spring systems.
By analog then The most important point to note is that the period is independent of the mass. Historically this was one of the first instruments to measure gg,h the gravitational constant.
<><><><><><><><><><><><>
The torsional pendulum, a disk suspended by a thin rod, can be analyzed using torque. The disk is rotated by applying a torque. Torque is proportional to the angle in the same manner as force is proportional to compression (or elongation) in a spring. The torque is written in terms of the moment of inertia and the angular acceleration [ ] which is set equal to the total torque, kθ the torsional constant times the angle, yielding the equation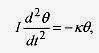
which is the same form as the force equation for the oscillating mass spring system. By analog then θ=θmaxsinωt where 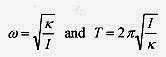
Fig. 15 8
15 10 What is the restoring constant (torsional constant) for a disk of mass 3.0kg and radius 0.20m oscillating with a period of 2.0s?
Solution: The moment of inertia for a disk is 
Note that these are the correct units since torque has the dimensions N.m, and in Torque= kθ the angle is in (dimensionless) radians.
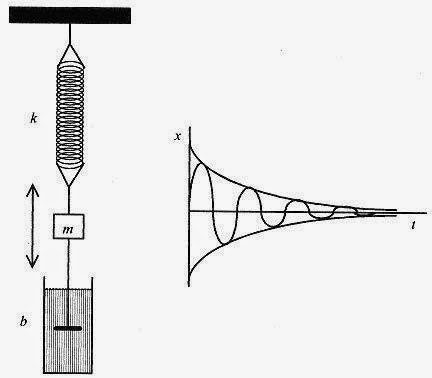
Damped Mass Spring System
The system is usually depicted as a mass spring with a dashpot, a piston in a thick liquid. In the case of the spring, the restoring force is proportional to displacement, compression or elongation of the spring. With the piston in the fluid, however, the retarding force is proportional to velocity, not position. Move your hand through water and notice that the retarding force (the force on your hand) is proportional to velocity! The velocity dependent force component of a typical automobile suspension is called a shock absorber. It is usually a piston in a cylinder of oil. Since the retardation force is velocity dependent the differential equation governing the motion is
Notice that the velocity dependent term has the same sign as the displacement dependent term. It too opposes the motion.
Fig. 15 9
This differential equation is solved with sophisticated techniques. Here we give an intuitive solution.
This second order differential equation is solved as a product of solutions, the position dependent part and the velocity dependent part. The position dependent part is a sine or cosine function with a phase angle. The velocity dependent part is from the velocity dependent part of the equation
The solution to this part of the equation is one which when differentiated once produces the negative of itself. The function that does this is an exponent to the negative power. The particular solution that satisfies this differential equation is![]()
where ω’ is slightly different from ω. The damping of the exponential does exactly as we know from experience. Oscillations subject to velocity damping (a velocity dependent restoring force) eventually die out.