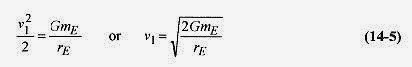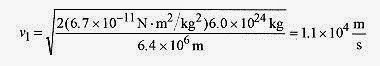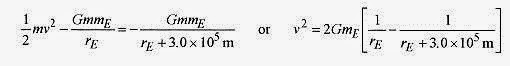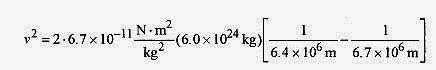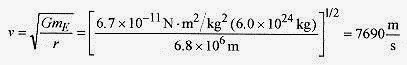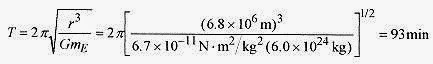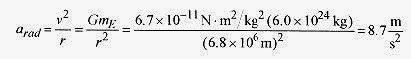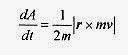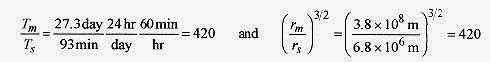Gravity
The basic law of gravity describes the force of one mass on another
where G is a constant that depends on the units of the m’s, the masses, and r, the distance between centers. In SI units the gravitational constant is G = 6.7 ~ 10 11 N.m2/kg2. The gravitational force acts along the line of centers and is an action reaction pair. (The force on both masses is the same.) Gravitational forces are vectors and superpose (add) as vectors.
The gravitational force of attraction for a mass of small (compared to the earth) radius on the surface of the earth is known as the weight of that mass on the earth and is 
being called the acceleration due to gravity. Weight then is the familiar W = mg. Acceleration units (the units of g) times mass produce force or weight units. Also, ggh is a vector that points between the center line of the masses involved.
14 1 What is the gravitational force of attraction between two 7.5kg bowling balls with 0.50m between centers?
Solution Use the gravitational force equation
Each ball is attracted to the other on a line between their centers.
Gravitational Potential
Following the general form for potential energy, the gravitational potential is defined as the work performed by the gravitational force when r increases from r1 to r2.
The minus sign is in the integral because the force points opposite to the direction of increasing r. The force decreases as r increases. The integral definition is necessary because the force depends on r. Performing the integration
Look at the signs in this expression. If r1 is taken at the surface of the earth and r2 above the earth then the gravitational potential at r2 is positive (less negative) with respect to the earth.
Notice that the general expression for gravitational potential is
The zero of gravitational potential is at r = ‡ The gravitational potential is negative at the surface of the earth and becomes more negative as we go in toward the center of the earth.
With the gravitational potential energy and the conservation of energy we can calculate the escape velocity. This is the velocity with which we would have to shoot something vertically up to completely remove it from the earth’s gravitational pull. 14 2 Calculate the escape velocity on the surface of the earth. Solution: The escape velocity is the minimum velocity for a mass to escape the gravitational attraction of the earth. Envision a mass being shot vertically up from the surface of the earth and write the energy statement for it at two levels, the surface of the earth and some height r further out from the center of the earth. The conservation of energy statement will read: The kinetic plus potential energy on the surface of the earth equals the kinetic plus potential energy at some point r.
The escape velocity is that velocity that will produce zero velocity (v2 = 0) when the mass is infinitely far away (when r = ‡, GmmE / r = 0). This reduces the equation to
The mass and radius of the earth are on the constants page of your text so
As an exercise calculate the escape velocity for the moon of radius 1.7 ~ 106 m and mass 7.4 ~ 1022kg.
<><><><><><><><><><><><>
14 3 What initial vertical speed is necessary to shoot a satellite to 300km above the earth?
Solution: Use energy analysis and the same energy balance equation as in the previous problem with the velocity at 300km equal to zero.
Putting in the numbers
or
Satellites
A satellite, whether artificial or moon, moves in a circular orbit about a larger planet. The gravitational force supplies the center directed acceleration necessary to make the satellite move in a circular orbit. Mathematically stated the force balance is
which reduces to v2r = Gm1 with the right hand side a constant.
The orbit radius, period, and velocity are related through
14 4 Calculate the speed, period, and radial acceleration of a satellite placed in orbit 400km above the earth.
Solution: The orbit radius is 6.4 ~ 106m+0.4 ~ 106 m = 6.8 x 106m
The radial acceleration is
<><><><><><><><><><><><>
A geosynchronous satellite is one that is orbiting at an equator and always over the same spot on earth. Using the expression for the period of a satellite, find the height for a satellite with a period of 1.0 day.
Kepler’s Laws
There are three Kepler’s laws that govern planetary motion. Kepler based these laws on observations made before the invention of the telescope! They are:
I. All planets move in elliptical orbits with the sun at one focus.
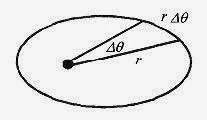
II. A line from the sun to the planet sweeps out equal areas in equal lengths of time (see Fig. 14 1).
III. The square of the period is proportional to the cube of the semi major axis of the ellipse.
Kepler’s second law is consistent with the angular momentum of the motion being a constant. Refer to Fig. 14 1. For small angles the area swept out by the arc is equal to the area of a triangle with the side rƒ¢ƒÆ nearly perpendicular to the sides r. The area of the triangle is (1 / 2)(rƒ¢ƒÆ)r. In calculus notation the differential area is dA = (1/2)r2dƒÆ, and the change in area with time is 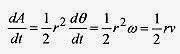
Fig.14 1
The magnitude of the cross product of two vectors is proportional to the area of the trapezoid defined by the vectors (see the Introduction, Mathematical Background). Here the two vectors defining the area swept out are r and v. Rewriting rv as the magnitude of the cross product of r and v, or more conveniently mv,
but r ~ mv is L, the angular momentum, which is a constant of the motion. Kepler’s second law is equivalent to conservation of angular momentum.
Going back to rotational dynamics, dL / dt = r ~ F, and in planetary motion the force is gravitational and acts along r, so r ~ F must equal zero, and if the derivative of the angular momentum is zero then the angular momentum is a constant.
Equation 14 6 is based on conservation of energy and shows T as proportional to the 3/2 power of r. This is verification of Kepler’s third law for the case of circular orbits.
14 5 The orbit radius of the satellite of problem 14 4 is 6.8 ~ 106 m, and the period is 93 min. The orbit radius of the moon is 3.8 x 108 m, and the period is 27.3 days. Is this consistent with Kepler’s third law?
Solution: Kepler’s third law states that T is a constant times the 3/2 power of the radius, so
If the constant is the same then 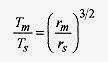
The satellite and moon orbits verify Kepler’s third law for circular orbits.