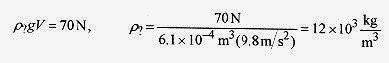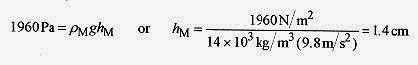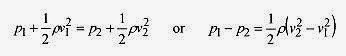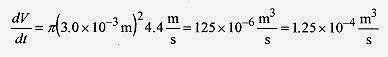Fluids
There are several definitions and concepts that are unique to the study of fluids. Let’s review these basic definitions and concepts working some problems along the way.
Density is simply mass per unit volume, or symbolically ρ = m/V. The density that is most useful in problems is the density of water, which is 1g / cm 3 or 1000kg / m3.
The ratio called specific gravity is better called the specific density since it is the ratio of the density of another substance to the density of water. Aluminum has a specific gravity of 2.7, which means that for the same volumes, aluminum has 2.7 times the mass of water. Relative density or specific gravity is used to measure the relative amount of sulfuric acid (compared to water) in a storage battery or the relative amount of ethylene glycol (compared to water) in an automotive cooling system.
Pressure
The pressure in a fluid is defined as the force per unit area, ρ = F / A. And the units of pressure are N / m2 , also called a Pascal or Pa. Atmospheric pressure, the force per unit area exerted by the air, is 1.0 x 105Pa=151b/in2 =1atm.
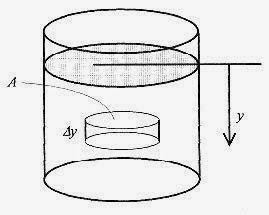
To determine the pressure at any depth in a liquid consider the differential change in force due to a column of liquid of cross section A. The increase in force between the top and bottom of this differential piece is equal to the weight of the piece.
It is convenient to write the mass of this little piece as ρΔV so that the force (difference) is
Now ΔV for this piece is A Δy so ΔF=ρgAΔy. The pressure is the force per unit area so rewriting
Dropping the differential notation
Fig. 16 1
Ify is measured from the bottom of the liquid then insert a negative sign on the right side of the equation. This discussion of pressure leading up to equation 16 3 is equally valid whether the delta or differential notation is used. This is true of most of the discussion of fluids. We start with delta notation and switch to differential notation in some later development.
16 1 Calculate the pressure at the bottom of a 3.0m deep pool.
Notice that this pressure is the difference between the top and the bottom. This difference is known as the gauge pressure. Adding the atmospheric pressure at the top surface of the pool gives the absolute pressure at the bottom of the pool.
<><><><><><><><><><><><>
The typical automobile tire pressure is the gauge pressure. With the tire inflated the pressure might read 30 1b / in 2. If the tire were gflath the pressure would read zero. The gauge pressure for the gflath tire would be zero but the absolute pressure would be 15 1b / in2. Likewise the tire with gauge pressure of 301b / in 2 would have an absolute pressure of (30+15) Ib / in2. The gauge that measures the tire pressure is measuring the difference in pressure between inside and outside the tire.
Pressure exerted on a liquid in a closed container is transmitted throughout the liquid. The pressure is the same in every direction varying only with height as described by equation 16 3. In most devices using fluids to transfer forces, the fluid is nearly incompressible and height variations are insignificant.
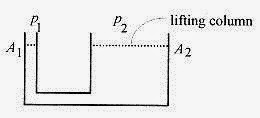
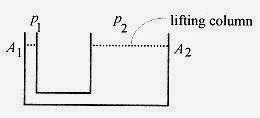
16 2 Consider the classic problem of a hydraulic lift. In a typical service station 12 atm is applied to the small area with the lifting column 9.0cm in radius. Find the force transferred to the large area and the mass of vehicle that can be lifted.
Fig. 16 2
Solution: The hydraulic fluid is at the same level so ρ1 = ρ2. or 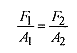
A force F1 applied at A1 is multiplied by the ratio of the areas so F2=(A2/A1)F1
The lifting force F2 can also be rewritten as F2= A2(F1/A1=A2ρ1, and putting in the numbers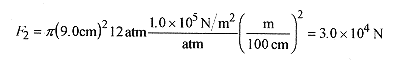
And the equivalent mass (from F=mg) is 3.1x 103 kg. This number is somewhat larger than the mass of typical cars.
Barometer and Manometer
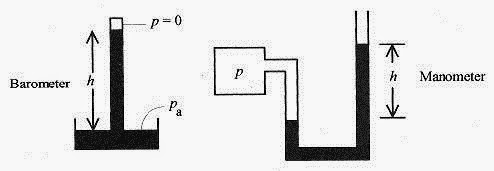
A simple atmospheric air pressure gauge is the mercury barometer. A closed tube is filled with mercury and then inverted in a beaker of mercury. The pressure at the top of the mercury column is zero. And the column is held up by the (atmospheric) pressure on the surface of the mercury. Looking to Fig. 16 3, the pressure at the bottom of the column is ρgh. This experiment was first done by Torricelli, and the pressure was given as the pressure associated with the column of mercury. Pressures are often measured in mm of Hg or Torr. and refer back to this experiment.
Pressure exerted on a liquid in a closed container is transmitted throughout the liquid. The pressure is the same in every direction varying only with height as described by equation 16 3. In most devices using fluids to transfer forces, the fluid is nearly incompressible and height variations are insignificant.
16 2 Consider the classic problem of a hydraulic lift. In a typical service station 12 atm is applied to the small area with the lifting column 9.0cm in radius. Find the force transferred to the large area and the mass of vehicle that can be lifted.
Fig. 16 2
Solution: The hydraulic fluid is at the same level so ρ1 = ρ2. or 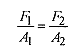
A force F1 applied at A1 is multiplied by the ratio of the areas so F2=(A2/A1)F1
The lifting force F2 can also be rewritten as F2= A2(F1/A1=A2ρ1, and putting in the numbers 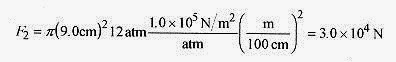
And the equivalent mass (from F=mg) is 3.1x 103 kg. This number is somewhat larger than the mass of typical cars.
Barometer and Manometer
A simple atmospheric air pressure gauge is the mercury barometer. A closed tube is filled with mercury and then inverted in a beaker of mercury. The pressure at the top of the mercury column is zero. And the column is held up by the (atmospheric) pressure on the surface of the mercury. Looking to Fig. 16 3, the pressure at the bottom of the column is ρgh. This experiment was first done by Torricelli, and the pressure was given as the pressure associated with the column of mercury. Pressures are often measured in mm of Hg or Torr. and refer back to this experiment.
Fig. 16 3
An open tube manometer as depicted in Fig. 16 3 can be used to measure pressure. The force exerted by the gas is ρA, and this force raises the column of (usually) mercury by exerting a force equal to ρgV, the weight of the column. The volume of the column. V is Ah so equating forces. ![]()
The manometer measures the difference in pressure between two points and as such measures gauge pressure. The open tube manometer is equally valuable as a vacuum gauge. The difference in column height (the height of the mercury in the open tube is now lower than in the tube connected to the vacuum chamber) is a measure of the vacuum.
Buoyancy
Material objects appear to weigh less when partially or completely submerged in liquids. This apparent loss in weight is due to the buoyant force of the liquid. This buoyant force is expressed by Archimedes’ principle: A body partially or completely immersed in a liquid is buoyed up by a force equal to the weight of the liquid displaced.
16 3 The classic problem in buoyancy is to determine if a (gold) statue is really gold by measuring its weight in and out of water. A certain ggoldh statue weighs 70N when out of water and 64N when immersed in water.
Solution: With the statue suspended from a scale the weight is mg, or density times g, times the volume of the statue. In equation form mg=ρ?gV = 70N.
In water the statue weighs less because of the buoyant force: ρ?gV ρwgV = 64 N
Subtracting one equation from the other we can determine the volume from
With this value for V, calculate the unknown density from the first equation
This density is much less than the density of gold ρgold =19x103kg/m3) and closer to the density of lead (ρlead = 11x 103 kg/m3) suggesting that the statue is lead with a thin covering of gold.
This same experiment can be conducted by lowering the statue into a beaker of water on a scale. The volume is determined by the increase in the level of water in the beaker. The drop in tension in the cord is offset by the increase in the scale reading when the statue is lowered into the beaker and the calculations proceed as before.
<><><><><><><><><><><><>
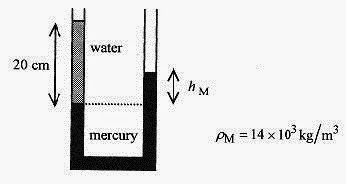
16 4 A U tube originally containing mercury has water added to one arm to a depth of 20cm. What is the pressure at the water mercury interface? What is the height of the mercury column as measured from the watermercury level?
Solution: Assume that the difference in air pressure can be neglected. The pressure at the watermercury interface is due to the column of water plus the atmospheric pressure ρo, or p=po+pwg. 20cm . Neglecting po gives the gauge pressure as![]()
Fig. 16 4
At this level (the level of the interface) the pressure on the mercury arm must be the same. (If it were not the liquids would move!) The height of the mercury level is
As we expect, the same pressure is produced by a much shorter column of mercury.
Second Solution:Analytically the problem can be approached by writing expressions for the pressure at the level of the water mercury interface.
In the left arm pW = po + pWghW, where the subscript W refers to the water column.
In the right arm the pressure is pM = po + pMghM, where the subscript M refers to the mercury column.
Since these pressures must be equal at the water mercury interface
Practice variations of this problem with different liquids (different densities).
<><><><><><><><><><><><>
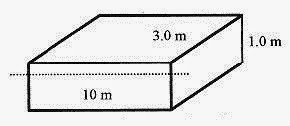
16 5 A rectangular shaped, open top steel barge is 10m by 3.0m and has sides 1.0m high. The mass of the barge is 8.0 x 103kg. How much mass can the barge hold if it can safely sink 0.75m?
Solution: The volume of water displaced is length times width times allowed depth, 10mx3.0mx0.75m=22.5m3. The buoyant force is the weight of the water displaced (Archimedes’ principle)
Fig. 16 5
This buoyant force must support the barge (mB) plus the load (mL.![]()
As an additional exercise calculate how deep the unloaded barge will sink in the water.
16 6 An iceberg floats in seawater. What percentage of the iceberg is submerged?
Solution: Assume a cubical iceberg of side s. You can assume any shape you wish. Cubical, however, seems convenient. The mass of the cubical iceberg is pices3 , and the weight is pices3g. Since the density of the iceberg is less than the density of the seawater the iceberg will float. The buoyant force of the seawater is pSWg times the volume submerged. Since the iceberg is cubical the submerged volume must be s2 , the area of the bottom of the iceberg, times a fraction of the side k times s.
This buoyant force must equal the weight of the iceberg. pices3g = pSwgs2(Ks).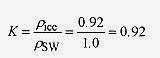
So K, the fraction of the iceberg submerged is
Thus 92% of the iceberg is submerged.
Fluid Flow.
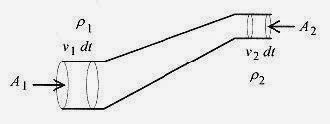
The last, and possibly most important, of the fluid laws are associated with the flow of liquids through tubes of varying cross section. The best place to start on this is with a simple statement of mass flow in a pipe of variable thickness.
Fig. 16 6
Mass flow requires p1A1v1dt = p2A2v2dt
Density is mass per unit volume, and Avdt = Adx is a differential volume, so that the above equation is a statement that there is the same mass of fluid in the two differential volumes shown. Since in most fluids p1 = p2, the mass flow (also called the continuity equation) statement reduces to
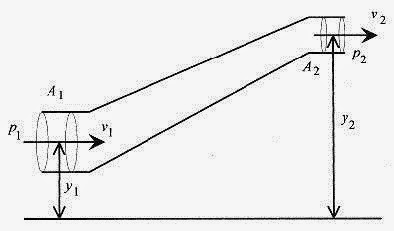
Now look to the Bernouli equation, which takes account of changes in the gravitational potential and pressure. The law is understood from a work energy point of view. If liquid is flowing through a tube as shown in Fig. 16 7, then the work done on the fluid in pushing it through the tube must be manifest as increased potential and kinetic energy.
Consider a mass of fluid moving through this tube. The volume of this mass of fluid is
where the ds’s are the respective distances along the tube necessary to make up the same mass of fluid. The work performed on this mass of fluid is (note that the work is force, pA, times distance,ds).t
The change in kinetic energy, basically (1/2)mv2 , is ![]()
And the change in potential energy (analogous to mgh) is dU = pgdV (y2 y1).
Equating the work performed to the energy change yields the Bernoulli equation ![]()
![]()
This is all the information necessary to do fluid problems as found in most physics texts. Buoyancy and pressure problems do not usually present a problem. Calculating pressures is sometimes encountered in the context of a flow problem, In flow problems the usual procedure is to first apply the mass flow statement, equation 16 5. Then apply the Bernoulli equation. In the application of this equation it is often necessary to take some of the terms as zero. The ability to know when to take a term as zero comes from experience.
Venturi Tube
The venturi tube is used as a siphon or speed indicator. The tube is constructed as shown in Fig. 16 8. Apply the Bernoulli equation without the gravitational term (most venturi tubes are mounted horizontally).
Fig.16 8
From the mass flow equation, equation 16 5, A1v1 = A2v2 so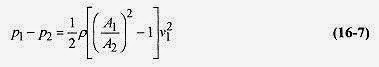
Since A1 is larger than A2, p1 p2 is positive. The pressure is reduced in the smaller section, or throat, of the venturi. A side tube connected to the narrow region will siphon liquid or gas. The side tube also can be connected to a pressure gauge to measure velocity, which is proportional to the square root of pressure.
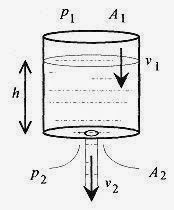
16 7 Calculate the speed at which liquid flows out a small hole in the bottom of a large tank containing liquid to a depth of 1.0m.
Fig. 16 9
Solution: Apply the Bernoulli equation![]()
Note that p1 = p2, so remove these terms from the equation. Because A1 >> A2,v1 is very small and is taken as zero. Take y1 = 0, so y2 is the depth of the liquid. The Bernoulli equation for this case reduces to
The velocity of liquid from a small hole in a container depends only on the height of the column of liquid.
Go back through this problem and note how the terms of the Bernoulli equation were handled, the pressure being the same, and the logic leading to the v1 term being taken as zero. This is the hard part of this problem. If, in doing a problem like this, you do not see that v1 can be taken as zero you will be stuck. You can move no further on the problem because there is not enough data to calculav1.
<><><><><><><><><><><><>
16 8 For the situation of problem 16 7, find the volume flow rate, that is dV/dt, for a hole of radius 3.0mm.
Solution: The flow rate is the instantaneous rate of liquid flowing out of the container. This is most easily obtained from the statement of the differential volume.
For a hole of radius 3.0mm and liquid depth 1.0m
<><><><><><><><><><><><>
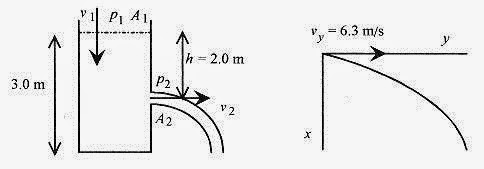
16 9 A cylindrical container of radius 20cm contains water to a height of 3.0m. Two meters down from the water level there is a hole 0.50cm in radius. Find the velocity and volume flow rate of water leaving the hole. What is the shape of the stream, and where does it strike the ground?
Solution: Apply the Bernoulli equation
The pressure is the same: p1 = p2. The velocity v1 is so small compared to v2 that it can be neglected: set v1 = 0. The distance from the top of the water to the hole is 2.0m.
Fig. 16 10
The differential volume of liquid flowing out of the hole is dV = A2v2dt 
To analyze the motion look at a differential piece of the stream. Set up a right handed coordinate system with origin at the exit hole. This coordinate system is similar to ones used in some projectile problems. The differential piece of liquid has initial velocity in the +y direction of 6.3m/s and is acted upon by gravity in the +x direction.
The six equations of motion for acceleration, velocity, and position in the x and y directions are 
These are the equations of a parabola or half of a parabola in this case.
The differential piece of water falls 1.0m so set x = 1.0m in the equation for x![]()
This is the time for the differential piece of the stream to reach the ground.
Then y at t= 0.45s is y|t=0.45=2.8m. The stream strikes the ground at 2.8m from the tank.
The approximation of v1 as zero is justified with the continuity equation, A1v1 = A2v2.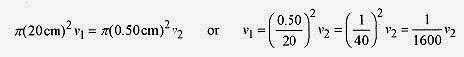
The v2 is 1600 times v1!
As a side exercise solve the equation y=6.3t for t and substitute into the equation for x, obtaining an equation in the form x = ay2, the equation of a parabola. Then substitute x = 1.0m to obtain y.