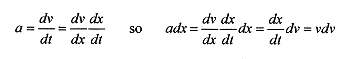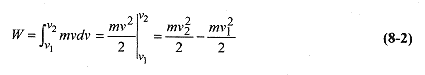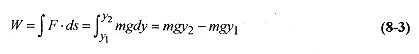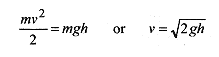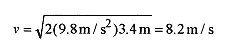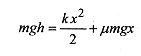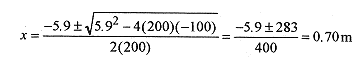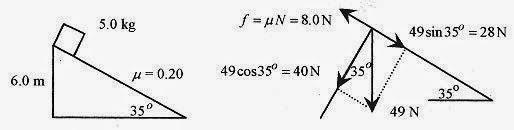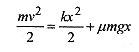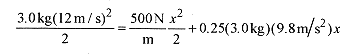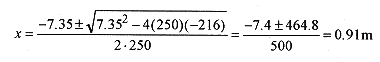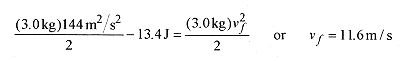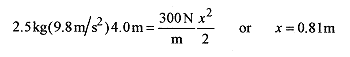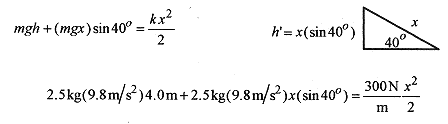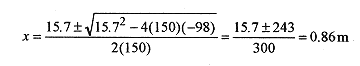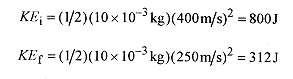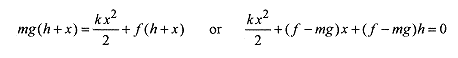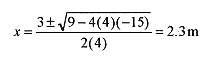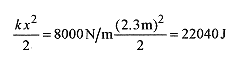Work Energy Problems
Work energy analysis is a very powerful tool for solving problems. Force analysis works well in problems where there is a constant force or where there is a balance of forces. But when the force acts over a distance, especially when that force is not constant, then work energy analysis is the appropriate analytic tool. To handle the transformation of force over a distance (or work performed) to energy, we first need to define the concepts of kinetic and potential energy.
Kinetic Energy
A constant force applied to a mass over a distance produces an acceleration according to F = ma. This acceleration over a distance changes the velocity of the mass in accord with the kinematic equation .
If a in this equation is replaced with F/m then .
If the constant force over a distance is identified as the work then
This simple derivation suggests a more formal look at the work performed on a mass by a force.
The work performed on a mass by a force is
defined as the integral of the force times the distance over a specific distance. Mathematically this is If the force is replaced by ma and the distance taken in one dimension, for convenience, then the work integral becomes
The acceleration can be written as a chain derivative (see the Introduction, Mathematical Background).
which makes the integral read
When a force is applied to a mass over a distance and that mass accelerates, then there is a difference in velocity of the mass between before and after the application of the force. The work performed is manifest in this velocity and the mv2/2 form is called kinetic energy. Work is transformed into kinetic energy.
Gravitational Potential Energy
Gravitational potential energy comes from the acceleration due to gravity and the accompanying force on a mass on the surface of the earth. If a mass is raised from a height y1 to a height y2, directly opposite the acceleration due to gravity, then the work performed appears as gravitational potential energy.
This mgy term is the gravitational potential. In mechanical systems the total energy of the system is the sum of the kinetic plus potential energies.
In a mechanical system on the surface of the earth, work performed on the system (mass) can appear as kinetic or potential energy.
A convenient way of understanding this is to say that work “goes into” kinetic or potential energy. Goes into may be shortened to the one very descriptive word “Goesinto.” As the problems get more complicated you will find this “Goesinto” concept more and more useful.
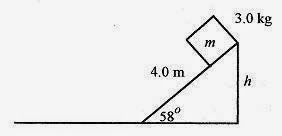
Look first at a block sliding down a frictionless incline plane as shown in Fig. 8 2. The velocity at the bottom of the plane is related to the height, h, from which the block “fell”.
The kinetic energy at the bottom of the plane is mv2 /2, and this must equal mgh, the potential energy at the top of the plane. The gravitational potential energy Goesinto kinetic energy.
For the geometric situation shown in Fig. 8 2, h is 3.4m so
If the block were to continue to slide horizontally along a frictionless surface it would maintain this 8.2m/s velocity.
Frictional Forces
Frictional forces are proportional to the normal force (the force between the two surfaces) and a constant characteristic of the interface (the surfaces involved). They also act to oppose the motion. Frictional forces acting over a distance result in energy lost due to friction. In the example shown in Fig. 8 2, a coefficient of friction between the block and a flat surface at the bottom of the plane would result in energy lost to friction and the block eventually coming to rest. In this problem, potential energy at the top of the incline plane Goesinto kinetic energy at the bottom of the plane, and this energy Goesinto work to overcome friction.
Give the flat surface (Fig. 8 2) a coefficient of 0.20 and the frictional retarding force is
To find the distance the block slides, we can go back to the kinetic energy at the bottom of the plane or the potential energy at the top of the plane. Since there are no losses these numbers must be the same. Take the potential energy at the top of the plane because it is easier to calculate and is original data in the problem. This energy must equal the work due to friction fL or
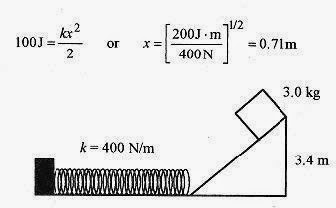
Place a 3.0kg block at the top of a 3.4m high frictionless incline. At the bottom of the incline the block encounters a spring with a constant of 400 N/m on a horizontal surface. No energy is lost to friction. How far is the spring compressed?
Solution: The energy at the top of the plane, which is the same as the energy at the bottom of the plane, Goesinto compressing the spring. The energy at the top of the plane is, from the example problem, 100J so
<><><><><><><><><><><><>
Now complicate problem 8 1 by adding a coefficient of friction of 0.20 for the horizontal surface. How far does the block slide while compressing the spring?
Solution: Now the potential energy Goesinto compressing the spring and overcoming friction. This statement is very helpful in writing the equation. In words, the potential energy, mgh, equals the energy to compress the spring, kx2 /2 plus the work to overcome friction, ìmgx, or
or
Eliminating the units, which are correct, the equation becomes
with solutions
The negative root is inappropriate for this problem. The block compresses the spring 0.70m while sliding on this frictional surface.
<><><><><><><><><><><><>
It is instructive to do a problem first with force analysis and then with work energy analysis. Consider the case of a block sliding down an incline plane with a coefficient of friction. As will become evident, it is possible to do this problem with force analysis techniques. Work energy analysis is, however, conceptually and computationally easier. The force analysis follows the procedures in the chapter on forces.
8 3 Consider the incline plane with friction shown in Fig. 8 4 and calculate the velocity of the block at the bottom of the plane using force analysis, then work energy analysis
Solution: The vector diagram is also shown in Fig. 8 4. The unbalanced force of 20 N acts on the 5.0kg block causing it to accelerate at 4.0m/ s2 down the plane.
The slant height of the plane is 6.0m / sin 35° = 10.5m.
This acceleration over the 10.5 m results in a velocity
Second Solution: Now do the same problem using work energy analysis. When the block is at the top of the plane, the energy is
At the bottom of the plane all of this (potential) energy has gone into kinetic energy (velocity of the block) except for the amount used to do work against the frictional force. The work (against the frictional force) is
The energy “left” after the block has slid down the plane is the 294 J at the top of the plane minus the 84 J lost due to friction, and this energy (= 210J) is manifest in the velocity of the block (kinetic energy). This “energy” is equal to the mv2/2, the kinetic energy of the block at the bottom of the plane.
<><><><><><><><><><><><>
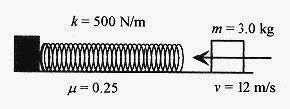
A 5.0kg block sliding at 12 m/s along a frictionless surface encounters a spring of force constant of 500 N/m resting on a surface with coefficient of friction 0.25. The friction surface is only under the spring. What is the maximum compression of the spring?
Solution: Before looking at Fig. 8 5 try drawing the diagram yourself. On exams you sometimes have to work a problem from only a word description. As you are working through these problems practice drawing the diagrams, and do not be hesitant in using several diagrams. Notice the previous problem where the system was depicted with the vector diagram beside it. Sketching the system and all the vectors on a single diagram is unnecessary confusion. Use multiple diagrams and reduce the clutter.
When the block encounters the spring and the friction surface all the energy is kinetic, mv2 /2. This energy Goesinto compressing the spring and sliding along the friction surface. In equation form
Be careful in writing this statement. Many people write the kinetic energy correctly, the energy to compress the spring correctly, and then write the force to slide along the friction surface. Be aware of this mistake and check the units to be sure the units on one side of the equation are the same as the units on the other side of the equation. You may have noticed that in all problems the first equation is written with units just as a reminder that checking units will reduce the number of errors. It is very frustrating to make a simple mistake that could have been avoided had units been checked in the problem before proceeding with the numerical calculations. Putting in numbers
The units are correct so without units the equation is
and
This is the amount the spring is compressed as the block comes to a stop.
<><><><><><><><><><><><>
For the situation described in problem 8 4, find the velocity with which the block leaves the spring.
Solution: Start this problem with the spring compressed the 0.91m. At this point the potential energy stored in the spring is used to push the block 0.91m back across the friction surface. The energy remaining as the block leaves the spring is the original kinetic energy less the energy expended in two 0.91m trips across this friction surface. In word equation form this is
This is a Goesinto problem. Initial KE Goesinto two trips across the friction surface plus the final KE.
The total work performed in the block moving across this surface is
The velocity of the block as it flies off the spring is
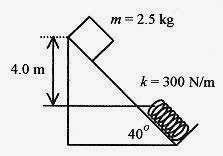
Go back to an incline plane problem (similar to problem 8 3) but with the addition of a spring with a force constant of 300 N/m placed at the bottom of the incline, as shown in Fig. 8 6. Calculate the compression of the spring when the block slides down the plane.
Solution: An approximate answer can be obtained by setting mgh, where h is the vertical distance from the block to the spring,
the compression of the spring. Fig. 8 6 The compression of the spring gives the block a little more potential energy so a more correct statement would take into account this “additional height,” h.
Eliminating the units and cleaning up the numbers yields a quadratic
with solution
This value is somewhat larger than calculated above as expected, due to the “additional height.”
The procedure of finding an approximate solution and then refining it to the exact solution is very helpful in attacking problems. First you get an approximate solution that is close to the exact one, and second you educate yourself as to how to proceed to the exact solution. This procedure is used in the last problem in this chapter.
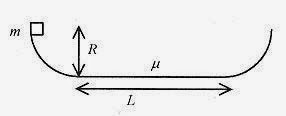
8 7 Place a mass on a track made up of a flat section, L, with coefficient of friction, ì, and two frictionless semicircular surfaces of radius R. Let the mass start from the top of one of the semicircles and calculate where it comes to rest.
Solution:
The initial potential energy is mgR. When the mass encounters the friction surface, this (potential) energy is dissipated in doing work to overcome friction. Assuming the energy lost due to friction in one traverse is less than the initial potential energy, the mass will rise to a height (on the opposite semicircle) R’ dictated by the energy statement
After another traverse of the flat portion of the track the height will be dictated by
and so on until all the original potential energy is dissipated.
<><><><><><><><><><><><>
8 8 For the track shown in problem 8 7 take ì = 0.10, R = 1.0m, and L = 2.0m and find where the mass stops.
Solution: The only way the mass loses energy is by sliding along the flat “friction” part of the track. The initial potential energy Goesinto frictional work.
The mass crosses the friction area 5 times and ends up at the edge of the friction surface opposite from where it started.
<><><><><><><><><><><><>
8–9 Consider a 10gm bullet passing through a 3.0kg block resting on a table. The velocity of the bullet is 400 m/s on entering the block and 250 m/s on exit. Calculate the energy lost by the bullet in passing through the block.
Solution: The energy is all kinetic, so calculating before and after
The kinetic energy lost or ÄKE = 488J.
<><><><><><><><><><><><>
8–10 If 30% of the energy lost in problem 8–9 is available to move the block along a surface with coefficient of friction 0.80, how far will it move?
Solution: Equate the energy available to the work performed
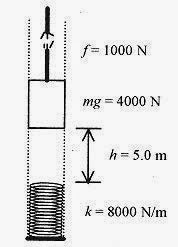
8–11 Consider an elevator weighing 4000N held 5.0m above a spring with a force constant of 8000 N/m. The elevator falls onto the spring while subject to a frictional retarding force (brake) of 1000 N. Describe the motion of the elevator.
Solution:
The potential energy of the elevator with respect to the top of the spring is
When the elevator falls, this energy, less the energy lost due to the frictional brake, is available to compress the spring.
The friction brake stays on while the elevator is in contact with the spring so this energy Goesinto kx2 / 2 + fx. In equation form this is
This expression is only approximately correct since if the spring is compressed a distance x, then this mgx provides an additional amount of potential energy. The correct equation that determines the compression of the spring is
Putting numbers into the equation
The units are correct so 4000×2 3000x 15000 = 0 or 4×2 3x 15 = 0 which has solution
The energy stored in the spring at this (compressed) point is
This amount of energy will raise the elevator and do work against friction according to the equation
This places the elevator 2.1m above the spring.
This analysis can be repeated until the elevator comes to a stop. As an exercise perform one more “bounce” of the elevator starting at 2.1m above the spring.
The elevator will continue to bounce up and down off the spring until the weight of the elevator is equal to the force
of the spring plus the frictional force so the elevator comes to rest with the spring compressed 0.38m.