Apparent Weight
Apparent weight problems usually are encountered in the discussion of force. Though relatively easy, they cause problems for some people. This short chapter should give you enough background and examples to show you how to do apparent weight problems.
One of the first points to get straight is the difference between mass and weight. Mass is that property that makes different objects accelerate differently when equal forces are applied. Operationally mass is the m in the equation F=ma. Mass is measured in kilograms or slugs. Weight in equation form is
and is the force something exerts. Weight is measured in Newtons or pounds. Popular usage scrambles weight and mass. Gold and silver are usually sold by the gram or kilogram while nails and chicken feed are sold by the pound.
The first step in keeping this all straight is to remember that weight is force. A scale that measures weight in pounds or Newtons is just a force meter. Tension in a rope is a force.
A person of 80kg mass standing on the surface of the earth is subject to the force of gravity that acts between the 80kg person and the mass of the earth. This force is expressed as an acceleration due to gravity. On the earth, this acceleration is 9.8m/s2. The force on the person, also called weight is
A force meter placed between the person and the earth would read 784 N.
To understand apparent weight take a “thought trip” (based on experience) on an elevator. Imagine a force meter between you and the floor of the elevator. As you step on the elevator the force meter reads the same as when you are standing on the ground. As the elevator accelerates upward, the force meter registers higher, reading maximum at maximum acceleration. As the acceleration decreases and the elevator assumes a constant velocity (upward) the force meter reads the same as when the elevator was not moving. This constant velocity condition is equivalent to forces in equilibrium, so that the only thing contributing to the reading of your force meter is the mg due to the earth. As the elevator slows, by decelerating, the force meter reads less than the force for constant velocity. As the elevator comes to rest at a higher level, the force meter again reads the same as when you were at ground level.
Let’s go back over this elevator ride and put in some numbers. At zero or constant velocity the force meter reads 784 N. If the elevator were accelerating upward at 2.0 m/s2, then the force meter would read
If the elevator were accelerating downward (or decelerating near the top of its trip up) at 3.0m/s2, then the force meter would read
If the elevator were in free fall, then the force meter would read zero. The acceleration acting on the elevator is the same as on the person.
6 1 A 12 kg flower pot is hanging by a cord from the roof of an elevator. What is the tension in the cord when the elevator is stationary and when it is accelerating upward at 3.0m/s2?
Solution: When the elevator is stationary the tension in the cord is
When the elevator is accelerating upward the tension in the cord is increased by an amount equal to ma
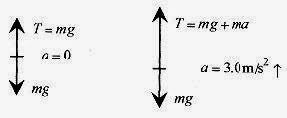
Second Solution: Now take another, more analytical, look at the problem. When the elevator is at rest the tension in the cord equals mg, so writing a force statement, T mg = ma where a is zero, produces T = mg.
Fig. 6 1
When the elevator is accelerating upward at 3.0m/s2 the T mg = ma equation has an acceleration. The hard part of doing the problem this way is keeping the algebraic sign of the acceleration correct. Looking at Fig. 6 1, T and a have the same sign so write
If you use this vector approach to doing apparent weight problems be especially careful of the signs. However you do the problems, go through a little thought experiment to make sure you have the signs right.
Another difficulty with the vector approach to apparent weight has to do with the interpretation of the vector diagram (on the right in Fig. 6 1). If you are an observer outside the elevator you observe an acceleration of the flower pot, as the analysis indicates. If, however, you are riding in the elevator you observe no acceleration of the flower pot because you are riding in the accelerating reference frame.
6 2 A rope (fastened at the top) is hanging over a cliff. What is the tension in the rope with a 70kg mountain climber sliding down the rope at a constant acceleration of 6.0m/s2.
Solution: Performing a little “thought experiment,” the tension in the rope is less than if he were at zero velocity or sliding at constant velocity. In this instance the 6.0m/s2 must be subtracted from the 9.8 m/s2.
When in doubt as to whether to add or subtract the acceleration from g, look to the extreme situation. In this problem the person sliding down the rope at constant velocity would produce (9.8m/ s2)70kg=686N of tension in the rope. If the person were in “free fall,” that is accelerating at 9.8m/s2. he would produce no tension in the rope. Thus, any acceleration down the rope should be subtracted from the 9.8m/s2.
6 3 What is the tension in a rope with the 70kg mountain climber of problem 6 3 accelerating (climbing) up the rope at a constant acceleration of 1.0m/s2.
Solution: In this case the tension in the rope is increased by ma.
6 4 A 100kg astronaut produces a force (weight) on the surface of the earth of 980 N. What force (weight) would the astronaut produce on the surface of the moon where the “g” is about one sixth of the g on earth?
Solution: The force or weight would be
<><><><><><><><><><><><>
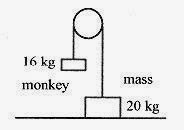
6 5 A 16kg monkey wishes to raise a 20kg mass by climbing (accelerating) up a rope that passes over a pulley attached to the mass.How much must the monkey accelerate up the rope in order to raise the mass?
Fig. 6 2
Solution: The mass produces a force or tension in the rope of
The mass of the monkey hanging on the rope produces a force of
To just balance the mass the monkey must accelerate up the rope to produce a force of (196 157) N = 39 N.
At this acceleration, the tension in the rope is 196N.
To raise the mass, the monkey must accelerate at a rate greater than 2.4 m/s2.
Any acceleration greater than 2.4 m/s2 will increase the tension in the rope by an amount equal to the “additional” acceleration times the mass of the monkey.