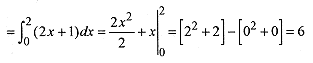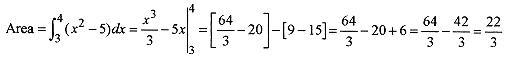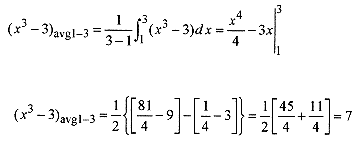Integrals
Integrals can be viewed two ways, as the area under a curve or as the inverse operation to the derivative. Look upon the derivative as an operation performed on a function. If y = 3×2 + 2x 1 is the function, then the derivative is d by dx of y or
The inverse of this operation is called integration. The actual operation of integration is seen by writing the d/dx operation as a total derivative dy = (6x + 2)dx, and the integral is
so =6x/2+2x+const=3x+2+const the original function plus a constant.
Just as the derivative of a power function y = cvn is dy = cnvn 1dv the integral of
The constant is necessary because constants are lost in differentiation! Evaluating the constant requires some knowledge of the physical problem.
The other definition of the integral is as the area under a curve. This definition is most convenient in many physical problems, especially those involving work. The integrals of several curves are done below. The integrals are represented by the area under the curves between two specific values.
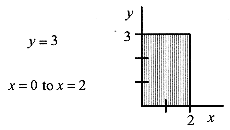
The area under the curve is
A quick glance at the graph of y = 3 confirms this calculation.
The area under the curve is
The shaded area consisting of a rectangle and triangle is equal to 6.
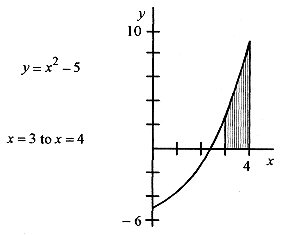
As an exercise approximate the area under the curve. The area between x = 3 and x = 4 can be approximated with a rectangle and triangle. Find the value of y at x = 3 and the area of the rectangle. Find the value of y at x = 4 and find the approximate area of the triangle. These two areas are very close to the area found from the integral.
The integrals of these three curves are what is known as definite integrals, ones that have specific limits. As such they do not need constants. The indefinite integrals, those without limits, need the constant. Listed below are the integrals of some common functions.
Average Value of a Function.
In Fig.I 18 the shaded area is the value of the integral. This area could be represented by a rectangle with one side of the rectangle equal to 1, the length of the integral, from 3 to 4, and the other side, the average height of the function between 3 and 4. This average height is the average value of the function over the interval from 3 to 4. From the geometry then we can say that the average value of the function times the length of the integral equals the area or value of the integral. Rearranging then, the average value of a function over a particular range is the value of
the integral over the range divided by the range. Applying this to the function y = x2 5 the average value of the function between 3 and 4 is
At x = 3 the function has value 4, and at x = 4 it has value 11, so 22/3 is a reasonable value for the average.
Likewise, the average value of the function y = x3 3 between 1 and 3 is
