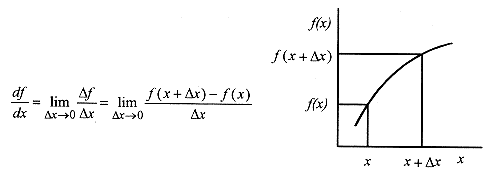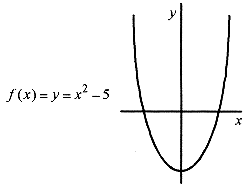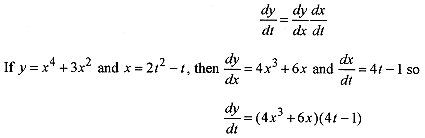Derivatives
There are numerous definitions of the derivative, but the one that fits most physics problems best is that the derivative of a function is another function that gives the slope of the original function at any point. Consider a function f(x), often written as y = f(x), over an interval äx. The notation y = f(x) is mathematical symbolism that says “a variable y is going to be described by certain operations on another variable x.”
Using the ä notation the general expression for slope is
This equation says that the slope of a function is the value of the function at a point x + ƒÂx minus the value of the function at x all divided by the ƒÂx. This assumes the function is linear between x and x + ƒÂx; an approximation that gets better as ƒÂx gets smaller. The slope defined this way is an average slope between x and x + ƒÂx. The derivative is the general expression for the slope at any point, thus, it is a function that gives the slope of another function at every point. The derivative, df/dx or f’ is the limiting case of the slope where ƒÂx ¨ 0
Now apply this procedure to several functions.
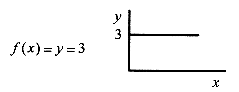
The function is a constant so f(x + δx) = f(x) and the slope is zero as is evident from the graph.
The slope of the curve y = x2 – 5 is 2x. Just pick a value of x, and the slope is two times this value.
As an exercise verify that the derivative of y = x3 is 3×2.
The derivative of power law functions is very easy with the procedure described above. After performing a few of these, we can come to the conclusion that for any power law y = cvn, the general expression for the slope (derivative) is y’ = cnvn 1. Listed below are the derivatives for power laws as well as some trigonometric, exponential, and logarithmic functions. All of these can be derived using the procedures employed above.
One other useful rule of differentiation is the chain rule. If y is written in terms of x and x is written terms of t, it is possible to write dy/dt through the simple expediency of a chain derivative.
and since x is written in terms of t, the derivative dy/dt can be written in terms of x or t.