Operation of a Synchronous Generator in a Large Power System
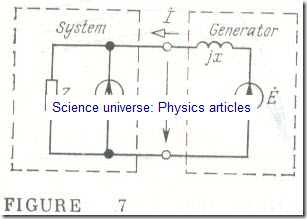
A large three-phase power system combines a great number of sources and of three-phase loads operating in parallel. It may be taken that a minor change in the number of sources and loads will not affect the operation of a large power system. Therefore, the rms voltage across the system busbars and the system frequency may be assumed to be constant. An equivalent circuit per phase of a large system comprising an infinite-power source Ė and the system load Z load is shown in Fig 7. The figure also shows an equivalent circuit for a phase of a synchronous generator, neglecting the phase-winding resistance connected to the system busbars .
On neglecting the resistance of a phase winding, the electric state per phase of a synchronous genera tor may be described by the following equation:
Ė0 = Ė + jİ = V̇ + jİ (15.8)
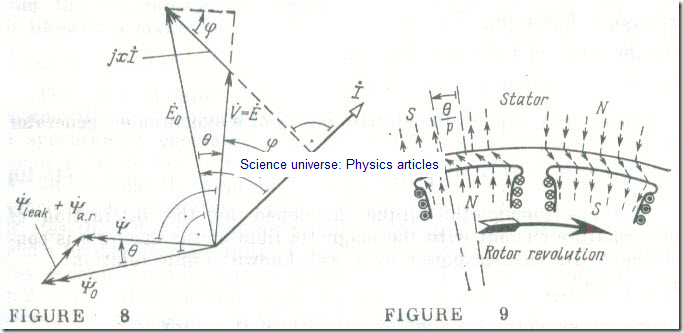
The events taking place in a synchronous genera tor connected to a large power system are illustrated in the phasor diagram of Fig 8. It is convenient to begin constructing this diagram by directing the phasor of the large-system terminal voltage, Ė = V̇ , upward along the axis of ordinates. This voltage is balanced by a part of the stator phase emf E0 induced in the stator winding by the flux linkage Ψ̇0 . Adding jİ which is at right angles to İ to the phasor V̇ yields the Ė0 phasor. On the ph as or diagram, Ψ̇0 is in quadrature leading with the phasor of the emf Ė0 it induces. Since
jİ = – Ė leak – Ėa.r
that is, equal to the sum of the emfs induced by the leakage flux linkage and the armature reaction flux linkage, it follows that the equation defining the electric state per phase of a synchronous generator; Eq. (15.8), may be re-written as
Ė0 + Ėleak + Ėa.r = V̇ = Ė
The phase voltage of a synchronous generator is equal to the sum of the emfs induced in each phase winding by three flux linkages, namely Ψ̇0, Ψ̇leak, and Ψa.r , Physically, however, these three flux linkages form a single resultant flux linkage with the phase winding
Ψ̇= Ψ̇0 + Ψ̇leak + Ψ̇a.r
In consequence, it may be thought that the terminal voltage per phase of a synchronous genera tor is equal to the emf induced by the resultant flux linkage Ψ̇ with a phase winding. This determines the direction of the Ψ̇ phasor which must be in quadrature leading with the phasor V̇ = ̅Ė . The phasors Ψ̇leak and Ψa.r are in line with the phasor İ . The phase angle θ between Ė0 and V̇ is equal to that between Ψ̇0 and Ψ̇. It is customary to reckon the phase angle θ from the phasors Ė0 and Ψ̇0 For a synchronous machine in the generating mode this angle is always less than zero (θ < 0).
The phase angle θ between the flux linkage phasors corresponds to a mechanical angle of θ/p between the axes of the rotor poles and the direction of the resultant magnetic field in a synchronous generator (Fig 9).
As follows from the phasor diagram, the rms value of the resultant flux linkage with a phase winding of a synchronous generator connected to a large power system with ( V = const ) is a constant quantity ( Ψ = const), independent of load.