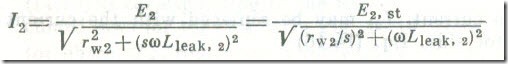The Equivalent Circuit for a Phase of an Induction Motor

The Equivalent Circuit for a Phase of an Induction Motor

In calculating the process taking place in an induction motor, resort is usually made to the equivalent circuit of one phase. This circuit is assumed to include fixed resistive and inductive elements and also a variable resistive element to represent the mechanical load applied to the motor shaft.

The difficulties. in developing such an equivalent circuit arise because, firstly, the frequency of the stator phase currents is f and that of the rotor phase currents is f ₂ = f _s , secondly, the stator phase has w₁ turns and the rotor phase has w₂ turns, thirdly, the two windings differ in the winding factors kw₁ and kw₂ and fourthly, the stator has m_l = 3 phases and a squirrel-cage rotor has m₂ = N pha­ses. Therefore, all rotor phase quantities must be referred (or trans­ferred) to the respective stator quantities.

To begin with, we will refer the rotor phase quantities to the sta­tor phase frequency. To this end we consider an equivalent stationa­ry rotor such that the rotating magnetic field of the motor remains unchanged and corresponding to the operating conditions. Since the rotating magnetic field remains unchanged, this implies that the energy transferred from the stator to the revolving rotor in opera­tion is the same as that transferred to the equivalent stationary rotor.

Since the rotating magnetic field remains constant, the rms value of the emf in the phase winding of the equivalent rotor is given by the same equation as the rms value of the emf in the stator phase winding in operation, Eq. (14.11 b), so, subject to Eq. (14.14), it is

E_2,st = 4.44fw₂k_w2Φ_r = E₂/s                                               (14.17)

where, for a squirrel-cage rotor, w₂ = 1/2 and k_w2 = 1.

The term E_2st is the rotor phase emf referred to the stator fre­quency. It is one of the key quantities used in the design of induc­tion motors, and its value is about twice that of the rms emf induced actually in a phase winding of the stalled rotor. This is because in the latter case the stator phase current is about 6.5 times the rated value, so that the voltage drop Z_w1I_l across it increases, and there is a decrease in the magnetizing current, Eq. (14.11a), which excites the rotating magnetic field, Eq. (14.11b).

For the rotating magnetic field to remain unchanged when the spinning rotor is replaced with a stationary one, it is necessary that. the currents in their phases, which have frequency f₂ = f s in the former case and frequency f in the latter, be the same in amplitude and phase shift relative to the emfs that induce these currents. This is achieved by referring the phase current of the spinning rotor to the frequency f of the stationary rotor. In view of Eq. (14.17), the phase current of the spinning rotor in Fig 15 may be written as

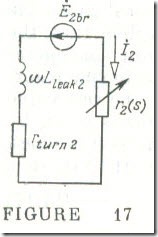
The right-hand side of the above equality corresponds to the equivalent circuit (Fig 17, in which Ė_2br= Ė₂,st) of a phase of the equivalent stationary rotor, where the current frequency is f , and the term r_w2/s is represented by the sum of the rotor phase winding resistance r_W2 and some additional resistance r₂ (s) which is many times r_W2 .

As follows from comparison of the equivalent phase circuits for the spinning and the stationary equivalent rotor, the current in them lags behind the emf by the same angle

Thus,

for design purposes an operating induction motor may be replaced with an equivalent stationary motor in which each rotor phase is shorted by a resistor

r₂ (s) = r_w2 (1 – s)/s

The power dissipated in this resistor, r₂I²₂ , is equal to the mechanical power developed by the rotor in one phase.

Now we can refer the phase quantities of the equivalent rotor to the number of turns, the winding factor and the number of stator phases in much the same way as the secondary quantities of a transformer are referred to the primary turns (see Sec. 8.5).

The stator phase emf E₁ is connected to the phase emf of an equi­valent stationary rotor, E_2,St , by a relation of the form

E₁ = (w₁k_w1/w₂k_w2) E_2.st = k_eE_2.st

where Ire is the voltage transformation ratio of an induction motor.

As follows from the equivalent circuit (see Fig.17) for a phase of a stationary rotor

– Ė_2.st = (r_w2/s + jɷL_1eak,2) İ₂

= (r_w2 + r₂ + jɷL_leak,2) İ₂ = Z_w2İ₂+ r₂İ₂

Therefore,

– Ė_l = (Z _w2 + r₂) k_eİ₂

The rotor phase current, İ₂ , may be replaced with the current referred to. the stator side, Eq. (14.16)

İ₂ = (3_w1k_w1/m₂k_w2) İ’₂ = k_iİ’₂

where k, is the current transformation ratio of an induction motor.

On substituting, we get

Ė₁ = – (Z_w2 + r₂) k_ek_iİ’₂

The product k_ek_i = k is the transformation ratio of an induction motor .

Now we will introduce the rotor circuit impedance and resistance referred to the stator side into the equation of electric state for a sta­tor phase of an induction motor

k_ek_iZ_w2 = Z’_w2 and k_ek_ir₂ = r’₂

In the stator phase circuit these elements would draw the same power and with the same phase angle between current and voltage as they do in the rotor phase circuit shown in Fig. 17.

Thus, the stator phase emf is

Ė_l = – (Z’_w2 + r‘₂) İ’₂

and the stator phase voltage, in accord with Eq. (14.11a), is

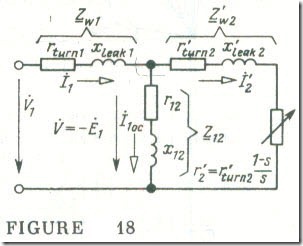
V̇₁ = – Ė_l + Z_w1İ₁ = Z_w1İ₁ + (Z’_w2 + r‘₂) İ’₂                                          (14.18)

On the other hand, Ė_l is proportional to the magnetizing current İ_l,_oc . So, for the same reason as for a transformer .

– Ė_l = Z₁₂ İ_l,_oc

where the term Z₁₂ whose magnitude has the dimensions of ohms, re­presents in the equivalent circuit the magnetic circuit of the motor. Hence, the stator phase voltage may alternatively be defined as

V̇₁ = Z_wl İ₁+ Z₁₂ İ_l,_oc                                                    (14.19)

Along with the stator current equation

İ_l = İ_l,_oc + İ’₂                                                          (14.20)

we may regard Eqs. (14.18) and FIGURE 14.18 (14.19) as corresponding to the Kirchhoff laws for the circuit in Fig. 18. Here, Z’_w1 represents a stator phase, Z’_w2 represents a rotor phase, Z₁₂ represents the magnetic circuit of the machine, and r~ represents mechanical load.

In accord with Eq. (14.16), at synchronous speed the resistive element representing the mechanical load in the equivalent circuit is

r‘₂ = r‘_w2/s – r‘_w2 = r‘_{w2 (1 –} s )/ s = ∞

If we bring the motor to a complete slop, then s = 1 and r‘₂ = 0 .

For this reason the test in which a motor is brought to a complete stop (the blocked-rotor test) may be called a short-circuit test since it is usually carried out at a very low stator voltage.