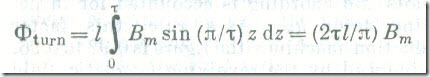The Equation of State for a Stator Phase of an Induction Motor

The Equation of State for a Stator Phase of an Induction Motor

The emf induced in each turn of the stator winding by the revol­ving magnetic field, is, in accord with Eq. (2.2), given by

e_turn = – dΨ/dt

Since it is legitimate to assume that the normal component of the magnetic induction is distributed sinusoidally around the cir­cumference of the rotor, the flux linkage of the turn as the field re­volves will also vary sinusoidally with time

Ψ = Φ_turn sin ɷt

.Therefore the rms value of the emf induced in a stator turn can be found from the equation for transformer emf, Eq. (7.2c)

E_turn = 4.44/ Φ_turn

In contrast to a transformer, however, Φ_turn symbolizes the avera­ge (constant) rather than peak value of the revolving magnetic flux threading the turn

Φ_turn = Ɩ ∫₀ ^τ B_m sin (π/τ) z dz = (2πƖ/π) B_m

where Ɩ is the rotor length and τ is the pole pitch.

‘When defining the emf, e₁, induced in each of the stator phase win­dings, it should be remembered that this emf is induced by a revol­ving rather than an alternating magnetic field. Usually the re­quisite number of turns in the stator winding consists of several coils located in q adja­cent slots rather than of one coil each side of which is located in one slot. These coils are connected in series to form a coil group (see Fig. 1 c). The angular width of a tooth in the stator core determines the central angle between two adjacent slots or the slot angle in mechanical degrees

β = 360^o/z

where z is the number of stator-core teeth or, which is the same, the number of stator-core slots.

The revolving magnetic field cuts the coil sides at different ti­mes, so there is a phase difference between the emfs induced in the adjacent coils of the same coil group. This phase angle a is p times the slot angle β in mechanical degrees

a = pβ = p X 360^o/z                                   (14.9)

Hence, each time a 2p-pole field rotates through 360⁰ mechanical the phase of the induced emfs changes by p X 360⁰ electrical. Be­cause of this phase angle between the emfs e_c induced in the coils of a coil group, the rms emf of a coil group, E_IC is smaller than the product of the rms emf in one turn, E_turn, by the number of turns, w_1C in a coil group (Fig. 12) .

E_1C < E_turnw_1c

This reduction in emf is thus caused by the distribution of coil sides among many slots. A second cause of the reduction in the winding emf is chording or the use of short-pitched coils in which the angular distance between the two sides of a stator-winding turn is less than the pole pitch τ . Chording is ordinarily used in order to reduce the length of the coil-overhang connections. As a result of chording, a stator turn links less than all of the pole flux, so a smaller emf is induced in it.

The reduction in the induced emf due to the distribution of the stator winding in several slots and chording is accounted for in de­sign by applying the winding factor, k_w . As a rule, this factor is less than unity. For induction machines the figure is 0.92 to 0.96.

Therefore, the rms emf induced by the revolving magnetic field in each phase winding with w₁ = w_1cP turns is equal to

E₁ = _PE_1c = w₁kw₁E_f = 4.44fw₁k_w1 Φ_f                                        (14.10)

It should be noted that the winding factor and the phase angle between induced emf apply not only to induction machines, but also to all other machines which utilize a revolving magnetic field in one form or another.

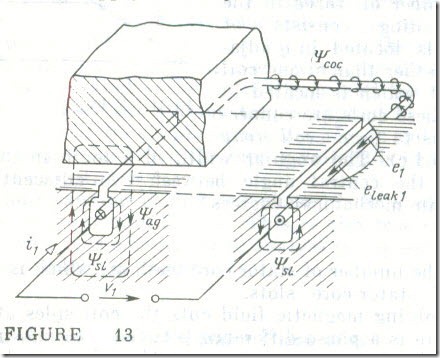
In addition to the air-gap flux crossing the clearance between the stator and the rotor and linking both windings, there is also a flux which avoids the rotor. It is called the leakage flux, and the flux linkage associated with it is called the leakage flux linkage, Ψ_leak,1 . As is seen from Fig. 13 where a stator phase winding is shown as a single-turn coil, the stator leakage flux linkage is the sum of the flux linkages across the core slots, Ψ_sl , around the coil-overhang conne­ctions, Ψ_coc , and across the air-gap between the stator and rotor, Ψ_ag , The stator leakage flux linkage increases with increasing air­gap, increasing length of coil-overhang connections, and increasing slot depth. Since most of the path for the magnetic flux is through air, we may take it, as we did for a transformer, that the stator lea­kage flux linkage is directly proportional to the stator current i₁ and is in phase with that current.

In each phase winding of the stator the leakage flux linkage in­duces a leakage emf e_leak.l in accord with Eq. (2.2). This emf is aligned with the stator current i_l (see Fig. 13). Referring to Fig. 13, e_l is the emf induced by the revolving magnetic field in each phase winding, and VI is the phase voltage of. the supply line.

Thus, the current in each phase winding may be looked upon as produced by the joint action of the phase supply voltage and two emfs, one induced by the revolving field and the other induced by the leakage flux.

On representing all sinusoidal quantities in complex form and using Kirchhoff’s voltage law, we may write for a stator phase circuit

V̇₁ + Ė₁ + Ė_leak,1 = r_turn,1 İ₁

where r_turn.l is the resistance of the turns in a stator phase winding.

On the basis of Eqs. (2.32) and (2.33), the emf, – Ė_leak,1 needed to balance the stator leakage emf may be written as

– Ė_leak,1 = j_leak,1, İ₁ = jɷL_leak,1 İ₁

where – L_leak,1 = Ψ _leak,1 / i₁ is the leakage inductance of the stator phase, and _leak.1 is the leakage inductive reactance in the stator phase winding.

Hence, an equation of electric state for a stator phase may be writ­ten as

V̇₁ = (-Ė_l) + (r_turn.1 + j_leak,1) İ₁ = (-Ė_l)+ Z_w1 İ₁                                    (14.11a)

where

Z_w1 = r_turn,1 + j_leak.l

is the complex impedance of a stator phase winding.

Equation (14.11a) does not differ from that of electric state for the transformer primary, Eq. (8.11a) which is quite natural because in an induction machine energy is transferred from the primary (sta­tor) circuit into the secondary (rotor) circuit by induction as this is done in a transformer.

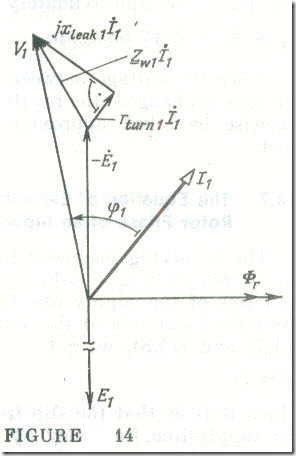
Graphically, the equation of electric state for a stator phase of an induction motor is illustrated in the phasor diagram of Fig. 14

where Z_w1İ_l is substantially greater than it counterpart is in the equation of the transformer primary. This results from the existence of an air gap in the magnetic circuit of an induction machine (Fig. 14).

In medium and large induction machines, the voltage drop Z_w1I₁ at I₁ ≼ I_1,rtd is relatively small, and V₁ may, in accord with Eq, (7.2c), be approximately written as

V₁ ≈ E₁ = 4.44 fw_fk_w1Φ_r                                         (14.Hb)

Since the voltage between the terminals of a phase winding, V₁ remains unchanged, the revolving magnetic flux Φ_r in operation may likewise be taken approximately as constant and independent of load.