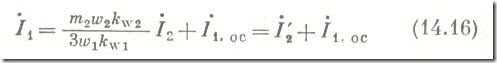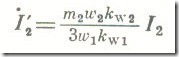The Equation of Electric State for a Rotor Phase of an Induction Motor

The Equation of Electric State for a Rotor Phase of an Induction Motor

The revolving magnetic field induces in a rotor phase an emf, e₂, at frequency f₂ = P (n_l – n)/60. We can express this frequency in terms of the supply-line frequency on dividing and multiplying the right-hand side of this expression by n₁ . Then. in view of Eqs. (14.7) and (14.8). we get

f₂ = fs                                    (14.12)

which tells us that the slip frequency, f₂ is equal to the product of the supply-line frequency by the slip.

The emf in a stator phase winding, e₁. and the emf in a rotor phase winding. e₂ are induced by a revolving magnetic field which is common to the two windings and is produced by the joint action of the stator current it and the rotor current i₂ . However, e₁ opposes a change in it. while e₂ induces i₂ Therefore, while the positive direc­tions of e_t and it are the same (see Fig. 13), it is convenient to assume that the positive direction of i₂ is opposite to that of e₂ . This corresponds to the connection of the stator and rotor phase win­dings in opposition so that the resultant mmf of the motor is the dif­ference between the stator mmf and the rotor mmf. This is also true of the primary and secondary emfs , currents and mmfs of a transfor­mer (see Fig. 5 b).

The rotor phase current i₂ sets up a magnetic field a part. of which avoids the stator winding. This part is responsible for the leak­age flux linkage of a rotor phase winding, Ψ_{leak,2 .}

Thus. the equation of electric state in complex form for a rotor phase is

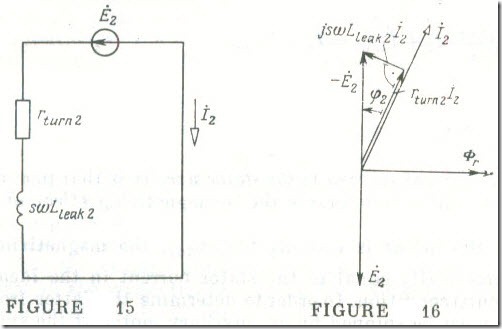
– Ė₂= r_turn.2İ₂ + jsɷL_1eak,2İ₂                                                 (14.13)

where r_turn.2 is the resistance of the rotor turns, L_leak.2 = Ψ_leak,2 /i₂is the leakage inductance of a rotor phase and sɷL_leak.2 is the induc­tive reactance of a rotor phase.

By analogy with Eq. (14.11b), the rms value of the rotor phase emf is

E₂ = 4.44fsw₂k_w2Φ_r                                                           (14.14)

where for a squirrel-cage rotor w₂ = 1/2 and kw₂ = 1 .

The equivalent circuit diagram of a rotor phase answering Eq. (14.13) appears in Fig.15, and the cone ponding phasor diagram is shown in Fig.16.

It is to be noted that Eq. (14.11a) and Eq. (14.13) are similar to Eq. (8.11a) describing the electric state of a transformer primary and Eq. (8.11b) describing the electric state of a transformer secondary in a short-circuit condition, that is, at V̇₂ = 0 .

The Balance of MMFs in an Induction Motor

The revolving magnetic field set up by the stator of an induction machine remains stationary relative to that produced by its rotor. Therefore, with the rotor spinning, the mmfs produced by the stator and rotor currents may be treated as phasors which, when combined vectorially , give the mmf exciting the revolving magnetic field of the machine. In calculating these mmfs, it is important to remember that they are produced by currents in the coil sides that are distribu­ted among several slots and are out of phase in space. This is accoun­ted for by applying the winding factor which usually is less than unity.

Thus, by analogy with the mmf balance for a transformer, Eq. (8. t,), the mmf balance for an induction motor, with its rotor sta tion­ary or spinning, is

3w₁k_w1İ₁ – m₂w₂kw₂ İ₂ = 3w₁k_w1İ_1,oc                                         (14.15)

Here we have accounted for the fact that the resultant mmf for a three-phase stator and rotor winding is 3/2 times that given by Eq, (14.4), and that the resultant mmf of an m₂-phase winding is m₂/2

times that of a single phase mmf.

On the basis of Eq. (14.15), the stator current may be expressed as

where

is the rotor current referred to the stator side. It is that part of the sta­tor current which counteracts the demagnetizing effect of the rotor current.

When the motor is running (s ≼ s_rtd), the magnetizing current İ_1,oc is practically equal to the stator current in the ideal no-load (open-circuit) condition. In order to determine the latter from a test, the rotor must be spinned by an auxiliary motor at the synchronous speed which is the speed of the rotating magnetic field (s = 0). In the circumstances, ‘the rotor current becomes equal to zero. Thus, with no load torque applied to the shaft, the open-circuit (no-load) current of an induction motor exceeds the ideal no-load (open-circuit) current due to the loss of power through friction in the rubbing parts, as heat in the winding rotor, etc.