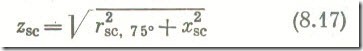The Short-Circuit Condition of a Transformer

The Short-Circuit Condition of a Transformer

This condition may arise as a result of a fault fraught with grave consequences (overheating and break-down), or it may be produced for testing purposes in which case it is called the short-circuit (or load loss and impedance) test.

The short-circuit test is carried out by short-circuiting the secon­dary winding and applying a low voltage to the primary winding sufficient to cause the rated full-load current, I₁,_sc = I_1.rtd , to flow. The objective of the test (Fig. 15) is to measure the power dissipated in the conductors, the impedance voltage, etc. It is one of the mandatory tests applied at the maker’s works to a finished transformer .

In the short-circuit test when V₂ = 0, the emf E₂,_sc induced in the secondary winding is, as follows from Kirchhoff’s voltage law, Eq. (8.11b), equal to the sum of the voltages across the resistance

and inductive leakage reactance of the secondary winding

Ė ₂,_sc = – (r₂ + j_leak.2) İ₂,sc

while under the operating conditions

Ė ₂ = – (V̇₂ + r₂ İ₂ + j_leak,₂İ₂)

The primary voltage applied in the short-circuit test, V_l,_sc, in order to cause a full-load rated current, I_l,_sc = I_l,_rtd, to flow amounts approximately to 5-10 % of the rated primary voltage, V_l,_rtd . Therefore the rms secondary emf, E_2.sc , is a mere 2 % to 5 % of the rms emf, E₂, under normal operating conditions. The magnetic flux in the core, Eq. (8.1h), decreases in proportion to the emf , and so do the magnetizing current and the core losses which are proportional to Φ². In consequence, it is legitimate to think that during a short-circuit test all of the primary power in the transformer, P_l,_sc, is equal to the copper (conductor) losses in the primary and secondary win­dings

P_1,sc= r₁I²_1,sc+r₂I²₂,_sc= r₁I²₁,_sc+ r’₂I’₂², sc

The magnetizing current I₁,_oc is negligibly small, therefore, by Eq. (8.10a), I_l,_sc = I‘_{2,sc ,} and

P_1,sc= (r₁+r’₂)I²₁,_sc= r_scI²₁, sc (8.14)

As the apparent power rating, S_rtd, of a transformer is increased, the short-circuit active power, P_1,sc, goes down in relative terms. For S_rtd = 20-5 KV A, the ratio P_1.sc/S_rtd is 3-3.7%, while for S_rtd = 320-5600 kV A, the figure is 2-1 %.

Using the value of load loss found in a short-circuit test, P₁,_se, and the full-load rated current I_l,_sc = I_1,rtd, we can, on the basis of Eq. (8.14), determine the short-circuit resistance of the transformer

r_sc = P_1,sc/I²_1,sc (8.15)

To keep the size of a transformer to a reasonable minimum, the designer has to choose the current density in the conductors and the magnetic flux density (magnetic induction) in the core such that the temperature rise of the transformer will remain within the safe limits. For this reason, in finding the load loss, the value of r_sc found by a short-circuit test must he re-scaled to a temperature of 75°C (see Table 1.2).

The short-circuit inductive reactance of a transformer may be deem­ed independent of temperature. Therefore, it is found directly from the results of a short-circuit test

Hence the short-circuit impedance of a transformer, as re-scaled to an operating temperature of 75°C, is

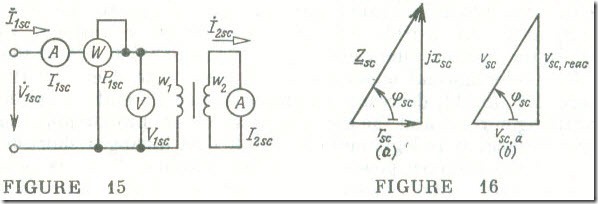
Figures 16 a and b show an impedance triangle and a geometric­ally similar short-circuit triangle in which the sides represent the active and reactive components of the short-circuit primary voltage, V₁,_sc, as a percentage of the primary rated voltage, V₁,_rtd . The two components are found at I₁,_sc = I₁._rtd so

ν_sc, _a = (r_scI₁, _rtd/V₁, _rtd) X 100%

ν_sc, _r = (_scI₁, _rtd/V₁, _rtd) X 100%

and the hypotenuse of the short-circuit triangle is

ν_sc = (Z_SCI₁, _rtd/V₁, _rtd) X 100%

where ν_sc is called the impedance voltage of a transformer. It is a very important parameter on the basis of which we can determine varia­tions in the secondary voltage of a loaded transformer (see Sec. 8.8). It is usual to state the impedance voltage of a transformer on its nameplate.

The value of ν_sc increases with increasing rated voltages across the transformer windings. The reason is that at an increase in the thickness of conductor insulation, the leakage flux linkages go up, and so do the two leakage reactances , _leak,1 and _leak,2 In a transformer rated at anywhere between 5000 and 5600 kVA and with a rated voltage of 6.3 and 10 kV on the H.V. side, the impedance voltage is 5.5%. In a transformer rated at anywhere between 3200 and 4200 kVA and with a rated voltage of 35 kVon the H.V. side, the impedance volt­age is 7%.

The short-circuit test can also provide a check on the turns (or transformation) ratio. In the short-circuit condition the term I₁,_oc w₁ in Eq. (8.4) is negligible in comparison with the other two terms, so

w₁I_1,sc ≈ w₂I_2,sc (8.18)

Hence the transformation (turns) ratio is

n_2I = w₂/w₁ ≈ I_1,sc/ I_2,sc (8.19)

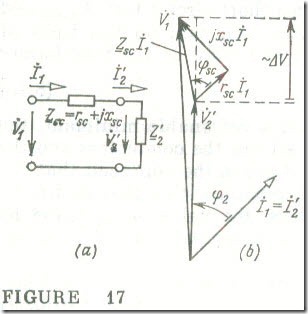
If we neglect the open-circuit (magnetizing) current I₁,_oc of a transformer and find its r_sc and _sc by a short-circuit test, we can draw up a simplified equivalent diagram (Fig. 17 a) for which a phasor diagram is drawn up in Fig. 17 b.