Pressure coefficient of expansion of a gas at constant volume
It now remains to investigate the manner in which the pressure of a gas varies when it is heated at constant volume. Let’s begin with a simple definition.
The pressure coefficient of expansion of a gas at constant volume is defined as the fraction of its pressure at 0°C by which the pressure of a fixed mass of gas increases per °C rise in temperature.
Philipp Jolly measured the pressure coefficient of expansion of air
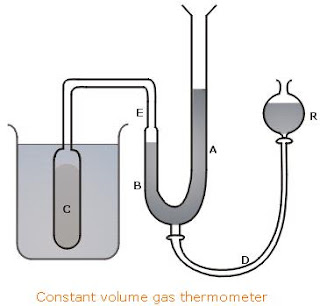 |
| Pressure law apparatus ( Simple constant volume gas thermometer |
The apparatus for this experiment was designed by the German professor of physics, Philipp Jolly, who lived during the nineteenth century. It is generally called the simple constant volume air thermometer.
A glass bulb B joined to a capillary tube is connected to a mercury reservoir R by a length of rubber tubing. By means of three-way tap air may be pumped out of the bulb and dry air afterwards admitted through a calcium chloride drying tube.
The bulb is placed in a large beaker or can and surrounded by cold water. The height of the reservoir is then adjusted until the mercury level reached a fixed mark M on the connecting tube. The temperature of the water bath is noted by a thermometer, and also the mercury levels at M and R are read on a vertical millimetre scale.
The barometric height H in mmHg is read on a Fortin barometer. If the difference in the mercury levels at M and R is h in mm, then the pressure of the air in the bulb is given by ( H± h) according as R is above or below M.
A series of such readings is obtained, as the temperature of the water bath is raised by about 10 degree intervals. Each time the mercury level is carefully adjusted to the mark M to ensure that the volume of the air remains constant.
The water is well stirred, and sufficient time must be allowed for the air to reach the same temperature as the water before the readings are taken.
The results are recorded in a table the same way we did in the previous experiment ( Charles’s law) , but this time the relation is between pressure and temperature.
Pressure coefficient calculation
Pressure coefficient = β = change in pressure/pressure at 0°C × temperature rise
or β =P100 – P0/ P0 × 100 per °C
The results is found to approximate very close to 1/273 or 0.00366/°C. All other gases besides air give very nearly the same result.
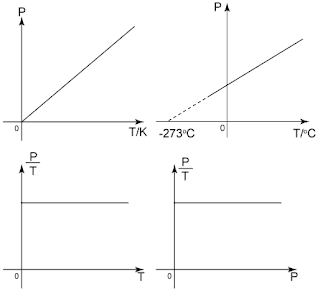 |
| Pressure law |
We therefore arrive at the important conclusion that the pressure coefficient is equal to the volume coefficient.
Pressure law
The pressure of a fixed mass of gas at constant volume increases by 1/273 of its pressure at 0°C per °C rise in temperature.
The absolute or Kelvin scale of temperature
If volume-temperature and pressure-temperature graphs for a gas are plotted on extra large sheets of paper it is found that when the graphs are produced, they cut the temperature axis at -273 °C. This suggests that -273 °C is the lowest temperature attainable or the absolute zero of temperature.
But this can only be an assumption since we know that most gases liquefy long before such a temperature is reached, and, in any case the mercury in our temperature freezes at -39°C.
Lord Kelvin showed that it is possible to define an absolute scale of temperature in terms of the product, PV, for a theoretical perfect or ideal gas, i.e., one which obeys Boyle’s law exactly under all temperature conditions.
Thus, if the product PV at the ice and steam points denoted by 0 °C are plotted on a graph and joined by a straight line, this line will cut the temperature axis at -273 °C. This is taken as the zero of what is now called the absolute or Kelvin scale of temperature.
Degrees on this scale are equal in magnitude to Celsius degrees on the perfect gas scale. They are represented by T and denoted by K.
It follows that degrees on the Celsius scale can be converted to degrees on the Kelvin scale simply by adding 273. Thus, 0 °C = 273 K,
θ °C = ( 273 + θ) K, and so on.
In the case of practical gas thermometer it is difficult to measure both P and V at the same time and so it is usual to keep V constant, in which case the temperature will be proportional to the pressure of the gas as we shall explain in the next article. So follow me please and thanks:)


