A method of verifying Boyle’s law for air

Boyle’s law animation

Boyle’s law apparatus

  We have already discussed Boyle’s law (see Galileo air thermometer and Boyle’s law), now there is a method of verifying Boyle’s law for pressures both above and below atmospheric. Nowadays we have the advantage of flexible tubing which was not available in the seventeenth century.

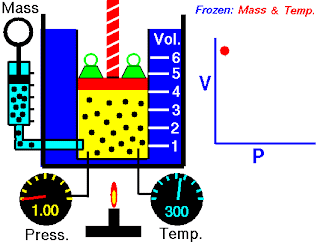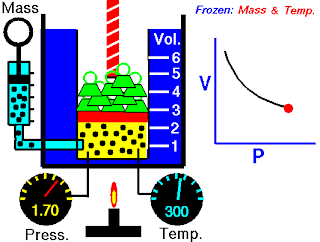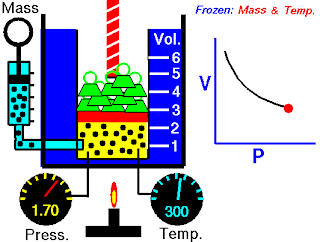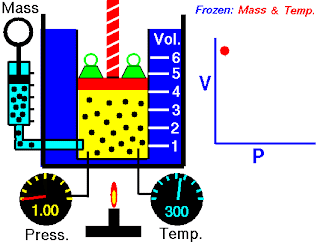
The apparatus ( not exactly as you see in the image, but close) consists of a burette ( like a test tube)  B connected by a length of rubber or plastic tubing to a glass reservoir containing mercury.

  It is first necessary to remove any water vapour which may be present with the air in the burette. The top T is opened and the reservoir raised until the burette is full of mercury. A calcium chloride CaCl₂ drying tube is attached to the top and the reservoir lowered until the burette is about half full of dry air. The tap is then closed and the drying tube removed.
  Starting with the reservoir A in its lowest position, the volume V, in cm³, of air in the burette is noted together with the readings of the mercury levels A and B on a vertical millimetre scale. The reservoir is then raised a few centimetres at a time and a series of such readings obtained.
  Now, If the volume of a gas is altered, the temperature will change. It is therefore necessary to wait a short while after each adjustment of the reservoir in order to give the air time to revert to room temperature.
  The atmospheric pressure H, in mm Hg, is read from a Fortin barometer. If the difference in the mercury level is h in mm, then the absolute pressure of the enclosed air is given by
    p= ( H + h )

when A is above B and

  p = ( H – h ) when A is below B.

Boyle’s law experiment

All the measurements made should be entered in a table as shown above.

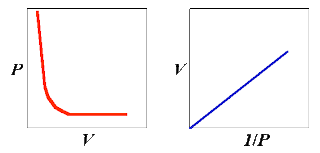
Graphical treatment of results

  In mathematics the equation y = m x gives a straight line through the origin when y is plotted against x on graph paper. In this equation m is a constant and a series of values of y are calculated by giving various values to x.

  Now, if both sides of the equation, PV = c, are divided by P it will be converted into an equation of the form y = m x.
Thus,  V= c × 1/P

Here, V is the dependent variable ( corresponding with y ) and 1/P i the independent variable ( corresponding with x ). The constant c plays the same part as m.

  Hence, if Boyle’s law is true we ought to get a straight line through the origin by plotting V against 1/P.

Coefficient of expansion of a gas at constant pressure: definition

  It may be remembered that when the coefficient of linear expansion of a solid was defined it was not specified that the original length of the rod of material should be measured at 0° C. Owing to the smallness of the linear expansion of most substances, it makes very little difference to the result obtained for the coefficient whether we start with the rod at 0° C or at ordinary temperature.
  In the case of a gas, however, the situation is different. The expansion of gases is very much larger than that of solids, and consequently the value of the coefficient of expansion obtained will depend on the starting temperature.

Hence, in order to make a satisfactory comparison between different gases, the coefficient is always calculated in terms of an original volume at 0° C. The volume coefficient of expansion of a gas is thus defined as follows:

  The coefficient of expansion of a gas at constant pressure is the fraction of its volume at 0° C by which the volume of a fixed mass of gas expands per °C rise in temperature.