Archimedes’ principle: worked examples

1. A ship of mass 1200 t floats in sea-water. What volume of sea-water does it displace?If the ship enters fresh water, what weight of cargo must be unloaded so that the same volume of water is displaced as before? ( Density of fresh water = 1000 kg/m³ , relative density of sea-water = 1.03; 1t = 1000kg)
   

Solution

  Mass of sea water displaced   = 1200 t

    Density of sea-water           =1000×1.03 kg/m³
   
    Volume displaced = mass/density = 1200×1000/1000×1.03 m³ = 1165 m³ of sea-water

The same volume of fresh water weights 1165×1000 kg = 1165 t

Therefore, weight of cargo to be unloaded = 1200 – 1165 = 35 t


2. What volume of brass of density 8.5 g/cm³ must be attached to a piece of wood of mass 100 g and density 0.2 g/cm³ so that the two together will just submerge beneath water?

Solution

  The brass and wood together will just submerge when the average density of the whole is equal to that of water ( 1 g/cm³).

  Let the volume of the brass = V ( in cm³)

then        mass of the brass =8.5 V ( in g)

           volume of the wood = 100/0.2= 500 cm³

and        mass of wood       = 100 g

   For an average density of 1 g /cm³ the total mass bust be numerically equal to the total volume.

Hence,
                100 + 8.5 V = 500 + V
rearranging     7.5 V       = 400
or                  V       = 400/7.5 = 53.3 cm³ of brass.


3. An ordinary hydrometer of mass 28 g floats with 3 cm of its stem out of water. The area of cross-section of the stem is 0.75 cm³ .

  Find the total volume of the hydrometer and the length of stem above the surface when it floats in a liquid of relative density 1.4.

Solution

    By the law of floatation, the hydrometer displaces its own weight of any liquid.

    Therefore,
              weight of water displaced = 28 g
              volume of water displaced = 28 cm³

Assuming top of stem to be flat and not rounded,

              volume of stem above water = 0.75×3 =2.25cm³
therefore


 total volume of hydrometer = 28+ 2.25 = 30.25 cm³                  
 volume of liquid displaced = mass / density = 28/1.4=20 cm³

 therefore
    length of stem above liquid = 10.25/0.75= 13.7 cm
   
 

An old story of Archimedes

 
 It is reported that on one occasion King Hiero of Syracuse asked Archimedes to ascertain if a gold crown mad by the Court goldsmith had been fraudulently alloyed with silver.
   The problem remained unsolved until Archimedes could find a way of measuring the volume of the crown.

 Tradition has it that the solution occurred to him one day at the baths. On immersing himself in a bath full to the brim he suddenly realized that the volume of water which spilled over was equal to his own volume.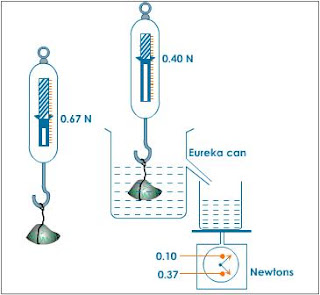  

The displacement vessel shown in the picture is sometimes called Eureka can in allusion to the legend that, in his excitement at the discovery Archimedes ran unclothed through the streets shouting, “Eureka” ( = I have found it).
   Our last worked example illustrates the kind of calculation which Archimedes may have made to incriminate the goldsmith.
 
4. A lump of gold-silver alloy has a mss of 350.8 g and a volume of 20 cm³ . Assuming that no change in volume occurs when gold is alloyed with silver, find the mass of silver in the sample. (Dinsity of gold = 19.3 g/cm³; of silver = 10.5 g/cm³ .)
 

Solution

   Let the volume of silver in the alloy = V ( in cm³ )
Then
     
     mass of silver in the alloy = 10.5 V ( in g)
and
     mass of gold in the alloy = 19.3 ( 20 – V ) ( in g)
but
    total mass of alloy        = 350.8 g
therefore
      10.5 V + 19.3 ( 20 – V ) = 350.8 g        
      10.5 V +386 – 19.3 V     =  350.8 g
      35.2                     = 8.8 V
whence                   V     = 4 cm³
it follows that
        mass of silver = 4 × 10.5
                       = 42 g.
  Finally, this a revision video for Archimedes’ principle

I hope that was fun.